(1 point) Find a unit vector that has the same direction as (4, -9, -1): 200 Find a vector that has the same direction as (4, -9, -1) but has length 8: 00 ) (1 point) A child pulls a sled through th
Answers
A vector that has the same direction as (4, -9, -1) but a length of 8 is approximately (4.528, -10.176, -1.136).
To find a unit vector that has the same direction as the vector (4, -9, -1), we need to divide the vector by its magnitude. Here's how:
Step 1: Calculate the magnitude of the vector
The magnitude of a vector (a, b, c) is given by the formula:
||v|| = √(a^2 + b^2 + c^2)
In this case, the vector is (4, -9, -1), so its magnitude is:
||v|| = √(4^2 + (-9)^2 + (-1)^2)
= √(16 + 81 + 1)
= √98
= √(2 * 49)
= 7√2
Step 2: Divide the vector by its magnitude
To find the unit vector, we divide each component of the vector by its magnitude:
u = (4/7√2, -9/7√2, -1/7√2)
Simplifying the components, we have:
u ≈ (0.566, -1.272, -0.142)
So, the unit vector that has the same direction as (4, -9, -1) is approximately (0.566, -1.272, -0.142).
To find a vector that has the same direction as (4, -9, -1) but has a different length, we can simply scale the vector. Since we want a vector with a length of 8, we multiply each component of the unit vector by 8:
v = 8 * u
Calculating the components, we have:
v ≈ (8 * 0.566, 8 * -1.272, 8 * -0.142)
≈ (4.528, -10.176, -1.136)
So, a vector that has the same direction as (4, -9, -1) but a length of 8 is approximately (4.528, -10.176, -1.136).
In this solution, we first calculate the magnitude of the given vector (4, -9, -1) using the formula for vector magnitude.
Then, we divide each component of the vector by its magnitude to obtain a unit vector that has the same direction.
To find a vector with a different length but the same direction, we simply scale the unit vector by multiplying each component by the desired length.
To learn more about vector, click here: brainly.com/question/17157624
#SPJ11
Related Questions
Find the slope and the y-intercept of the line.
y=-5/4x+5
Answers
Answer:
Slope: -5/4
y-intercept: 5
Step-by-step explanation:
y = mx + b
m = slope
b = y-intercept
the parcel is located in the sw1/4 of the sw1/4 of the nw1/4 of the se1/4 of the n1/2 of the ne1/4 of section 13. how many acres is the parcel and in which quadrant of the section will the parcel be located?
Answers
The area of the given parcel is 4.4 acres.
The given parcel is located in the SW1/4 of the SW1/4 of the NW1/4 of the SE1/4 of the N1/2 of the NE1/4 of Section 13.
Therefore, the parcel is located in the southwest quadrant of the section.
There are different methods of calculating the area of the parcel.
Here, we will be using the Chain Survey method for calculating the area of the given parcel.
Steps to calculate the area of the given parcel using the chain survey method are as follows:
Step 1 : Draw the rough sketch of the given parcel.
The rough sketch is shown below:
Step 2 : Calculate the length of the sides of the parcel using the scale.
Step 3 : Calculate the area of each triangle using the formula,
A = 1/2 bh.
The area of each triangle is shown in the diagram below:
Step 4: Add the areas of the triangles to get the total area of the parcel.
Total Area = 4.4 acres.
For similar question on area.
https://brainly.com/question/17335144
#SPJ11
Solve-3(z-6) ≥ 2z-2 for z
Answers
Answer: Z<4
Step-by-step explanation:
Rearrange the equation
-3(z-6) - (2z-2)>0
-3z+18-2z+2>0
-5z +20>0
-5(z-4)>0
divide both side by -5
z-4<0
z<4
A scale model of a buliding is 24 inches tall. The actual building is 180 feet tall. What is the scale factor of the model?
Answers
Answer:
1 inch = 7.5 feet.
Step-by-step explanation:
24 divided by 24 equals 1. 180 divided by 24 equals 7.5
- 4y = - 40
please show work if you can
Answers
-4 * 10 = 40
Hope this helps!!!:):):)
If -3r+1/2=4 then r=-7/6
Answers
Answer:
Thats the answer r=-7/6
Step-by-step explaintion
r=-7/6
The Original Price of an item is $150.00. It is on sale for 30% off. You also have a store coupon for 10% off of the sale price. What will you pay?
Answers
150 * 0.40 = $60 off
150 - 60 = $90
Solution: you pay $90
Answer:
$94.50
Step-by-step explanation:
150 x .30 = 45, so you can $45 off.
105 x .1 = 10.5 so you can take another $10.50 off.
The final price would be $94.50
find the slope of a line perpendicular to y=-2/3x+4/5
Answers
In this case, it’s just 3/2.
If your original slope was 2, then the slope of the perpendicular line would be -1/2.
how do I solve this?

Answers
Answer:
sinJ = \(\frac{12}{13}\)
Step-by-step explanation:
sinJ = \(\frac{opposite}{hypotenuse}\) = \(\frac{IK}{JK}\) = \(\frac{12}{13}\)
pls thank you pls explain it

Answers
The surface area of the shed is given as follows:
S = 367.2 m².
What is the surface area of a rectangular prism?The surface area of a rectangular prism of height h, width w and length l is given by:
S = 2(hw + lw + lh).
This means that the area of each rectangular face of the prism is calculated, and then the surface area is given by the sum of all these areas.
The shed in this problem is composed as follows:
Rectangular prism of dimensions 6m, 10 m and 3 m.Two rectangles of dimensions 6m and 10 m.Two triangles of base 6 m.The height of each triangle is obtained as follows:
h² + 3² = 6²
\(h = \sqrt{6^2 - 3^2}\)
h = 5.2 m.
Hence the surface area is given as follows:
S = 2 x (6 x 10 + 6 x 3 + 10 x 3) + 2 x 6 x 10 + 2 x 0.5 x 6 x 5.2
S = 367.2 m².
More can be learned about the surface area of a rectangular prism at brainly.com/question/1310421
#SPJ1
hi please help! i’m somehow stuck on this

Answers
Answer:p=12
Step-by-step explanation:
(9p)+(p+21)+(p+27)=180
9*12=108
12+21=33
12+27=39
108+33+39=180
so p=12
Unit 3 discussion part 2



Answers
The above problems are related to Trigonometry. See the answers below.
The answer to question 1 is 15.10 (Option C)The answer to question 2 is 18.01 (Option C)The answer to question 3 is Sine (Sin) (Option A)The answer to question 4 is (Option 4)The answer to question 5 is 54.16 (Option C)The answers to question 6 are:A right triangle (Option A)The length of at least one side (Option B)The measure of at least one angle (Option C)What is the Trigonometry?
Trigonometry is a discipline of mathematics dealing with angle relationships and length ratios. The topic arose in the Hellenistic civilization during the third century BC from geometric applications to astronomical research.
1) Using Cos θ = Adjacent /Hypothenus
Where θ = 58°;
Adjacent = 8 and Hypothenus is x
Cos 58 = 8/x; multiply each side by x
x Cos 58 = 8
0.53x = 8
x = 8/0.53
x = 15.094
x \(\approx\) 15.10 (Option C)
2) Using Sin θ = Opposite /Hypothenus; where
θ = 37 °
Hypothenus = 30
Opposite = x
⇒ Sin 37 = x/30; multiply both sides by 30
⇒ 30 Sin 37 = x
x = 30 * 0.60181502315
x \(\approx\) 18.01 (Option C)
3) The function used to solve this problem is Sine (Sin) because the Opposite and Hyphothenus are both given. (Option A)
4) To solve for the problem in this case we must use Option 4 - that is Tan x = 18/13
5) To solve for x in Tan x = 18/13
the expression goes as follows:
x = Tan⁻¹ (18/13)
x = Tan⁻¹ (1.3846153846153846153846153846154)
x = 54.16234705
x \(\approx\) 54.16 (Option C)
6) Note that from the elements listed below those required for an inverse trig problem to find a missing angle are:
Learn more about Trigonometry:
https://brainly.com/question/12473370
#SPJ1
How many solution does the system of linear equations represented in the graph below have?.
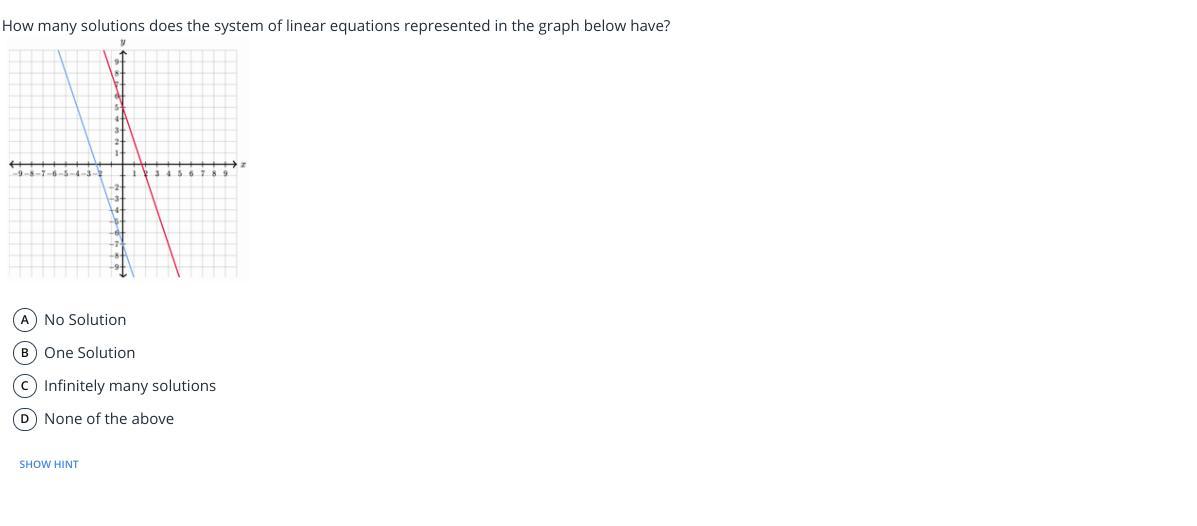
Answers
Answer:
No solution
Step-by-step explanation:
The given lines on the graph are parallel . So they will never intersect each other. Therefore they have no solution.
5. Brody bought 12 books. Two-thirds of the books are science fiction.
How many books are science-fiction? *
Vour answer
Answers
Answer:
8
Step-by-step explanation:
12 divided by 3 is 4 and since its 2/3 you multiple 4 times 2 giving you 8
Answer:
8
Step-by-step explanation:
Multiply amount of books by the fraction of

Can someone plz explain and answer ASAP??

Answers
Answer
100 times 3.14=314 times 360=113,040
Step-by-step explanation:
a little help would be nice!

Answers
Answer:
1 in : 6 ft
Step-by-step explanation:
48 / 8 = 6
24 / 4 = 6
It was scaled down by a factor of 6
Answer: 6 feet per inch
53% of MIS graduates from UIUC make above $48,861 in their first job. If we randomly select 10 graduates, what is the probability that, in their first job: a) None of them make above $48,861
Answers
The probability that none of the 10 randomly selected MIS graduates from UIUC make above $48,861 in their first job is (0.47)^10, which can be calculated using the binomial probability formula.
To find the probability that none of the 10 graduates make above $48,861, we raise the probability of an individual not making above $48,861 to the power of 10 (since we want all 10 to not make above that amount) and multiply it by the total number of combinations for selecting 10 graduates out of the total population.
Using the binomial probability formula, the probability that none of the 10 graduates make above $48,861 can be calculated as:
\(P(X = 0) = C(10, 0) * (0.47)^0 * (1 - 0.47)^{10-0}\)
where C(10, 0) represents the number of combinations of selecting 0 graduates out of 10.
Simplifying the equation, we find:
\(P(X = 0) = (1) * (1) * (0.47)^{10}\)
Therefore, the probability that none of the 10 randomly selected MIS graduates from UIUC make above $48,861 in their first job is \((0.47)^{10}\).
To learn more about Binomial probability, visit:
https://brainly.com/question/12474772
#SPJ11
What is a degree 4 function called?
Answers
Answer:
Quartic function
Step-by-step explanation:
Answer: Quartic function
Step-by-step explanation:
the average age of everyone in the class is an example of what type of statistics?
Answers
Answer: descriptive statistics
Step-by-step explanation: The average age of everyone in the class is an example of descriptive statistics.
45,987,159.9 in short form
Answers
Answer:
forty-five million, nine hundred eighty-seven thousand, one hundred fifty-nine, point nine
Step-by-step explanation:
forty-five million, nine hundred eighty-seven thousand, one hundred fifty-nine, point nine
What is the answer 250 - 150x
Answers
Answer:
50(5 - 3x)
Step-by-step explanation:
Answer:
\(50(5 - 3x)\)
Step-by-step explanation:
1) Find the Greatest Common Factor (GCF).
\(gcf = 50\)
2) Factor out the GCF. (Write the GCF first. Then, in parentheses, divide each term by the GCF.)
\(50( \frac{250}{50} + \frac{ - 150x}{50}) \)
3) Simplify each term in parentheses.
\(50(5 - 3x)\)
Therefor, the answer is 50 ( 5 - 3x ) .
What is the monthly repayment on the following hire-purchase agreement? A colour TV costs £640. The deposit was £200. The interest rate was 10% p.a. and it was paid off in two years.
Answers
The monthly repayment on the following hire-purchase agreement is £22.
What is an expression?The mathematical expression combines numerical variables and operations denoted by addition, subtraction, multiplication, and division signs.
Mathematical symbols can be used to represent numbers (constants), variables, operations, functions, brackets, punctuation, and grouping. They can also denote the logical syntax's operation order and other properties.
Given that a colour TV costs £640. The deposit was £200. The interest rate was 10% p.a. and it was paid off in two years.
The monthly repayment will be calculated as,
Total interest for two years = ( 640 - 200 ) x 1.2
Total interest for two years = ( 440 x 1.2 )
Total interest for two years = £528
The value of monthly repayment = 528 / 24 = £22
Therefore, the monthly repayment on the following hire-purchase agreement is £22.
To know more about an expression follow
https://brainly.com/question/28223788
#SPJ1
Q4. Which system of inequalities describes the graph?

Answers
Answer:
Step-by-step explanation:
2. What is the equation of a line that is passing through the point (8,11) and is perpendicular to y=2x+1.
Answers
Answer:
u need to substitute (8,11) in the equation because the line passses through that point

a^2 x b^6 if a =1/2 and b=2
Answers

The heights (in inches) of a sample of eight mother/daughter pairs of subjects were measured. Using a spreadsheet with the paired mother/daughter heights, the linear correlation coefficient is found to be 0.693.
Find the critical value, assuming a 0.05 significance level. Is there sufficient evidence to support the claim that there is a linear correlation between the heights of mothers and the heights of their daughters?
Answers
The critical value for a 0.05 significance level with df = 6 is approximately 0.632.To find the critical value for the linear correlation coefficient, we need to use a table or a statistical calculator that provides critical values for different significance levels.
Assuming a significance level of 0.05, which corresponds to a confidence level of 95%, we can find the critical value using the degrees of freedom (df), which is equal to the number of pairs minus 2 (n - 2) in this case.
For a two-tailed test, the critical value for a 0.05 significance level with df = 6 is approximately 0.632.
Now, we compare the calculated correlation coefficient (0.693) with the critical value (0.632).
If the calculated correlation coefficient is greater than the critical value in absolute value, then there is sufficient evidence to support the claim that there is a linear correlation between the heights of mothers and the heights of their daughters.
Since |0.693| > 0.632, we can conclude that there is sufficient evidence to support the claim that there is a linear correlation between the heights of mothers and the heights of their daughters at the 0.05 significance level.
To learn more about critical value go to:
https://brainly.com/question/31213260
#SPJ11
Mrs. sato tries to stabilize the gate by joining the corners at n
and q with a diagonal wooden beam of length nq. she finds
that this does not restore the right angles to the gate, although it
does divide the gate into two congruent triangles.
Answers
The diagonal beam joining N and Q forms the dividing line between the two congruent triangles within the gate.
If joining the corners at points N and Q with a diagonal wooden beam of length NQ does not restore the right angles to the gate but divides it into two congruent triangles, it suggests that the gate was not originally a rectangle or a square. A rectangle or square would have right angles at the corners, and joining the opposite corners with a diagonal would restore the right angles. However, since the gate is divided into congruent triangles, it implies that the gate has an irregular shape or a different type of quadrilateral.
To know more about diagonal,
https://brainly.com/question/14478918
#SPJ11
Find the area. Simplify your answer.
x+8
x+5
Answers
Answer:
9x+5
Step-by-step explanation:
Add x and 8x
A lump of butter has a mass of 250 g and a volume of 290 cm³. Find its density in g/cm³. Give your answer to 3 decimal places.
Answers
The density in butter has a mass of 250g and volume of 290cm³ is 0.862 g/cm³.
What is meant by density?Density is a physical property of matter that is defined as the amount of mass per unit of volume. It is measured in units of kilograms per cubic meter (kg/m^3) and is usually represented by the Greek symbol ρ (rho).
Density is a measure of how tightly the particles of a substance are packed together. The denser a substance is, the more mass it contains in a given volume.
Most commonly, density is used to compare different substances, such as solids, liquids, and gases.
In general, solids are more dense than liquids, and liquids are more dense than gases.
The density of a substance can change based on temperature, pressure, and other factors. For example, water has a greater density at 4°C than at any other temperature, and the density of a gas increases with increasing pressure.
Density is an important factor in many physical and chemical processes, including buoyancy, diffusion, and surface tension.
Density is also used in many calculations, such as calculating the volume of a container or the mass of an object.
Density, p = m/v
Where, m is mass of butter and v is volume of butter.
p = 250/290
p = 0.862 g/cm³
To learn more about density, visit
brainly.com/question/1354972
#SPJ1
rewriting subtraction into addition
-3-6=
Answers
Answer: 6+3 = 9
Step-by-step explanation:
-3-6 is equivalent to 6-(-3). Subtracting a negative number is the same as adding a positive number, so 6-(-3) is the same as 6+3. 6+3 = 9.