19.
∠P and ∠Q are supplementary. m∠P = 5x + 3 and m∠Q = x + 3. x = ?
14
0
29
30
AND
P lies in the interior of ∠RST. m∠RSP = 40° and m∠TSP = 10°. m∠RST = ?
100°
50°
30°
10°
AND
6.
What is AB?

Answers
Answer:
Step-by-step explanation:
1). Since angles P and Q are supplementary,
m∠P + m∠Q = 180°
(5x + 3)° + (x + 3)° = 180°
6x + 6 = 180
6x = 174
x = 29
Therefore, Option (3) is the answer.
2). If a point P lies in the interior of ∠RST,
m∠RST = m∠RSP + m∠TPS
(Since, line SP is bisector of angle RST)
m∠RST = 40° + 10°
= 50°
Therefore, option (2) is the answer.
3). AB = distance between two points A and B on a number line
= 8 - (-12)
= 8 + 12
= 20
Therefore, length of AB = 20 units.
Related Questions
Write the property that justifies each mathematical statement. Look carefully!!
1. a(cd) = ac - ad
2. 2x + y = y + 2x
3. — 3(4c) = (- 3 * 4)c
4. 3(4ab) = (4ab)3
5. - 9(3 + 2x) = -9(3) - 9(2x)
6. 3+ (2 + n) = (3 + 2) + n
Answers
The properties of algebra used here are
For first point the algebraic properties used is Distributive Properties of Addition Over Multiplication.
For Second point the algebraic properties used is Associative Property of Addition
For third point the algebraic properties used is Associative Property of Multiplication.
For fourth point the algebraic properties used is Commutative Property of Multiplication
For fifth point the algebraic properties used is Distributive Properties of Addition Over Multiplication.
For sixth point the algebraic properties used is Associative Property of Addition
The correct question is Write the property that justifies each mathematical statement. Look carefully!!
1. a(c-d) = ac - ad
2. 2x + y = y + 2x
3. — 3(4c) = (- 3 * 4)c
4. 3(4ab) = (4ab)3
5. - 9(3 + 2x) = -9(3) - 9(2x)
6. 3+ (2 + n) = (3 + 2) + n
To know more about properties of algebra - https://brainly.com/question/1448147
#SPJ9
1/6 of a pan and how many brownies does he need if he is going to give brownies to 5 friends
Answers
The computation shows that the number of pans needed will be 5/6.
How to compute the value?From the information given, it should be noted that it was stated that 1/6 of a pan will be needed for one person.
Therefore, the pans that will be needed for the 5 friends will be:
= 1/6 × 5..
= 5/6
Learn more about computations on:
brainly.com/question/4658834
#SPJ1
write an equation that represents the situation
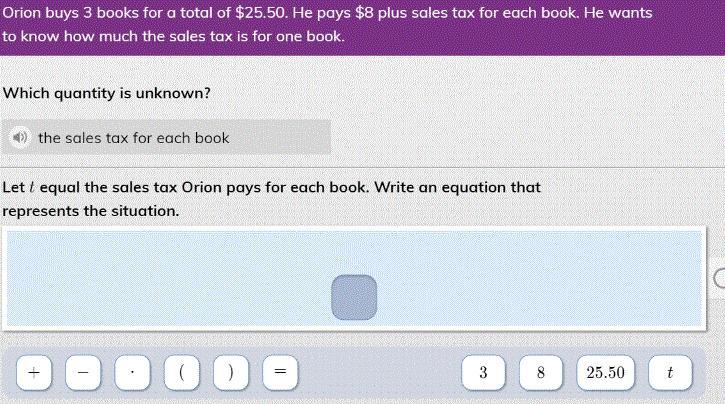
Answers
Answer:
$8 + 3t = $25.50
Two factory plants are making TV panels. Yesterday, Plant A produced 6000 fewer panels than Plant B did. Five percent of the panels from Plant A and 2% of the panels from Plant B were defective. How many panels did Plant B produce, if the two plants together produced 820 defective panels?
Answers
Answer:
16000
Step-by-step explanation:
You want to know the number of panels produced at plant B if that was 6000 more panels than at plant A, and the total number of defective panels was 820. The defect rate at plant A is 5%, and at plant B is 2%.
SetupLet b represent the number of panels produced at plant B. Then the number produced at plant A is (b-6000). The total number of defects is ...
0.05(b -6000) +0.02b = 820
SolutionSimplifying the equation, we have ...
0.07b -300 = 820
0.07b = 1120
b = 16000
Plant B produced 16000 panels.
<95141404393>
how do you Evaluate. 58−(14)2= ________
Answers
Answer:
58-(14)2
58-28
30
Step-by-step explanation:
can someone help :(!

Answers
Answer:
A
Step-by-step explanation:
You should know the area of a circle, A = πr².
So, all you need to find is the area of half a circle, or A = \(\frac{1}{2}\) πr²
We see that the radius is half of the diameter or r = 6 in.
A = \(\frac{1}{2}\) (3.14)(6)²
A = 56.52 in²
This app deleted my question so im going to ask again. please help!!! due at 5:00

Answers
Answer:
1. 87x20(4) divided by 20.
Step-by-step explanation:
Answer:
9. 0.87
10. 1.24
11. 3.4
write an equation in slope intercept form of the line that passes through the given point point and is parallel to the graph of the given equation(0,0);y=5/8x+6the equation of the line y= ?
Answers
hello
to solve this question, let's write the standard equation of a straight line
\(\begin{gathered} y=mx+c \\ \end{gathered}\)\(y=\frac{5}{8}x+6\)since, this equation passes the given gpoint and it's parallel to it, we will have to modify our equation
\(y-y_1=m(x-x_1)+c\)the given points are (0, 0)
let's insert it and solve
\(\begin{gathered} y-0=\frac{5}{8}(x-0)+6 \\ y=\frac{5}{8}x+6 \end{gathered}\)from the calculation above, the equation of the line is y = 5/8x + 6
a certain radioactive isotope has leaked into a small stream. one hundred days after the leak 8% of the original amount of substance remained. Determine the half life of this radioactive isotope
Answers
Answer:
The half-life of a radioactive substance is the time it takes for half of the initial amount of the substance to decay. We can use the fact that 8% of the original amount remains after 100 days to determine the half-life of the isotope.
Let's assume that the initial amount of the substance is 1 unit (it could be any amount, but we're assuming 1 unit for simplicity). After one half-life, half of the original amount remains, or 0.5 units. After two half-lives, half of the remaining amount remains, or 0.25 units. After three half-lives, half of the remaining amount remains, or 0.125 units. We can see that the amount of substance remaining after each half-life is half of the previous amount.
We can use this information to set up the following equation:
0.08 = (1/2)^n
where n is the number of half-lives that have elapsed. We want to solve for n.
Taking the logarithm of both sides, we get:
log(0.08) = n*log(1/2)
Solving for n, we get:
n = log(0.08) / log(1/2) = 3.42
So the number of half-lives that have elapsed is approximately 3.42. Since we know that 100 days is the time for three half-lives (from the previous calculation), we can find the half-life by dividing 100 days by 3.42:
Half-life = 100 days / 3.42 = 29.2 days (rounded to one decimal place)
Therefore, the half-life of the radioactive isotope that leaked into the stream is approximately 29.2 days.
30 %of 50 pidhdbdjduwywggdhfnfjrhdvdvdndjdjf
Answers
Answer:
15
Step-by-step explanation:
50 x .3
Answer:15
Step-by-step explanation:
Need help please!! Will give brainliest!!!

Answers
Answer:
3
-1/3
Step-by-step explanation:
up 3 right 1
down 1 right 3
Four sparking plugs and a fan belt cost $10.50 if the fen belt costs $1.75 more than sparking plugs. Find the cost of each material
Answers
Answer: x = $1.75
Step-by-step explanation:
Let's assume that the cost of each sparking plug is x dollars. Then, the cost of the fan belt is x + $1.75 since it costs $1.75 more than each sparking plug.
According to the problem, the total cost of four sparking plugs and a fan belt is $10.50. We can express this as an equation:
4x + (x + $1.75) = $10.50
Simplifying and solving for x, we get:
5x + $1.75 = $10.50
5x = $8.75
x = $1.75
Therefore, each sparking plug costs $1.75 and the fan belt costs $3.50 ($1.75 + $1.75).
Evaluate : -35 ÷ (-7) =
Answers
Answer:
-35/-7=5
Step-by-step explanation:
Diviser par de nombre négatif alors sa devient positif pareil pour la multiplication
The first four terms of an arithmetic sequence are: a, a + d, a + 2d, a + 3d. The first
four terms of another sequence are: A, Ar, Ar2, Ar3, where r 6= 1. The eight terms
satisfy:
a + A = 27 (1)
(a + d) + (Ar) = 27 (2)
(a + 2d) + (Ar2) = 39 (3)
(a + 3d) + (Ar3) = 87 (4)
By using the substitution a = 27 − A, or otherwise, find the all eight terms
Answers
The first eight terms of the sequence are 27, 27, 39, 87, 117.378, 147.755, 178.132 and 208.509.
Determination of a given set of successive values of a sequence
By (1) we have that \(a = 27 - A\), and we simplify the system of equations as follows:
\((27-A)+d + A\cdot r = 27\)
\(d + (r-1)\cdot A = 0\) (2b)
\((27-A) +2\cdot d + A\cdot r^{2} = 39\)
\(2\cdot d + (r^{2}-1)\cdot A = 12\) (3b)
\((27-A) +3\cdot d + A\cdot r^{3} = 87\)
\(3\cdot d + (r^{3}-1)\cdot A = 60\) (4b)
By (2b), we simplify the system of equations once again:
\(2\cdot (1-r)\cdot A+(r^{2}-1)\cdot A = 12\)
\([2\cdot (1-r)+(r^{2}-1)]\cdot A = 12\) (3c)
\(3\cdot (1-r)\cdot A + (r^{3}-1)\cdot A = 60\)
\([3\cdot (1-r) + (r^{3}-1)]\cdot A = 60\) (4c)
And by equalising (3c) and (4c) we have an expression in terms of \(r\):
\(\frac{12}{[2\cdot (1-r)+(r^{2}-1)]} = \frac{60}{[3\cdot (1-r)+(r^{3}-1)]}\)
\(12\cdot [3\cdot (1-r)+(r^{3}-1)] = 60\cdot [2\cdot (1-r) + (r^{2}-1)]\)
\(36\cdot (1-r) +12\cdot (r^{3}-1) = 120\cdot (1-r)+60\cdot (r^{2}-1)\)
\(84\cdot (1-r) +60\cdot (r^{2}-1)-12\cdot (r^{3}-1) = 0\)
\(84-84\cdot r +60\cdot r^{2}-60-12\cdot r^{3}-12 = 0\)
\(-12\cdot r^{3}+60\cdot r^{2}-84\cdot r +2 = 0\) (5)
The roots of this third order polynomial are: \(r_{1} \approx 0.0242\), \(r_{2} = 2.488 + i\,0.831\) and \(r_{3} \approx 2.488-i\,0.831\). Since \(r\) must be a real number, then \(r \approx 0.0242\).
By (4c) we have the value of \(A\):
\(A = \frac{60}{3\cdot (1-0.0242)+(0.0242^{3}-1)}\)
\(A \approx 31.130\)
By (2b) we find the value of \(d\):
\(d = (1-0.0242)\cdot 31.130\)
\(d = 30.377\)
And by (1) we find the value of \(a\):
\(a = 27-A\)
\(a = 27-31.130\)
\(a = -4.13\)
The first eight terms are calculated below:
\(n_{1} = 27\)
\(n_{2} = 27\)
\(n_{3} = 39\)
\(n_{4} = 87\)
\(n_{5} = [-4.13 + 4\cdot (30.377)]+(31.130)\cdot (0.0242)^{4} = 117.378\)
\(n_{6} = [-4.13+5\cdot (30.377)+(31.130)\cdot (0.0242)^{5}] = 147.755\)
\(n_{7} = [-4.13+6\cdot (30.377)\cdot (31.130)\cdot (0.0242)^{6}] = 178.132\)
\(n_{8} = [-4.13+7\cdot (30.377)\cdot (31.130)\cdot (0.0242)^{7}] = 208.509\)
The first eight terms of the sequence are 27, 27, 39, 87, 117.378, 147.755, 178.132 and 208.509. \(\blacksquare\)
RemarkThe statement present typing mistakes and is poorly formatted. Correct form is shown below:
The first four terms of an arithmetic sequence are: \(a\), \(a + d\), \(a + 2\cdot d\), \(a + 3d\). The first four terms of another sequence are: \(A\), \(A\cdot r\), \(A\cdot r^{2}\), \(A\cdot r^{3}\). The eight terms satisfy:
\(a + A = 27\) (1)
\((a+d)+A\cdot r = 27\) (2)
\((a + 2\cdot d) + A\cdot r^{2} = 39\) (3)
\((a + 3\cdot d) + A\cdot r^{3} = 87\) (4)
By using the substitution \(a = 27-A\), or otherwise, find the all eight terms.
To learn more on sequences, we kindly invite to check this verified question: https://brainly.com/question/21961097
A picture frame has a perimeter of 100 cm. It’s width is 4 cm less than twice its length. What is the width of the picture
frame?
18 cm
32 cm
48 cm
50 cm
Answers
For every 21 litres of petrol a car can run for 80 km.
How much petrol do you need (to 1 DP) to ensure the car has enough petrol of a trip that is 224 km?
Answers
For every 21 liters of petrol a car can run for 80 km- 58.2 liters of petrol would be required to ensure the car has enough petrol of a trip that is 224 km unitary method
It is provided that to run 80km car requires 21 liters of petrol
To know how much petrol is required for a trip of 224 km, we first would require to know how much petrol is required to run 1 km
This is called unitary method
Petrol required by car to run 1 km would be petrol required by car to cover 80kms of distance divided by 80 km of distance = 21 liters of petrol/ 80 km distance
= 21/80
=0.26 liters
So, petrol required by car for a trip of 224 km= 0.26 liters ×224km
= 0.26× 224
= 58.24 liters
to know more about unitary method - https://brainly.com/question/28276953
#SPJ9
what is 15^10 + 15^11 + 15^12+ 15^13
Answers
Step-by-step explanation:
\(15^{10}+15^{11}+15^{12}+15^{13}\)
You can look at it like this:
\(15^{10}+15^{11}+15^{12}+15^{13}=15^{10}+15^{10}\cdot15+15^{10}\cdot15^2+15^{10}\cdot15^3\\\\=15^{10}\cdot(1+15+15^2+15^3)=15^{10}\cdot(1+15+225+3375)\\\\=15^{10}\cdot3616=15^{10}\cdot32\cdot113=15^{10}\cdot2^5\cdot113=2.085.167.812.500.000\)
Answer:
Below.
Step-by-step explanation:
15^10 + 15^11 + 15^12+ 15^13
= 15^10(1 + 15 + 15^2 + 15^3)
= 15^10 * 3616
= 2,085,167,812,500,000
What is the domain of the square root function graphed below?

Answers
7. Kelly developed a formula for using a computer to find for a specific quote within a large set of articles.
The following function gives the length of the search, in seconds, over a set of n articles.
S(n) = 1.6 In (0.8n). What is the instantaneous rate of change of the search length for a set of 12 article:
Use correct units to explain the meaning of the answer.
Answers
instantaneous rate of change of the search length 0.1667 seconds per
How to find the instantaneous rate of change?We need to take the derivative of the function S(n) with respect to n and evaluate it at n=12 in order to determine the instantaneous rate of change of the search length for a set of 12 articles.
Taking the derivative of S(n) with respect to n using the chain rule, we get:
S'(n) = 1.6 * (1/(0.8n)) * 0.8 = 2/n
Evaluating this expression at n=12, we get:
S'(12) = 2/12 = 0.1667 seconds per article
This indicates that the search time will increase by approximately 0.1667 seconds per article if the set contains 12 articles by one unit. Alternately, the search time per article will decrease by approximately 0.1667 seconds if the set's number of articles decreases by one unit from 12.
Therefore, the units for the instantaneous rate of change are "seconds per article", indicating the change in search time for each additional or fewer article in the set.
know more about rate of change visit
https://brainly.com/question/12786410
#SPJ1
Which pair of angles are supplementary?

Answers
Answer:
1 and 7
Step-by-step explanation:
supplementary means that they add up to 180º
1,3 5,6 1,7
2,4 7,8 2,8
1,2 5,7 3,5
3,4 6,8 4,6
There are still some more but my hands are tired of typing.
Answer:
A: 1 and 7
Step-by-step explanation:
when 2 angles are put next to each other on a straight line with a line going through the middle those two angles always add to 180 since 180 is equal to a straight line.
supplementary angles are 2 angles that together make 180.
out of the options given, all of them except 1 and 7 are equal angles and as a result would not add to 180. if you put angles 1 and 7 on a straight line together, they do, thus the answer is A, aka 1 and 7.
Carlos walked to school on 14 of the 20 school days in February. Which value is equivalent to the fraction of the school days in February that Carlos walked to
school?
A 70%
B. 0.07
C. 0.142
DO 56%
Answers
Answer: A
Step-by-step explanation: 14/20 = 70%
In India, many families use mini solar cookers on their balconies. In most instances, the sun’s rays reflect off the parabolic mirror toward the dish, which is placed 32 cm from the center of the cooker. The distance from the vertex to the edge of the cooker is 68 cm.
A parabolic reflector in orange that is 32 cm away from the center of the cooker. The center of the cooker is labeled as (0,0) and the vertex of the parabola is labeled as (-32,0). The distance from the vertex to the edge of the cooker is 68 cm.
1. What are the coordinates of the edges of the cooker? What is the distance all the way across the cooker from the two points labeled "edge of the cooker?"
2. Write an equation for the parabolic mirror using the coordinates. Explain how you wrote the equation.

Answers
1. The distance all the way across the cooker. In this case, 136 cm.
1. The coordinates of the edges of the cooker can be determined by considering the distance from the vertex to the edge of the cooker. Given that the distance from the vertex to the edge is 68 cm, and the vertex is at (-32, 0), we can find the coordinates of the edges as follows:
One edge of the cooker: (-32 + 68, 0) = (36, 0)
The other edge of the cooker: (-32 - 68, 0) = (-100, 0)
The distance all the way across the cooker. In this case, it is 36 - (-100) = 136 cm.
2. To write an equation for the parabolic mirror, we can use the standard form of a parabolic equation, which is y = a(x - h)² + k, where (h, k) represents the vertex of the parabola.
In this case, the vertex is at (-32, 0).
The equation becomes y = a(x + 32)² + 0.
Substituting these coordinates into the equation, we get:
0 = a(36 + 32)² + 0
0 = a(68)²
0 = 4624a
From this, we can see that a = 0, which means the equation simplifies to y = 0, indicating that the parabolic mirror is a straight line along the x-axis.
Learn more about Parabola here:
https://brainly.com/question/29267743
#SPJ1
3. Letx = -5 and y=-*
0
Which expression has a negative value?
A. x + y
B. x - y
C. X. y
D.
Y
Answers
Answer:
A
Step-by-step explanation:
I don't really understand the numbers but if both X and Y are negative, then adding the 2 will result in a negative value. Multiplying 2 negative values will be positive, as will dividing them, and sometimes subtracting will too.
six slips of paper, each containing a different one of the numbers 3,4,5,6,7,8 and 10 are placed in a hat. then two of the slips are drawn at random
Answers
The probability of drawing two slips of paper containing numbers that are the lengths of two sides of a triangle in the (3,4,5) family is 1/50.
The (3,4,5) family of triangles is a set of triangles that are similar to the triangle with side lengths 3, 4, and 5. In particular, the sides of any triangle in the (3,4,5) family must satisfy the Pythagorean theorem, i.e., the sum of the squares of the two shorter sides must equal the square of the longest side.
We can start by listing all the possible pairs of numbers that can be drawn from the hat
(3,4), (3,5), (3,6), (3,7), (3,8), (3,10)
(4,5), (4,6), (4,7), (4,8), (4,10)
(5,6), (5,7), (5,8), (5,10)
(6,7), (6,8), (6,10)
(7,8), (7,10)
(8,10)
Out of these 20 possible pairs, we need to count the pairs that satisfy the conditions for being lengths of two sides of a triangle in the (3,4,5) family. For any such triangle, the longest side must be either 5 or 10. Therefore, we can split the pairs into two groups
Pairs that include 5: (3,4), (5,6), (5,8), (5,10)
Pairs that include 10: (6,8), (7,10), (8,10)
For a pair to be the lengths of two sides of a triangle in the (3,4,5) family, the sum of the squares of the two shorter sides must equal the square of the longest side. We can check this condition for each of the pairs in the two groups
Pairs that include 5
(3,4): not a valid triangle
(5,6): not a valid triangle
(5,8): the sum of the squares of the two shorter sides is 5^2 + 8^2 = 89, which is not equal to 10^2
(5,10): the sum of the squares of the two shorter sides is 3^2 + 4^2 = 25, which is equal to 5^2
Pairs that include 10
(6,8): the sum of the squares of the two shorter sides is 6^2 + 8^2 = 100, which is equal to 10^2
(7,10): not a valid triangle
(8,10): the sum of the squares of the two shorter sides is 4^2 + 8^2 = 80, which is not equal to 10^2
Therefore, out of the 20 possible pairs, only 2 pairs satisfy the conditions for being lengths of two sides of a triangle in the (3,4,5) family. The probability of drawing one of these pairs is 2/20 = 1/10.
Once the first number is drawn, there are only 5 slips of paper left in the hat, and only one of them is the other side of the triangle, so the probability of drawing the second number is 1/5. Therefore, the probability of drawing two numbers that are the lengths of two sides of a triangle in the (3,4,5) family is (1/10) × (1/5) = 1/50.
Learn more about probability here
brainly.com/question/11234923
#SPJ4
Express the following quentitis as ratio 10k to 50k
Answers
Answer:
watch me whipzdudbbd iv ex
Use the given information to find the compound interest earned by the deposit: ... of $12000 invested at 9% compounded quarterly for 1.25 years.
Answers
The compound interest earned is $1412.13.
Compound interest is the interest on savings calculated on both the initial principal and the accumulated interest from previous periods.
Compound interest is accelerated, and not linear.
"Interest on interest," or the power of compound interest, is believed to have originated in 17th-century Italy. It will make a sum grow faster than simple interest, which is calculated only on the principal amount.
Compounding multiplies money at an accelerated rate and the greater the number of compounding periods, the greater the compound interest will be.
Here, for this question, we will have to use the compound interest formula.
A = P(1 + r/n)^nt (Amount earned after compounding)
A = 12000(1 + .09/4)^[4(1.25)]
A = 12000(1.0225)^5
A ≈ 12000(1.117678)
A ≈ $13,412.13
i = A - P (interest earned)
i = 13,412.13 - 12000
i ≈ $1412.13
To learn more about compound interest, visit
brainly.com/question/14295570
#SPJ4
SOMEONE HELP PLEASE EXPLAIN THIS

Answers
1. The exponential growth function for Jennifer's investment account balance, I(t), can be expressed as: I(t) = a * (1 + b)ⁿ
2. The value of $50,000 after 10 years with an annual interest rate of 15% would be approximately $202,300.
3. Jennifer would have approximately $271,790 in her investment account after 8 years with an initial investment of $100,000.
How to calculate the value1. The exponential growth function for Jennifer's investment account balance, I(t), can be expressed as:
I(t) = a * (1 + b)ⁿ
Therefore, the function for Jennifer's investment account balance after t years is:
I(t) = $50,000 * (1 + 0.15)ⁿ
2. In order to calculate how much money Jennifer will have after 10 years, we can substitute t = 10 into the function:
I(10) = $50,000 * (1 + 0.15)¹⁰
= $50,000 * (1.15)¹⁰
A ≈ $202,300
3. In order to calculate how much money Jennifer would have after 8 years using this new function, we substitute t = 8 into the equation:
N(8) = $100,000 * (1 + 0.15)⁴ × (1 + 0.15)⁴
≈ $271,790
Learn more about interest on
https://brainly.com/question/25793394
#SPJ1
sat question I can’t seem to understand fully.

Answers
The value of m + n is given as follows:
A. 18.
How to obtain the value of m + n?The exponential expression for this problem is defined as follows:
\((x^5y^6)^{\frac{1}{5}}(x^3y^4}^{\frac{1}{4}}\)
Applying the power of power rule, we have that the exponents are given as follows:
x: 5/5 + 3/4 = 1 + 3/4 = 7/4.y: 6/5 + 4/4 = 6/5 + 1 = 11/5.Then the values of m and n are given as follows:
m = 7, n = 11.
Thus the sum is given as follows:
m + n = 7 + 11 = 18.
More can be learned about exponent rules at https://brainly.com/question/11975096
#SPJ1
Where do the medians of the triangle intersect?

Answers
Answer:
The medians intersect at 4-4 (i think).
Step-by-step explanation:
Every triangle has three medians and they all intersect in the triangles centroid. i believe that the medians intersect at 4-4.
i am not a very trustworthy source so you should probably ignore this awnser.
The domain of the function f(x)=-x3+4
Answers
Answer:
Domain= {x:x £|R}
|R=any real number