34°
ength of b
3
C
10
First, complete the equation.
c²= a² + b² - 2abcosC
c²=32 10²-2(3)([?]) cos
Enter the side length that belongs in the green box.
Enter

Step-by-step explanation:

the car is traveling east and therefore relative to the intersection in the west directly with 50 ft/s.

the speed away from the tower is the additional distance to the tower after the 3 seconds.

the distance from the tower we get via Pythagoras

c² = a² + b²

c being the Hypotenuse (= the distance car to the tower). a and b are the legs (the distance car-intersection, and the distance tower-intersection).

the distance from the intersection after 3 seconds is 3×50 ft = 150 ft.

let's call the distance from the car to the tower "distance".

distance² = 150² + 80² = 22,500 + 6,400 = 28,900

distance = sqrt(28,900) = 170 ft

the initial distance car to tower at the intersection was 80 ft.

after 3 seconds it is 170 ft. so, the distance increased by 170 - 80 = 90 ft.

so, the relative speed of the car at that moment moving away from the tower is

90 ft / 3s = 30 ft/s

Answer:

266640 population

Step-by-step explanation:

Answer:

$3606.44

Step-by-step explanation:

The question asks us to calculate the principal amount that needs to be invested in order to earn an interest of $6180 in 28 years at an annual interest rate of 6.12%.

To do this, we need to use the formula for simple interest:

\(\boxed{I = \frac{P \times R \times T}{100}}\),

where:

I = interest earned

P = principal invested

R = annual interest rate

T = time

By substituting the known values into the formula above and then solving for P, we can calculate the amount that James needs to invest:

\(6180 = \frac{P \times 6.12 \times 28}{100}\)

⇒ \(6180 \times 100 = P \times 171.36\)     [Multiplying both sides by 100]

⇒ \(P = \frac{6180 \times 100}{171.36}\)    [Dividing both sides of the equation by 171.36]

⇒ \(P = \bf 3606.44\)

Therefore, James needs to invest $3606.44.

So, the two balls are located on the same plane, the x-y plane, with the baseball at <3,5,0>m and the tennis ball at <-3,-1,0>m, with the vector pointing from baseball to the tennis ball is <-6,-6,0>m.

The position vector of the baseball is <3,5,0>m. The position vector of the tennis ball is <-3,-1,0>m.

The position vector of the tennis ball relative to the baseball is the vector pointing from the baseball to the tennis ball and it is calculated by subtracting the position vector of the baseball from the position vector of the tennis ball: <-3,-1,0>m - <3,5,0>m = <-6,-6,0>m

So the two balls are located on the same plane, the x-y plane, with the baseball at <3,5,0>m and the tennis ball at <-3,-1,0>m, with the vector pointing from baseball to the tennis ball is <-6,-6,0>m.

To learn more about Position Vector

Visit; brainly.com/question/14552074

#SPJ4

Hello and Good Morning/Afternoon:

The table shows a linear function:

 \(\hookrightarrow \text{This means that the slope is consistent between all the points}\\\)

Thus we can use any point to help us find our slope:

 \(\hookrightarrow \text{let's pick two points}\)

       \(\hookrightarrow \text{Slope} = \dfrac{y_2-y_1}{x_2-x_1} = \dfrac{16-7}{5-2} =\dfrac{9}{3} =3\)

Answer: 3

Hope that helps!

#LearnwithBrainly

Answer:

m = 6

Step-by-step explanation:

First, add like terms:

2m + 7m + 3m = 72

12m = 72

Divide each side by 12:

m = 6

So, the answer is m = 6

Answer:

m = 6

Step-by-step explanation:

2 m + 7 m + 3 m = 72

12 m = 72

\( \sf \small\: m \: = \frac{72}{12} \\ \)

m = 6

Hence, your answer is 6.

Answer:

Step-by-step explanation:

Given that,

x*y=\(\dfrac{(2x-y)}{(x+y}\)

3*2=

\(\dfrac{2(3)-2}{3+2}\\\\\dfrac{6-2}{5}\\\\\dfrac{4}{5}\)

Therefore:-

\(\therefore {3*2}=\dfrac{4}{5}\)

Answer:

Here's your answer I hope I'm right and i apologize if I am wrong.

It might be the second attachment if it is not I deeply apologize.

Here is the answer

\(\\ \sf\longmapsto 8888+888+88+88+8+8+8+8+8+8\)

Similar Question .

Write 1000 using 8

Answer:-

888+88+8+8+8

Answer:

One of the options comes from this:

It means 10000 contains 1250 of 8's:

The 8's to make 10000 are:

Answer:

7250%

Step-by-step explanation:

To express 72 1/2 as a percentage, you need to convert it to a decimal first and then multiply by 100.

72 1/2 is equivalent to 72.5 as a decimal.

To convert it to a percentage:

72.5 * 100 = 7250

Therefore, 72 1/2 is equal to 7250% when expressed as a percentage.

let me know it is helpful for you or not

This system has no solutions, which means that the expression 4a + 5b + 6c has no minimum value.

An expression is a mathematical phrase that can contain variables, constants, operators, and functions.

It can be a combination of numbers,variables, and operators that represents a mathematical relationship or formula. Expressions can be simple or complex, and they can be used to represent a wide range of mathematical concepts, including equations, inequalities, functions, and identities.

Examples of expressions include:

3x + 5y

(2a + 3b) / (4c - 1)

√(x² + y²)

sin(x) + cos(y)

5 - 2z

Expressions can be evaluated or simplified by substituting values for the variables or using mathematical rules to simplify the expression.

As the expression 4a + 5b + 6c does not have any constraints or limitations, there are infinitely many values of a, b, and c that can satisfy the expression.

However, if you want to find the minimum value of the expression, you can use techniques such as calculus. Taking partial derivatives of the expression with respect to each variable and setting them equal to zero gives the system of equations:

4 = 0

5 = 0

6 = 0

To know more about minimum value visit:

https://brainly.com/question/15069186

#SPJ1

Answer:

77,350

Step-by-step explanation:

77,345

you look the next number after 4

It is 5 so if its more than 5 or 5 itself you need to plus 1 ( + 1 ) - 4 plus one - and the 5 you need to do it as 0

So 77,34+ 0001 = 77,350

I think so

Dx describes the median of the given triangle, Option A is correct.

A triangle is a three-sided polygon that consists of three edges and three vertices.

A median of a triangle is a line segment that joins a vertex to the mid-point of the side that is opposite to that vertex

The line segment that is drawn from a vertex to the opposite side bisecting the side at a right angle is called perpendicular bisector.

An angle bisector or the bisector of an angle is a ray that divides an angle into two equal parts

The altitude of a triangle is the perpendicular line segment drawn from the vertex to the opposite side of the triangle.

By considering all the definitions Dx will be median, Dx divides the opposite side equally.

Hence, Dx describes the median of the given triangle, Option A is correct.

To learn more on Triangles click:

https://brainly.com/question/2773823

#SPJ7

Based on the supply graph and demand graph shown above, the price at the point of equilibrium is $ 30.

Demand refers to quantity of a commodity that the consumers are willing to, able to purchase at a given price during a given period of time. Supply refers to quantity of a commodity that the producers are willing to, able to offer for sale at a given price during a given period of time.

Demand curve slopes downward due to inverse relationship between price and quantity demanded whereas supply curve slopes upward due to direct relationship between quantity supplied and price. When both demand and supply curve intersect with each other balance is achieved. Intersection point between demand and supply curve is known as equilibrium.

At this point when prices are equal is known as equilibrium price and when quantiy demanded or supplied are equal it is known as equilibrium quantity. When we combine the given graph. Equilibrium is achieved at a point when price is equal to $ 30 and quantity is equal to 20 units.

To learn more about equilibrium of demand and supply:

https://brainly.com/question/30237240

The values of the variables are;

x = 7

y = -9

From the information given, we have that;

5x−y=44

−3x−y=−12

Using the elimination method of solving simultaneous equations

Subtract equation (2) from equation (1), we get;

5x - y - (-3x - y) = 44 - (-12)

Now, expand the bracket

5x - y+ 3x + y = 56

collect the like terms

5x + 3x = 56

add the terms

8x = 56

Make 'x' the subject of formula

x= 7

Now, substitute the value of x in equation (2)

-3x - y = -12

-3(7) - y = -12

expand the bracket

-21 - y= - 12

collect like terms

-y = 9

y = -9

Learn about simultaneous equation at: https://brainly.com/question/16863577

#SPJ1

Answer:

  (9ln(3) -6)/4 ≈ 0.97187765

Step-by-step explanation:

A horizontal slice of the area will range from g^-1(y) to f^-1(y). The inverse functions are ...

  f^-1(x) = 1/2·ln(x)

  g^-1(x) = -1/4·ln(x)

Then the integral can be written as ...

  \(\displaystyle A=\int_1^3{\left(\dfrac{1}{2}\ln(y)-\dfrac{-1}{4}\ln(y)\right)}\,dy=\dfrac{3}{4}\int_1^3{\ln(y)}\,dy=\dfrac{3}{4}\left.(x\ln{(x)}-x)\right|_1^3\\\\=\dfrac{3}{4}(3\ln(3)-(3-1))=\boxed{\dfrac{9\ln(3)-6}{4}\approx0.97187765}\)

__

The attachment shows a numerical integration using a vertical slice of area. The result is identical to 12 significant figures.

The sample space for rolling a fair 12-sided die is S = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12}.

Sample space is a set of all possible outcomes of an experiment or random event. It is the collection of all possible results or outcomes that can occur when a particular event is performed.

In the given question,

The sample space is the set of all possible outcomes of an experiment. In this case, the experiment is rolling a fair 12-sided die. The sample space would include all possible values that the die can land on when rolled.

Since a 12-sided die has 12 equally likely outcomes, the sample space would consist of the numbers 1 through 12. However, only one of the options presented in the question includes all 12 numbers in the sample space, which is S = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12}.

Option S = {1, 2, 3, 4, 5, 6} represents the sample space of a regular 6-sided die, while options S = {1} and S = {12} only include one possible outcome, which is not applicable for a 12-sided die.

To know more about sets and sample space, visit:

https://brainly.com/question/30464166

#SPJ1

The equation of the line that is perpendicular to the line 5x + 6y= 18 and passes through the point (10, 7) is

According to the question,

We have the following information:

The equation of the line: 5x+6y = 18

Point through which line is passing = (10,7)

Now, we will convert the given equation of the line into slope-intercept form:

y = mx+b where m is the slope

6y = -5x+18

y = -5x/6+3

Now, the slope of this line is -5/6.

So, the slope of the perpendicular line:

-1/m

6/5

Now, following formula is used to find the equation:

(y-y') = m(x-x')

We have x' = 10 and y' = 7.

(y-7) = 6/5(x-10)

y-7 = 6x/5-12

Moving 7 from the left hand side to the right hand side:

y = 6x/5-12+7

y = 6x/5-5

Hence, the equation of the line that is perpendicular to the line 5x + 6y= 18 and passes through the point (10, 7) is y = 6x/5-5.

To know more about equation of the line here

https://brainly.com/question/21511618

#SPJ1

Answer:

ito yung link o /fhytuewfxnopjbfw/ yan na po

Step-by-step explanation:

Mass (m) = 11 g

volume (v) = 13 ml

density (d) = ?

We know density is defined as mass per unit volume so

d = m / v

= 11 / 13

= 0.85 g/ml

Density (d) = 0.85 g/ml

Answer:

B

Step-by-step explanation:

Answer:

30, 5 , 21

Step-by-step explanation:

substitute the values of x into f(x) , then

f(- 5) = (- 5)² + 5 = 25 + 5 = 30

f(0) = 0² + 5 = 0 + 5 = 5

f(4) = 4² + 5 = 16 + 5 = 21

Answer:

Step-by-step explanation:

range = highest value - lowest value

Jesse plays basketball for his high school team. In his last 12 twelve games he scored the following points: 18, 22, 19, 15, 24, 22, 18, 15, 10, 14, 18, 10

highest is 24 and lowest is 10

range os Jesse = 24-10= 14

Barbara plays basketball for her high school team In her last 10 games she scored the following points: 15, 12, 12, 16, 18, 22, 16, 20, 20, 14

highest is 22 and lowest is 12

range is 22-12=10

Jesse has largest range of 14 points

difference in range points is 14-10=4

Answer:

)0

Step-by-step explanation:

Answer: a = 9, b = 48, c = -1

Step-by-step explanation:

"a" represents the points you receive if an Ace is picked.  It is given that you get 9 points ----> a = 9

"b" represents the number of cards that are Not an Ace.  4 cards in the deck are Aces so 52 - 4 = 48 cards are Not an Ace -----> b = 48

"c" represents the points you receive if Not an Ace is picked.  It is given that you lose 1 point ----> c = -1

Answer:

Here is the rest of the page

Step-by-step explanation:

DEFINITIONS:

Rational Exponents are also called Fractional Exponents. Consider the expression below:

The fractional exponent m/n indicates a radical with index n and exponent m, such that

SOLUTION TO THE QUESTION:

The question is given to be:

Applying the rule of exponents we have stated earlier, we can write out the expression to be:

where, in our case

Therefore, the answer is:

Answer:

Step-by-step explanation:

Question 8

Let the equation of a line passing through two points \((x_1,y_1)\) and \((x_2,y_2)\) is given by,

y = mx + b

Here, m = slope and y-intercept = b

Since, m = \(\frac{y_2-y_1}{x_2-x_1}\)

For two points (1, 4) and (5, 8),

m = \(\frac{8-4}{5-1}\)

m = 1

Equation will be,

y = (1)x + b

y = x + b

Since, this line passes through (1, 4),

4 = 1 + b

b = 3

Therefore, equation of the line will be,

y = x + 3

Question 9

Let the equation is y = mx + b

m = \(\frac{y_2-y_1}{x_2-x_1}\)

For two points (2, 10) and (6, 4)

m = \(\frac{10-4}{2-6}\)

m = \(-\frac{3}{2}\)

By substituting the value of 'm' in the equation,

y = \(-\frac{3}{2}x+b\)

Since, this line passes through (2, 10)

10 = \(-\frac{3}{2}(2)+b\)

b = 10 + 2

b = 12

Therefore, equation of the line will be,

\(y=-\frac{3}{2}x+12\)

Step-by-step explanation:

-coefficent=1

-collect like terms /variables

-add numbbers (1+2)=3p

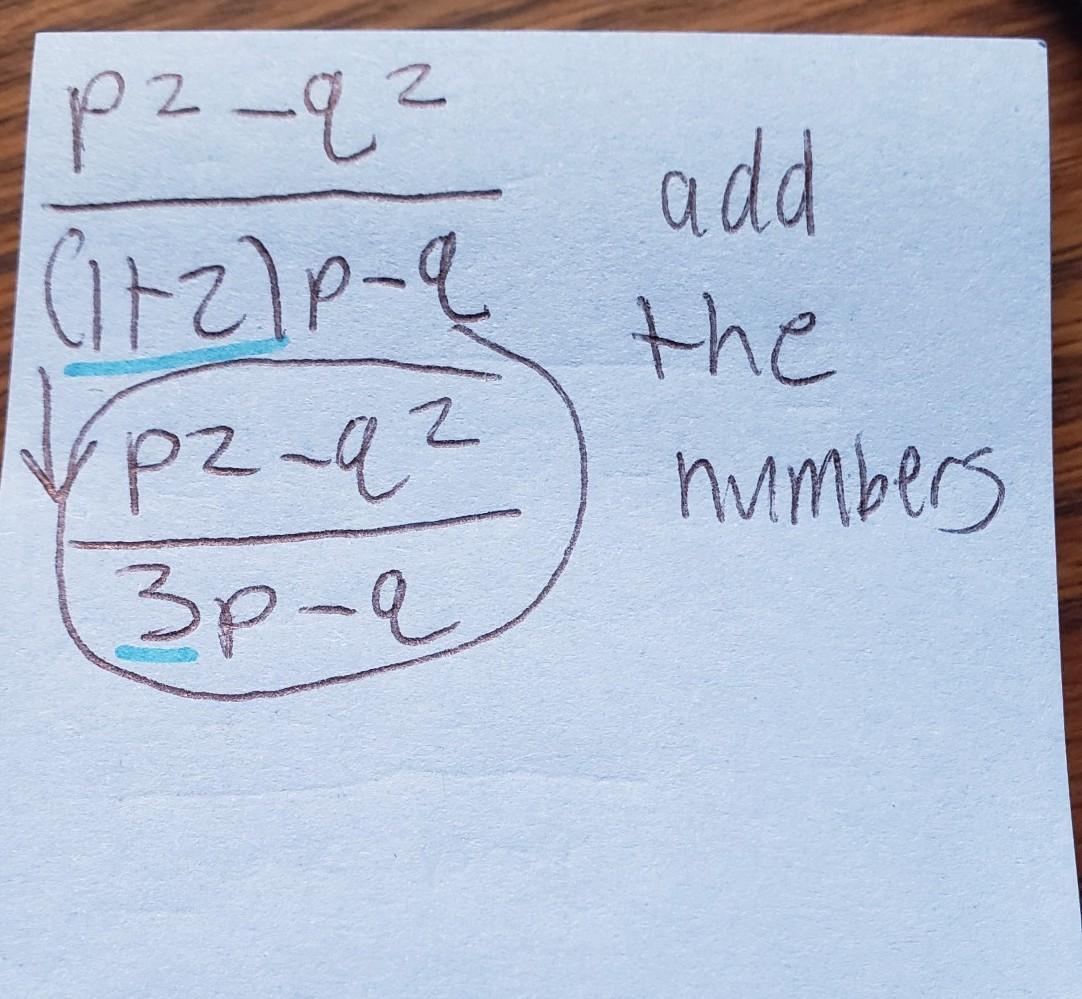
Answer:

1

Step-by-step explanation:

(p+q)(p-q)/(p+q)(p-q)=1

[It is an identity : a²-b²= (a+b)(a-b)]

Hope this will help:)