A firm will establish a branch office in Toronto with probability 0.7 and a branch office in Mexico City with probability 0.4. Suppose the probability they will open an office in at least one of the two cities is 0.8. Find the probability that
(a) the firm establishes a branch office in both cities.
(b) the firm establishes a branch office in neither of the cities.
Answers
a) The probability of establishing branch offices in both cities is 0.4. (b) The probability of not establishing branch offices in either city is 0.2.
(a) Let A represent the event of establishing a branch office in Toronto, and B represents the event of establishing a branch office in Mexico City. The probability of opening an office in both cities is P(A ∩ B). Since the events are independent, P(A ∩ B) = P(A) * P(B) = 0.7 * 0.4 = 0.28.
(b) The probability of not establishing a branch office in either city is equivalent to the complement of opening an office in at least one of the cities. Let C represent the event of not opening an office in Toronto, and D represent the event of not opening an office in Mexico City. We want to find P(C ∩ D). Since the events are mutually exclusive, P(C ∩ D) = P(C) * P(D) = (1 - P(A)) * (1 - P(B)) = 0.3 * 0.6 = 0.18.
Therefore, the probability of establishing a branch office in both cities is 0.28, and the probability of not establishing a branch office in either city is 0.18.
To learn more about “probability” refer to the https://brainly.com/question/13604758
#SPJ11
Related Questions
Please someone help

Answers
Answer:
cos is used if there is adjacent and hypotenuse
let f(x,y) = exy sin(y) for all (x,y) in r2. verify that the conclusion of clairaut’s theorem holds for f at the point (0,π/2).
Answers
To verify that the conclusion of Clairaut's theorem holds for f at the point (0,π/2), we need to check that the partial derivatives of f with respect to x and y are continuous at (0,π/2) and that they are equal at this point. Since e^(π/2) is not equal to π/2, the conclusion of Clairaut's theorem does not hold for f at the point (0,π/2).
First, let's find the partial derivative of f with respect to x:
∂f/∂x = yexy sin(y)
Now, let's find the partial derivative of f with respect to y:
∂f/∂y = exy cos(y) + exy sin(y)
At the point (0,π/2), we have:
∂f/∂x = π/2
∂f/∂y = e^(π/2)
Both partial derivatives exist and are continuous at (0,π/2).
To check that they are equal at this point, we can simply plug in the values:
∂f/∂y evaluated at (0,π/2) = e^(π/2)
∂f/∂x evaluated at (0,π/2) = π/2
Since e^(π/2) is not equal to π/2, the conclusion of Clairaut's theorem does not hold for f at the point (0,π/2).
To know more about Clairaut's theorem visit:
https://brainly.com/question/13513921
#SPJ11
please Just give me the right answers thank you
Identify the choice that best completes the statement or answers the question. [6 - K/U] 1. If x³ - 4x² + 5x-6 is divided by x-1, then the restriction on x is a. x -4 c. x* 1 b. x-1 d. no restrictio
Answers
The restriction on x when x³ - 4x² + 5x - 6 is divided by x - 1 is x = 1.
How to find the value of x that satisfies the restriction when x³ - 4x² + 5x - 6 is divided by x - 1?When we divide x³ - 4x² + 5x - 6 by x - 1, we perform polynomial long division or synthetic division to find the quotient and remainder.
In this case, the remainder is zero, indicating that (x - 1) is a factor of the polynomial.
To find the restriction on x, we set the divisor, x - 1, equal to zero and solve for x.
Therefore, x - 1 = 0, which gives us x = 1.
Hence, the value of x that satisfies the restriction when x³ - 4x² + 5x - 6 is divided by x - 1 is x = 1.
Learn more about polynomial long division
brainly.com/question/32236265
#SPJ11
A land surveyor wants to measure the distance across a portion of a lake. The surveyor forms the right triangle shown by forming two legs of a right triangle measured on land.
Answers
The surveyor first measures the length of the base of the triangle, which is the side of the lake that is closest to the shore.
What is triangle?Triangle is a three-sided geometric shape which has three angles and three sides. The three sides of a triangle are typically referred to as the base, the height, and the hypotenuse. A triangle can be classified as either right, obtuse, or acute depending on the measure of its angles. Right triangles have one right angle, obtuse triangles have one obtuse angle, and acute triangles have three acute angles.
This is done by stretching a measuring tape between two points on land. The surveyor then measures the height of the triangle by stretching a measuring tape up from the base to the point on land opposite of the base.
By using the Pythagorean Theorem, the surveyor is able to determine the length of the hypotenuse of the triangle, which is the distance across the lake. This hypotenuse is the measurement the surveyor wanted to make. The Pythagorean Theorem states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
Using this method, the surveyor is able to accurately measure the distance across the lake without ever having to set foot in the water. This method is used for many types of land surveys, and is a great way to measure distances that are difficult to measure directly.
To know more about triangle click-
brainly.com/question/17335144
#SPJ1
Does (3, 0) make the equation y = x true?
Answers
Hey there! I'm happy to help!
This does not satisfy the equation y=x as x and y have two different values. 0 and 3 are not equal. A point like (3,3) would satisfy that equation.
Have a wonderful day! :D
y=x
0=3
Now, is 3=0? No. So the equation is false
Hope this helps :)
Consider the nonlinear ordinary differential equation dx/dt =x^{2}-x-6. Find all equilibrium points and determine their stability.
Answers
The equilibrium points of the given nonlinear ordinary differential equation dx/dt = x^2 - x - 6 are x = -2 and x = 3.
To find the equilibrium points of the given nonlinear ordinary differential equation, we set dx/dt equal to zero and solve for x. In this case, we have:
x^2 - x - 6 = 0
Factoring the quadratic equation, we get:
(x - 3)(x + 2) = 0
Setting each factor equal to zero, we find two equilibrium points:
x - 3 = 0 --> x = 3
x + 2 = 0 --> x = -2
So, the equilibrium points are x = -2 and x = 3.
To determine the stability of these equilibrium points, we can analyze the behavior of the system near each point. Stability is determined by the behavior of solutions to the differential equation when perturbed from the equilibrium points.
For the equilibrium point x = -2, we can substitute this value into the original equation:
dx/dt = (-2)^2 - (-2) - 6 = 4 + 2 - 6 = 0
The derivative is zero, indicating that the system is at rest at x = -2. To analyze stability, we can consider the behavior of nearby solutions. If the solutions tend to move away from x = -2, the equilibrium point is unstable. Conversely, if the solutions tend to move towards x = -2, the equilibrium point is stable.
For the equilibrium point x = 3, we substitute this value into the original equation:
dx/dt = 3^2 - 3 - 6 = 9 - 3 - 6 = 0
Similar to the previous case, the system is at rest at x = 3. To determine stability, we analyze the behavior of nearby solutions. If the solutions move away from x = 3, the equilibrium point is unstable. If the solutions move towards x = 3, the equilibrium point is stable.
In conclusion, the equilibrium points of the given nonlinear ordinary differential equation are x = -2 and x = 3. The stability of x = -2 and x = 3 can be determined by analyzing the behavior of nearby solutions.
Learn more about equilibrium points:
brainly.com/question/32765683
#SPJ11
If an object looks the same on both sides when divided by a plane, it has
O rotational symmetry.
no plane of symmetry.
O reflectional symmetry.
Ono axis of symmetry.
K
Answers
Answer:
reflectional symmetry
Step-by-step explanation:
Work out 97.974436÷14and give your answer to 2 decimal places.
Answers
Answer:
7
Step-by-step explanation:
97.974436/ 14= 6.998174
round to 2 decimal places by looking at the third digit after decimal and is 8 that is greater than 5 so will round up the second digit after the decimal
But, that will make 9 a 10 so we have to make the number 7
From a survey of coworkers you find that 42% of 150 have already received this year's flu vaccine. An approximate 95% confidence interval is (0.339.0.501). Which of the following are true? If not, explain briefly. ses a) 95% of the coworkers fall in the interval (0.339,0.501). b) We are 95% confident that the proportion of coworkers who have received this year's flu vaccine is between 33.9% and 50.1%. om c) There is a 95% chance that a randomly selected coworker has received the vaccine. d) There is a 42% chance that a randomly selected coworker has received the vaccine. e) We are 95% confident that between 33.9% and 50.1% of the samples will have a proportion near 42%.
Answers
The approximate 95% confidence interval for the proportion of coworkers who have received this year's flu vaccine is (0.339, 0.501). Based on this information, it is true that 95% of the coworkers fall within the interval (0.339, 0.501), and we can be 95% confident that the proportion of coworkers who have received the vaccine is between 33.9% and 50.1%. However, it is false to say that there is a 95% chance that a randomly selected coworker has received the vaccine or that there is a 42% chance for a randomly selected coworker to have received the vaccine.
The approximate 95% confidence interval for the proportion of coworkers who have received this year's flu vaccine is (0.339, 0.501). Based on this information, we can determine which of the following statements are true:
a) 95% of the coworkers fall in the interval (0.339, 0.501).
This statement is true. The 95% confidence interval represents the range of values within which we can be 95% confident that the true proportion of coworkers who have received the flu vaccine lies. Therefore, we can say that 95% of the coworkers fall within the interval (0.339, 0.501).
b) We are 95% confident that the proportion of coworkers who have received this year's flu vaccine is between 33.9% and 50.1%.
This statement is true. The 95% confidence interval (0.339, 0.501) provides us with a range of values within which we can be 95% confident that the true proportion of coworkers who have received the flu vaccine lies. Therefore, we can say that we are 95% confident that the proportion of coworkers who have received the vaccine is between 33.9% and 50.1%.
c) There is a 95% chance that a randomly selected coworker has received the vaccine.
This statement is false. The 95% confidence interval does not represent a probability or chance for an individual coworker. It provides a range of values within which we can be 95% confident that the true proportion of coworkers who have received the flu vaccine lies. It does not give information about the likelihood of an individual coworker receiving the vaccine.
d) There is a 42% chance that a randomly selected coworker has received the vaccine.
This statement is false. The 42% represents the proportion of coworkers in the survey who have received the flu vaccine, but it does not represent the chance or probability for a randomly selected coworker to have received the vaccine. The 42% is a point estimate, not a probability.
e) We are 95% confident that between 33.9% and 50.1% of the samples will have a proportion near 42%.
This statement is false. The confidence interval (0.339, 0.501) does not directly provide information about the proportion of samples that will have a proportion near 42%. The confidence interval represents the range of values within which we can be 95% confident that the true proportion of coworkers who have received the flu vaccine lies, but it does not specifically address the proportion of samples near 42%.
To know more about confidence intervals, refer here:
https://brainly.com/question/32546207#
#SPJ11
A triangle has one angle that measures 20° and another that measures 50°. What kind of triangle is it?
Answers
Answer:
obtuse triange
Step-by-step explanation:
20+50=70 degrees
since a triangle adds up to 180 degrees, subtract 70 from 180 to find the measure of the final angle
180-70=110
110 degrees is obtuse since it's greater than 90, so the triangle is obtuse
a sign in the elevator of a college library indicates a limit of 16 persons. in addition, there is a weight limit of 2,500 pounds. assume that the average weight of students, faculty, and staff at this college is 155 pounds, that the standard deviation is 29 pounds, and that the distribution of weights of individuals on campus is approximately normal. a random sample of 16 persons from the campus will be selected.
Answers
The probability that a randomly selected group of 16 individuals from the campus will be selected is 0.8023 or 80.23%
Based on the sign in the elevator of the college library, the limit of 16 persons and weight limit of 2,500 pounds need to be adhered to. To ensure compliance with both limits, we need to consider both the number of people and their weight.
Assuming that the distribution of weights of individuals on campus is approximately normal with an average weight of 155 pounds and a standard deviation of 29 pounds, we can use this information to estimate the total weight of a group of 16 randomly selected individuals.
The total weight of a group of 16 individuals can be estimated as follows:
Total weight = 16 x average weight = 16 x 155 = 2480 pounds
To determine if this total weight is within the weight limit of 2,500 pounds, we need to consider the variability in the weights of the individuals. We can do this by calculating the standard deviation of the total weight using the following formula:
Standard deviation of total weight = square root of (n x variance)
where n is the sample size (16) and variance is the square of the standard deviation (29 squared).
Standard deviation of total weight = square root of (16 x 29^2) = 232.74
Using this standard deviation, we can calculate the probability that the total weight of the group of 16 individuals is less than or equal to the weight limit of 2,500 pounds:
Z-score = (2,500 - 2,480) / 232.74 = 0.86
Using a standard normal distribution table or calculator, we can find that the probability of a Z-score less than or equal to 0.86 is approximately 0.8023.
Therefore, the probability that a randomly selected group of 16 individuals from the campus will comply with both the number and weight limits in the elevator of the college library is approximately 0.8023 or 80.23%.
To know more about probability click on below link :
https://brainly.com/question/14210034#
#SPJ11
Wen says the function is continuous, because the number of people is unlimited. Is Wen right or wrong? Explain.
Answers
Answer: Wrong
Step-by-step explanation:
Continuous means every value is included. Since fractions of people do not exist, it cannot be continuous.
Wen is wrong.
It is given that ; according to Wen a function is continuous because number of people are unlimited.
We have to tell that whether Wen is right or wrong ?
What is a continuous function ?
A continuous function, is a function whose graph is continuous without any breaks or jumps.
We know that ;
A continuous function in calculus is continuous at a point x = a ; if the curve of the function does NOT break at the point x = a.
We can also say that , continuous means every value is included.
Since fractions of people don't exist, i.e., unlimited , So, it cannot be continuous.
To learn more about continuous function click here ;
https://brainly.com/question/23344689
#SPJ2
6) Practice: Using Visual Cues Label each part of the diagram. Then use your labels to complete the sentences. Square Root Notation √6 1. The expression √ means "the of b". 2. The exponent 1 symbol (√) stands for the 3. The number or expression under the radical symbol is called the
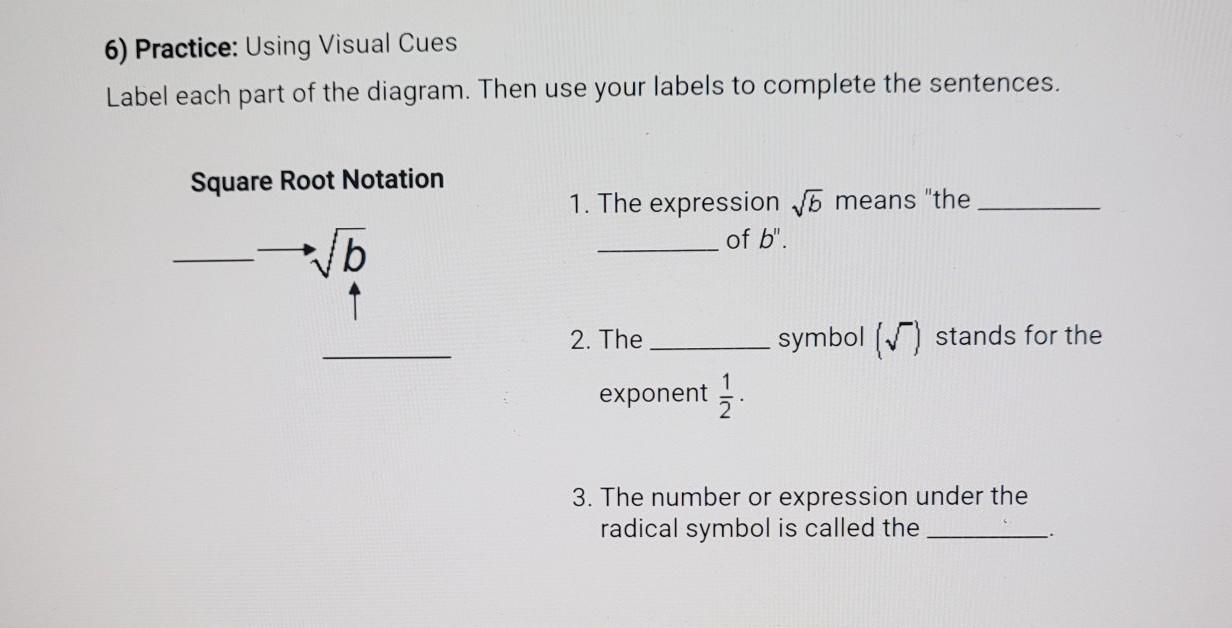
Answers
1. The expression √b means "the square root of b".
2. The radical symbol (√) stands for the exponent 1/2.
3. The number or expression under the radical symbol is called the radicand.
What is radicand?A radicand is the number or expression underneath a radical symbol (√). It is the number or expression that is being operated on by the root. The square root of the radicand is the result of the operation.
The expression √6 represents the square root of 6. This is the value of x that, when multiplied with itself, results in 6.
The square root of 6 is equal to 2.44948974, which is the positive solution to the equation x² = 6.
The radical symbol (√) indicates that the expression is a root and the number or expression under the radical symbol is called the radicand, which is 6 in this case.
The exponent of the radical symbol is 1/2, which implies that the expression is a square root.
For more questions related to expression
https://brainly.com/question/395066
#SPJ1
Question 3 The Schwarzschild metric is given by 2M 2M ds² -(₁-²M) di² + (1-²¹)- 1- dr² +r² (d0² + sin² 0 dó²). There are Killing vectors associated with time invariance and angular momen- tum invariance in the direction in this geometry leading to the conserved quantities e = (1-2) and l= r² sin² 0 dr From this one can derive an analog to the radial energy equation in Newtonian mechanics by orienting the coordinates so that the orbits are confined to the equatorial plane where 0 = π/2 and u = 0. One finds 2 1 dr + Veff (r) = E 2 dr (e²_ -1) where E = and Veft(r) = - + 2/²/²2 - Mp³². Further, for circular orbits one can show that M | [₁ + √/₁−12 (+1)]. r+= | 2M Finally, for circular orbits of radius R do 1/2 M dt R³ (a) Which value of r corresponds to the Schwarzschild radius of stable circular orbits: r or r? Justify your answer. [3 marks] (b) Show that for circular orbits of radius R do 1/2 M -1/2 3M (²) ¹² (1-³) dT R³ R where is the proper time. [6 marks] (c) A free particle is moving in a circular orbit around a spherical source of curvature of mass M. The Schwarzschild radius of the orbit is 8M. Use the equivalence principle to argue that the period as measured at infinity should be larger than that measured by the particle. [4 marks] (d) Find the period of the orbit as measured by an observer at infinity. Find the period of the orbit as measured by the particle. [7 marks] M
Answers
(A) Circular orbits of stable particles are possible at radii greater than three times the Schwarzschild radius for the non-rotating spherically symmetric mass.
This represents the radius of a black hole's event horizon, within which nothing can escape. The Schwarzschild radius is the event horizon radius of a black hole with mass M.
M can be calculated using the formula: r+ = 2Mwhere r+ is the radius of the event horizon.
(B) 1/2 M -1/2 3M (²) ¹² (1-³) dT = R³ R. This is the required expression.
Tau is the proper time of the particle moving around a circular orbit. Hence, by making use of the formula given above:1/2 M -1/2 3M (²) ¹² (1-³) dT = R³ dt.
(C) Time passes differently in different gravitational fields, and it follows that the period as measured at infinity should be larger than that measured by the particle.
The principle of equivalence can be defined as the connection between gravitational forces and the forces we observe in non-inertial frames of reference. It's basically the idea that an accelerating reference frame feels identical to a gravitational force.
(D) The period of the orbit as measured by an observer at infinity is 16π M^(1/2) and the period of the orbit as measured by the particle is 16π M^(1/2)(1 + 9/64 M²).
The period of orbit as measured by an observer at infinity can be calculated using the formula: T = 2π R³/2/√(M). Substitute the given values in the above formula: T = 2π (8M)³/2/√(M)= 16π M^(1/2).The period of the orbit as measured by the particle can be calculated using the formula: T = 2π R/√(1-3M/R).
Substitute the given values in the above formula: T = 2π (8M)/√(1-3M/(8M))= 16π M^(1/2)(1 + 9/64 M²).
To know more about Schwarzschild radius
https://brainly.com/question/29534114
#SPJ11
El valor de 40.7 es 10 veces el valor del 4.07 ¿tiene razon?
Answers
The requried 40.7 is 10 times the value of 4.07, which is correct.
What is simplification?The process in mathematics to operate and interpret the function to make the function or expression simple or more understandable is called simplifying and the process is called simplification.
Here,
Given that,
The value of 40.7 is 10 times the value of 4.07, we have to very whether the given expression is correct or not.
To verify, you can divide 40.7 by 10, which gives you 4.07.
So, 40.7/10 = 4.07.
Learn more about simplification here:
https://brainly.com/question/12501526
#SPJ1
Type a related division fact for 10 × 12 = 120.
Answers
Answer:
120 divided by 10 is 12. 120 divided by 12 is 10.
Simplify (2a+5)6a^2
Can someone help please
Answers
Answer:
12a^3+30a^2
Step-by-step explanation:
6a^2(2a+5)
12a^3+30a^2
A box contains 5 yellow balls, 3 blue, and 1 red ball. Two balls are drawn at random. Find the probability that the two balls are yellow
Answers
Answer:
5/18
Step-by-step explanation:
5/9 times 1/2
5/18
I may be wrong sorry
with reference to time series data patterns, a cyclical pattern is the component of the time series that
Answers
Answer:
Step-by-step explanation:
1012in a garden, the ratio of the areas of lawn to beds to paths is 3:1:1/2. Find the areas if the total area is 63m
Answers
Answer:
252
Step-by-step explanation:
it turns out that approximately 20% of the students in the sample already read the book as part of another class. obviously, these students are likely to perform better than students who did not already read the book. assuming that random assignment was used to ensure equivalent groups in the two conditions, this variable (i.e., whether or not subjects already read the book) should be considered:
Answers
The given problem falls under the extraneous type of variable.
What do you mean by an extraneous variable?To find out whether or not changing the values of an independent variable has an impact on a dependent variable is the entire objective of performing an experiment. Any variable that you're not interested in researching but that might also impact the dependent variable is an unnecessary variable. For instance, we would be interested in finding out how a basketball player's average points per game are influenced by the number of hours they exercise each week. The amount of time they spend stretching each week, though, is an unrelated factor that might also impact their points per game.
To learn more about variables, Visit:
https://brainly.com/question/28248724
#SPJ4
You are the owner of four Taco Bell restaurant locations. You have a business loan with Citizens Bank taken out 60 days ago that is due in 90 days. The amount of the loan is $50,000, and the rate is 9.5% using ordinary interest. You currently have some excess cash. You have the choice of sending Citizens $35,000 now as a partial payment on your loan or purchasing an additional $35,000 of serving supplies such as food containers, cups, and plastic dinnerware for your inventory at a special discount price that is "10% off" your normal cost of these items. (a)
Answers
Answer:
(a) The amount of interest you will save on this loan if you make the partial payment and don't purchase the additional serving supplies is $553.80.
(b) The amount you will save by purchasing the discounted serving supplies and not making the partial payment is $2,946.20.
Step-by-step explanation:
Note: This question is note complete. The complete question is therefore presented before answering the question as follows:
You are the owner of four Taco Bell restaurant locations. You have a business loan with Citizens Bank taken out 60 days ago that is due in 90 days. The amount of the loan is $50,000, and the rate is 9.5% using ordinary interest. You currently have some excess cash. You have the choice of sending Citizens $35,000 now as a partial payment on your loan or purchasing an additional $35,000 of serving supplies such as food containers, cups, and plastic dinnerware for your inventory at a special discount price that is "10% off" your normal cost of these items.
(a) How much interest will you save on this loan if you make the partial payment and don't purchase the additional serving supplies?
(b) How much will you save by purchasing the discounted serving supplies and not making the partial payment?
Explanation of the answers is now given as follows:
(a) How much interest will you save on this loan if you make the partial payment and don't purchase the additional serving supplies?
Since an ordinary interest is used, it implies that the total interest amount has to be calculated based on a 360-day year instead of a 365-day year. Therefore, the the interest saved can be calculated as follows:
I_$50K = PRT ........................ (1)
Where,
I_50K = Total Interest on the original $50,000 loan = ?
P = Principal = $50,000
R = interest rate = 9.5%
T = Number of days in the loan period / 360 = (60 + 90) / 360 = 150 / 360 = 0.416666666666667
Substituting the values into equation (1), we have:
I_$50K = $50,000 * 9.5% * 0.416666666666667
I_$50K = $1,979.17
Daily interest on $50,000 loan = I_$50K / Number of days in the loan period = $1,979.17 / 150 = $13.19
Also, we have:
I_PB = PRT ........................ (2)
Where,
I_PB = Innterest on principal balance = ?
P = Principal balance = $50,000 - $35,000 = $15,000
R = interest rate = 9.5%
T = Number of days remaining / 360 = 60 / 360 = 0.166666666666667
Substituting the values into equation (2), we have:
I_PB = $15,000 * 9.5% * 0.166666666666667
I_PB = $237.50
Daily interest on principal balance = I_PB / Number of days remaining the loan period = $237.50 / 60 = $3.96
Daily interest to save = Daily interest on $50,000 loan - Daily interest on principal balance = $13.19 - $3.96 = $9.23
Interest amount to save on the loan = Number of days remaining * Daily interest to save = 60 * $9.23 = $553.80
Therefore, the amount of interest you will save on this loan if you make the partial payment and don't purchase the additional serving supplies is $553.80.
(b) How much will you save by purchasing the discounted serving supplies and not making the partial payment?
This can be calculated as follows:
Discount receivable from purchasing serving supplies = Cost of serving supplies * Discount rate = $35,000 * 10% = $3,500
Amount to save by purchasing serving supplies = Discount receivable from purchasing serving supplies - Interest payable on loan for the remaining 60 days ................ (2)
Where;
Discount receivable from purchasing serving supplies = $3,500
Interest payable on loan for the remaining 60 days = Interest amount to save on the loan as calculated in part (a) = $553.80
Substituting the values into equation (2), we have:
Amount to save by purchasing serving supplies = $3,500 - $553.80 = $2,946.20
Therefore, the amount you will save by purchasing the discounted serving supplies and not making the partial payment is $2,946.20.
The table of values below represents a linear function and shows the amount of money in Juanita’s savings account since she began her part-time job. What is her monthly rate of savings?
Amount in Juanita’s Savings Account
Number of Months Working at Part-Time Job
0
2
4
6
8
Amount in Savings Account (in dollars)
$36
$60
$84
$108
$132
a.$12 per month
b.$18 per month
c.$24 per month
d.$36 per month
Answers
Answer:
The answer is C., $24 per month.
Answer:
A $12
Step-by-step explanation:
she has saved $36 before she gets a part time job, after 2 months, her savings have increased from 36 to 60. 2 months = 60 - 36, 2 months = 24. now we divide 24 by 2, and the answer is 12. option A is correct.
One month before an election, a poll of 630 randomly selected voters showed 55% planning to vote for a certain candidate. A week later it became known that he had had an extramarital affair, and a new poll showed only 53% of 1010 voters supporting him. Do these results indicate a decrease in voter support for his candidacy?
Determine the test statistic. z= (Round to two decimal places as needed.)
Find the P-value.
estimate that difference, p1−p2, with a 95% confidence interval
Answers
The statistics are as follows:
- Test Statistic: The calculated test statistic is approximately 1.02.
- P-value: The P-value associated with the test statistic of 1.02 is approximately 0.154.
- Confidence Interval: The 95% confidence interval for the difference in proportions is approximately -0.0186 to 0.0786.
To solve the problem completely, let's go through each step in detail:
1. Test Statistic:
The test statistic can be calculated using the formula:
z = (p1 - p2) / √[(p_cap1 * (1 - p-cap1) / n1) + (p_cap2 * (1 - p_cap2) / n2)]
We have:
p1 = 0.55 (proportion in the first poll)
p2 = 0.53 (proportion in the second poll)
n1 = 630 (sample size of the first poll)
n2 = 1010 (sample size of the second poll)
Substituting these values into the formula, we get:
z = (0.55 - 0.53) / √[(0.55 * (1 - 0.55) / 630) + (0.53 * (1 - 0.53) / 1010)]
z = 0.02 / √[(0.55 * 0.45 / 630) + (0.53 * 0.47 / 1010)]
z ≈ 0.02 / √(0.0001386 + 0.0002493)
z ≈ 0.02 / √0.0003879
z ≈ 0.02 / 0.0197
z ≈ 1.02 (rounded to two decimal places)
Therefore, the test statistic is approximately 1.02.
2. P-value:
To find the P-value, we need to determine the probability of observing a test statistic as extreme as 1.02 or more extreme under the null hypothesis. We can consult a standard normal distribution table or use statistical software.
The P-value associated with a test statistic of 1.02 is approximately 0.154, which means there is a 15.4% chance of observing a difference in proportions as extreme as 1.02 or greater under the null hypothesis.
3. Confidence Interval:
To estimate the difference in proportions with a 95% confidence interval, we can use the formula:
(p1 - p2) ± z * √[(p_cap1 * (1 - p_cap1) / n1) + (p_cap2 * (1 - p_cap2) / n2)]
We have:
p1 = 0.55 (proportion in the first poll)
p2 = 0.53 (proportion in the second poll)
n1 = 630 (sample size of the first poll)
n2 = 1010 (sample size of the second poll)
z = 1.96 (for a 95% confidence interval)
Substituting these values into the formula, we get:
(0.55 - 0.53) ± 1.96 * √[(0.55 * (1 - 0.55) / 630) + (0.53 * (1 - 0.53) / 1010)]
0.02 ± 1.96 * √[(0.55 * 0.45 / 630) + (0.53 * 0.47 / 1010)]
0.02 ± 1.96 * √(0.0001386 + 0.0002493)
0.02 ± 1.96 * √0.0003879
0.02 ± 1.96 * 0.0197
0.02 ± 0.0386
The 95% confidence interval for the difference in proportions is approximately (0.02 - 0.0386) to (0.02 + 0.0386), which simplifies to (-0.0186 to 0.0786).
To know more about confidence intervals, refer here:
https://brainly.com/question/32546207#
#SPJ11
A water park offers two types of membership an unlimited use for $70 per month or a monthly $10 fee and $5 per visit which is the better plan?
Answers
Two membership options are available at a water park: unlimited use for $70 per month or $10 per month plus $5 per visit. 12 visits are the preferred schedule.
AQUAPARK THE BIGGEST IN THE NETHERLANDS! An incredible sight to behold a floating playground island that is 2,000 square meters in size and made up of various floating playsets. For anyone who like climbing, sliding, and jumping, this is a veritable wonderland. Malta's waters received a 96.6% outstanding rating in the assessment and are renowned for their clear waters and undeveloped coasts. Look no farther if you're searching for one of Europe's cleanest open-water locations. Siam Park is the place to go if you want a rush of adrenaline and fast-moving water rapids. While travelling with children, Aqualand is a great option if you're seeking for more family-friendly rides.
The cost of a $10 monthly charge plus $5 for each visit can be calculated as follows:
Cost = 10 + 5*v
where v denotes the monthly visits.
If both membership levels have the same price, then:
\(10 + 5*v = 70\)
\(5*v = 70 - 10\)
\(v = 60/5v = 12\)
To know more about water park here
https://brainly.com/question/28517101
#SPJ4
The time length in hours of a certain airplane flight can be represented by the function f(x) = 0. 0025x 0. 75, where x is the number of miles for the flight. The time length of another airplane flight in hours can be represented by the function g(x) = 0. 004x 0. 5, where x is the number of miles for the flight. Which function represents the sum of the flight time lengths, h(x) = f(x) g(x)? h(x) = –0. 029x 0. 25 h(x) = 0. 0065x – 1. 25 h(x) = 0. 029x – 0. 25 h(x) = 0. 0065x 1. 25.
Answers
The function h(x) = 0.0065x – 1.25 represents the sum of the flight time lengths of two airplane flights.
To find the sum of the flight time lengths represented by the functions f(x) and g(x), we need to multiply the functions together. Given f(x) = 0.0025x^0.75 and g(x) = 0.004x^0.5, the function h(x) = f(x) * g(x) represents the sum.
Multiplying the functions:
h(x) = (0.0025x^0.75) * (0.004x^0.5)
Simplifying the expression:
h(x) = 0.0025 * 0.004 * x^0.75 * x^0.5
h(x) = 0.0065x^1.25
Therefore, the function h(x) = 0.0065x – 1.25 represents the sum of the flight time lengths of the two airplane flights.
Understanding how to multiply functions and simplify the resulting expression allows us to determine the correct function that represents the sum. By multiplying the functions f(x) and g(x) together, we obtain the function h(x), which represents the desired sum of the flight time lengths.
Learn more about function: brainly.com/question/11624077
#SPJ11
Answer:
0.0065x + 1.25
Step-by-step explanation:
A researcher is using bootstrapping methods to estimate the mean IQ in the population of all students at one large university. What will the mean of his bootstrap sampling distribution be approximately equal to?
Answers
The mean of the researcher's bootstrap sampling distribution will be approximately equal to the sample mean IQ of the students at the university.
Bootstrap is a resampling strategy that relies on random sampling with replacement. A researcher who is estimating the mean IQ of all students at a university would take a random sample of n students, compute the mean IQ of the sample, and repeat this process many times. The sample mean of IQ will be a summary statistic that we are trying to estimate.
The researcher will repeat this process of sampling and computing the sample mean many times, thereby generating a bootstrap sampling distribution. The mean of this distribution will be approximately equal to the mean of the student population's IQ. The sample size must be large enough to produce an accurate estimate of the population's mean.
In conclusion, the researcher will generate a bootstrap sampling distribution by taking many random samples of n students and computing the sample mean IQ of each sample. The mean of this distribution will be approximately equal to the sample mean IQ of the students at the university.
Learn more about Bootstrap here:
https://brainly.com/question/31851368
#SPJ11
which statistical test is most appropriate to determine if there is a significant difference between the means of two independent groups, given a specific level of significance and assuming that population variance are equal?
Answers
The independent t-test is most appropriate to determine if there is a significant difference between the means of two independent groups.
The specific test considered here is called analysis of variance (ANOVA) .
The independent t-test, also called the two sample t-test, independent-samples t-test or student's t-test, is an inferential statistical test that determines whether there is a statistically significant difference between the means in two unrelated groups.
The specific test considered here is called analysis of variance (ANOVA) and is a test of hypothesis that is appropriate to compare means of a continuous variable in two or more independent comparison groups.
Learn more about Statistical test at:
https://brainly.com/question/31746962
#SPJ4
Find the amount accumulated after investing a principal P for t years at an interest rate compounded k times per year. P = $3,500 r= 5% t = 10 k = 4
A = ?

Answers
Answer:
To the nearest cent, the accumulated amount over the 10 years is $5,752.67
Step-by-step explanation:
Here, we want to use the given formula to find the accumulated amount on the deposit/investment
we have this as follows;
A = P(1 + r/k)^kt
P is the amount invested which is the principal = $3,500
r is the interest rate = 5% = 5/100 = 0.05
k is the number of times interest is compounded yearly = 4
t is the number of years = 10
Substituting these values in the formula, we have;
A = 3500(1 + 0.05/4)^(4 * 10)
A = 3500 (1 + 0.0125)^40
A = 3500(1.0125)^40
A = $5,752.67
What is the area of a circle with the radius of 16 meters?
Answers
Answer: 804 meters
Step-by-step explanation:
Area of a circle = πr²
Radius = 16m
π(16)² = 804.2477193 meters