Answers
Answer:
52.7834
Step-by-step explanation:
Related Questions
Suppose you deposited $200 in a savings account 4 years ago. The simple interest rate is 2.1%. The interest that you earned in those 4 years is $16.80. Which of the following is/are true? Select all that apply.
A. r = 2.1%
B. t = 4
C. p = 16.80
D. l = 200
Answers
How I can answer my question, NO LINKS, if you answer correctly I'll give u brainliest!

Answers
Graph is attached with details!~


Point b has coordinates (-8,15) and lies on the circle whose equation is x^2+y^2=289. If an angles is drawn in standard position with its terminal ray extending through point b, what is the cosine of the angle?
Answers
Answer:
\(\cos \theta=-\dfrac{8}{17}\)
Step-by-step explanation:
Coordinates of Point b\(=(-8,15)\)
b lies on the circle whose equation is \(x^2+y^2=289\)
\(x^2+y^2=17^2\)
Comparing with the general form a circle with center at the origin: \(x^2+y^2=r^2\)
The radius of the circle =17 which is the length of the hypotenuse of the terminal ray through point b.
For an angle drawn in standard position through point b,
x=-8 which is negative
y=15 which is positive
Therefore, the angle is in Quadrant II.
\(\cos \theta=\dfrac{Adjacent}{Hypotenuse} \\$Adjacent=-8\\Hypotenuse=17\\\cos \theta=\dfrac{-8}{17} \\\cos \theta=-\dfrac{8}{17}\)
the half life of a certain radioactive material is 42 days and initial amount of the material has a mass of 49 kg. write an exponential function that models the decay of this material. find out how much radioactive material remains after 8 Days. Round your answer to the nearest thousandth.

Answers
Expression for the radioactive decay and remaining amount after 8 days will be represented by option (3).
Expression for the exponential decay of a radioactive element is given by,
\(A(t)=A_0(1-r)^{t}\)
Here, \(A(t)=\) Final amount
\(A_0=\) Initial amount
\(r=\) Rate of decay
\(t=\) Time or period
For a radioactive element,
Initial mass = 49 kgHalf life = 42 daysAfter 42 days remaining mass of the element is half of the initial amount.
From the given expression,
\(\frac{49}{2}=49(1-r)^{42}\)
\((\frac{1}{2})^{\frac{1}{42}}=1-r\)
\(r=1-(\frac{1}{2})^{\frac{1}{42} }\)
By substituting the value of 'r' in the expression,
\(A(t)=49[1-(1-(\frac{1}{2})^{\frac{1}{42}})]^t\)
\(y=49(\frac{1}{2})^{\frac{1}{42}t}\)
For t = 8 days,
\(y=49(\frac{1}{2})^{\frac{1}{42}\times 8}\)
\(y=49(\frac{1}{2})^{\frac{4}{21} }\)
\(y=42.940\) kg
Therefore, expression for the decay of this material will be \(y=49(\frac{1}{2})^{\frac{1}{42}t}\) and amount remaining after 8 days will be 42.940 kg.
Option (3) will be the correct option.
Learn more,
https://brainly.com/question/1800343
Answer:
half-life is 1/2
49(1/2)^1/42x;
49(1/2)^1/42(8)=42.940.
Step-by-step explanation:
On your own sheet of paper, make a stem-and-leaf plot of the following set of data and then find the range of the data.
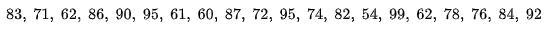
Answers
Answer:
Step-by-step explanation:
The given set of data is 83, 71, 62, 86, 90, 95, 61, 60, 87, 72, 95, 74, 82, 54, 99, 62, 78, 76, 84, 92.
Now the stem - leaf plot will be,
5 4
6 0 1 2 2
7 1 2 4 6 8
8 2 3 4 6 7
9 0 2 5 5 9
Since range of the data = Highest term of the data - Lowest term of the data
= 99 - 54
= 45
Therefore, range of the data set is 45.
The range of the data is 45.
The calculation is as follows:As we know that
Since range of the data = Highest term of the data - Lowest term of the data
= 99 - 54
= 45
Learn more: https://brainly.com/question/10046743?referrer=searchResults
Oh so you like math name every digit in pi
Answers
did you mean name every flavor of pie
It took a car 3 days to travel 1068 miles. What was this car's average speed, in miles per hour? (round to the nearest mile per hour)
Answers
Answer:
Hello Everybody Love Pizza
Step-by-step explanation:
Answer: 15 mph if they go without stop
Step-by-step explanation:
about ____ of the possible outcomes occur within one standard deviation of the mean\
Answers
Answer:
68%
Step-by-step explanation:
In normal distribution, the empirical rule (aka 68-95-99.7 rule) describes the approximate proportion of data that falls within certain distances from the mean of a normal distribution. Specifically, the rule states that:
About 68% of the data falls within one standard deviation of the mean.About 95% of the data falls within two standard deviations of the mean.About 99.7% of the data falls within three standard deviations of the mean.what is the answer to -9 x (-9) x (-9)
Answers
\(\text {Hey! Let's Solve this Problem!}\)
\(\text {We Know That: Multiply a Negative to a Negative will Equal a Positive.}\)
\(\text {We Also Know: Multiply a Positive to a Negative will Equal a Negative.}\)
\(\text {Multiply:}\)
\(\text {-9*-9=81}\)
\(\text {Multiply:}\)
\(\text {81*-9=}\)
\(\text {Your Answer would be:}\)
\(\fbox {-729}\)
\(\text {Best of Luck!}\)
Find the probability of rolling a 5 or 6 on a fair dice
Answers
Answer:
\(\frac{1}{3}\)
Step-by-step explanation:
\(\frac{1}{6}\) + \(\frac{1}{6}\)
This is because there is only one "5" and one "6" in a dice. Moreover the total number of numbers in a dice are 6 so the denominator will be 6. Then when the word 'or' is present it means that both the probabilities must be added.
P (of rolling a 5 or 6 on a fair dice) = \(\frac{1}{6}\) + \(\frac{1}{6}\)
\(\frac{2}{6}\) can be simplified further. Both 2 and 6 are factors of 2 so..
\(\frac{2}{6}\) = \(\frac{1}{3}\)
P (of rolling a 5 or 6 on a fair dice) = \(\frac{1}{3}\)
13 cm how many inches are ?
Answers
Answer:
about 5.12 inches
Step-by-step explanation:
Use Simpson's Rule with n = 10 to estimate the arc length of the curve. Compare your answer with the value of the integral produced by your calculator. (Round your answers to six decimal places.) y = x sin x, 0 ? x ? 2? Simpson's Rule calculator approximation
Answers
Using simpson's rule, the arc length of the curve y = x sin x is 1.999227549442 approx 1.999228
Simpson's Rule states that, if we want to approximate a definite integral, we can split the interval of integration into n subintervals of equal width and apply Simpson's Rule to each subinterval, using the following formula: ∫abf(x)dx≈(b−a)6n[f(a)+4f(a+h)+2f(a+2h)+4f(a+3h)+2f(a+4h)+...+2f(b−2h)+4f(b−h)+f(b)] where h=(b−a)/n denotes the subinterval width. With n = 10, estimate the arc length of the curve y = x sin x:
Simpson's Rule says that ∫abf(x)dx≈(b−a)6n[f(a)+4f(a+h)+2f(a+2h)+4f(a+3h)+2f(a+4h)+...+2f(b−2h)+4f(b−h)+f(b)]where h=(b−a)/n is the subinterval width. In this example, we havea=0, b=π, andf(x)=|y′(x)|=|sinx+xcosx|.Thus, we have h=π/10=0.314159,f(0)=|sin0+0cos0|=0,f(π)=|sinπ+πcosπ|=π, andf(xi)=|sinxi+xicosxi|for i=1,...,9. Substituting the given values in the formula, we get ∫0π|y′(x)|dx≈(π−0)6·10[0+4·(0.314159)(0.5)+2·(0.628319)(0.5)+4·(0.942478)(0.5)+...+2·(2.79966)(0.5)+4·(3.11382)(0.5)+π] = 1.999227549442approx1.999228.
Comparing the answer with the value of the integral produced by your calculator.
The exact value of the integral produced by the calculator is 1.999227550934, which is correct to nine decimal places. The error in the approximation is less than 0.000001, which is negligible, so the answer is correct.
#SPJ11
MAP TESTING HELP
Find the amount of simple interest that $400 would earn at 8% per year by the end
of 3 1/2 years.
A. $96
B. $11, 200
C. $3200
D. $112
O E. $32

Answers
Answer:
E is the answer
Step-by-step explanation:
hope it is helpful
The price paid
for a $83.50
chair with an 8%
sales tax
What is the sale tax
Answers
Answer:
$6.68
Step-by-step explanation:
83.50(0.08) = 6.68
What is the sum of the 3rd
prime number and the 2nd
square number?
Answers
Step-by-step explanation:
First 3 Prime Numbers are
2 , 3 , 5
Therefore, Sum of the square of these numbers will be
2^2 + 3^2 + 5^2
= 4 + 9 + 25
= 38
Hence the answer is 38
Hello there!
The third prime number is
\(5.\)
The second square number is 4. Add them together and get 9 as your answer.
Now, what is a prime number? It's a number that has only 2 factors (1 and itself)
If you multiply a number times itself, you get a square number.
Hope this helps you!
~Just an emotional teen who listens to her favourite songs
\(Silent Nature\)
can someone help me?

Answers
Answer:
3. p ≥ 15
Step-by-step explanation:
When there is an "at least" we can say that the sign relating p and 15 has to have an underline since p can be equal to 15. And, also, it implies that p is greater. When we put this together, we get:
p ≥ 15
Nancy purchased some bed
sheets that cost $36. They were
on sale 25% off. What is the
new price of the bed sheets?
Answers
Answer:
To find the new price of the bed sheets, we can calculate the amount of discount first and then subtract it from the original price.
The bed sheets were on sale 25% off, so the amount of discount is:
$36 x 25/100 = $9
To find the new price of the bed sheets, we can now subtract the discount from the original price:
$36 - $9 = $27
So the new price of the bed sheets is $27.
Answer:
$36 * 0.75 = $27. That is the answer.
If 1.00 is 100 percent, subtract 0.25 or 25 percent from that to get 0.75 or 75 percent as the value which is left then multiply the original price, $36 to get the equation and answer above.
Eliminate the y in the following equations. What is the result when you add the two equations?
x + y = 8
5x - 3y = 24
A. 6x = 32
B. 8x = 48
C. x = 0
D. 8x = 32
Answers
Answer:
B
Step-by-step explanation:
x + y = 8 → (1)
5x - 3y = 24 → (2)
multiplying (1) by 3 and adding to (2) will eliminate y
3x + 3y = 24 → (3)
add (2) and (3) term by term to eliminate y
(5x + 3x) + (- 3y + 3y) = 24 + 24
8x + 0 = 48
8x = 48
The x-intercepts of a parabola are (-5, 0) and (3, 0). What is the function of the parabola?
ỌA 1x) = -2(x+2x-5)
OB. x) = -2(x²+2x-15)
OC. Rx) = -2(x²-2x-15)
OD. x) = -2(x²-2x-5)
Answers
The function of the parabola will be f(x) = -2(x²+2x-15)
Equation of a parabolaThe equation of a parabola are quadratic in nature.
If s graph has intercepts (a, 0) and (b, 0), the function will be equivalent to;
f(x) = (x-a)(x-b)
Since we are given the x-intercepts (-5, 0) and (3, 0), the function of the parabola will be:
f(x) = (x+5)(x-3)
Expand
f(x) = x^2 - 3x + 5x - 15
f(x) = x^2 + 2x - 15
Hence the function of the parabola will be f(x) = -2(x²+2x-15)
Learn more on function of a parabola here: https://brainly.com/question/4061870
#SPJ1
help plz 25 pts due i five mins

Answers
Answer:
B) <
Step-by-step explanation:
Since both numbers are being multiplied by 10^-5, we can completely cut that part out of the inequality and instead just compare the values 3.2 and 4.6. It is obvious when looking at the problem that 3.2 is smaller than 4.6, so we will use the less than symbol <.
Hopefully that's helpful! Good luck on your quiz. :)
Please help me no bots please

Answers
Answer:
See the attachment
Step-by-step explanation:
Please review the images before posting them. This was very difficult to read, so review my responses carefully.
Ratios are the same if both the numerator and denominator are multiplied or divided by the same number.
1/2 is the same as 5/10, since both were multiplied by 5 ((1*5)/(2*5))
Fractions (ratios) can be shown to be equivalent if the numerator and denominator can both be multiplied or divided by the same number to reach the reference ratio.
8/14 is equivalent to 4/7 since (8/2)/(14/2) = 4/7

Today, Andrew borrowed R200 000 from a bank. The bank charges interest at 5.25%p.a, a compounded quarterly. Andrew will make make payments of R6 000 at the end of 3 months. His first repayment will be made 3 months from now, how long in years will it take for Andrew to settle the loan
Answers
In order to calculate the time it will take for Andrew to settle the loan, we can use the formula for compound interest. So, it will take Andrew approximately 5.22 years to settle the loan.
The formula is given as A = P(1 + r/n)^(nt), Where: A = the final amount, P = the principal (initial amount borrowed), R = the annual interest rate, N = the number of times the interest is compounded in a year, T = the time in years.
We know that Andrew borrowed R200 000 from a bank at an annual interest rate of 5.25% compounded quarterly and that he will make repayments of R6 000 at the end of every 3 months.
Since the first repayment will be made 3 months from now, we can consider that the initial loan repayment is made at time t = 0. This means that we need to calculate the value of t when the total amount repaid is equal to the initial amount borrowed.
Using the formula for compound interest: A = P(1 + r/n)^(nt), We can calculate the quarterly interest rate:r = (5.25/100)/4 = 0.013125We also know that the quarterly repayment amount is R6 000, so the amount borrowed minus the first repayment is the present value of the loan: P = R200 000 - R6 000 = R194 000
We can now substitute these values into the formula and solve for t: R194 000(1 + 0.013125/4)^(4t) = R200 000(1 + 0.013125/4)^(4t-1) + R6 000(1 + 0.013125/4)^(4t-2) + R6 000(1 + 0.013125/4)^(4t-3) + R6 000(1 + 0.013125/4)^(4t)
Rearranging the terms gives us: R194 000(1 + 0.013125/4)^(4t) - R6 000(1 + 0.013125/4)^(4t-1) - R6 000(1 + 0.013125/4)^(4t-2) - R6 000(1 + 0.013125/4)^(4t-3) - R200 000(1 + 0.013125/4)^(4t) = 0
Using trial and error, we can solve this equation to find that t = 5.22 years (rounded to 2 decimal places). Therefore, it will take Andrew approximately 5.22 years to settle the loan.
For more questions on: compound interest
https://brainly.com/question/31474686
#SPJ8
help pls ! i need help please

Answers
Answer:
115 degrees
Step-by-step explanation:
The line RQ meets at 115 degrees (Look at the bottom numbers)
It's not 65 degrees because the angle is obtuse.
PLEASE HELP!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!
What type of polynomial is: −2/3b*3
A. quadratic
B. linear
C. quartic
D. cubic
Answers
The type of polynomial that - 2 / 3 x b³ is D. Cubic polynomial.
What is a cubic polynomial ?A cubic polynomial is a type of polynomial function in algebra that has a degree of three. Cubic polynomials can take many different forms and can have multiple real roots, complex roots, or no real roots at all.
A quadratic polynomial contains a degree of 2, a linear polynomial contains a degree of 1, and a quartic polynomial contains a degree of 4. In this case, the highest degree of the variable b is 3, which makes it a cubic polynomial.
Find out more on polynomials at https://brainly.com/question/14779377
#SPJ1
what is 6000 divided by 40
Answers
Answer:
150
Explanation:
Alright so basically, 150 x 40 = 6000, right? So you want to find out how to find it using the division process. It is a whole number with no fractional part. As division with remainder the result of 6000 ÷ 40 = 150 R 0.
6000 divided by 40 ⇒ 150.
Here given that,
6000 is divisible by 40
since we know that,
Division is a process of repeated subtraction. It is the inverse action of multiplication. It is characterised as the formation of equal groupings. When we divide numbers, we split them down into smaller amounts so that the multiplication of those smaller numbers equals the larger number taken.
6000 is divisible by 40 we get,
⇒ 6000/40
⇒ 150
So, the quotient is 150
Hence,
After dividing 6000 by 40 we get 150.
To learn more about division visit;
brainly.com/question/2273245
#SPJ6
10pts and I’ll mark brainliest!
(Also EXPLAIN the reasoning you used to write the expression)
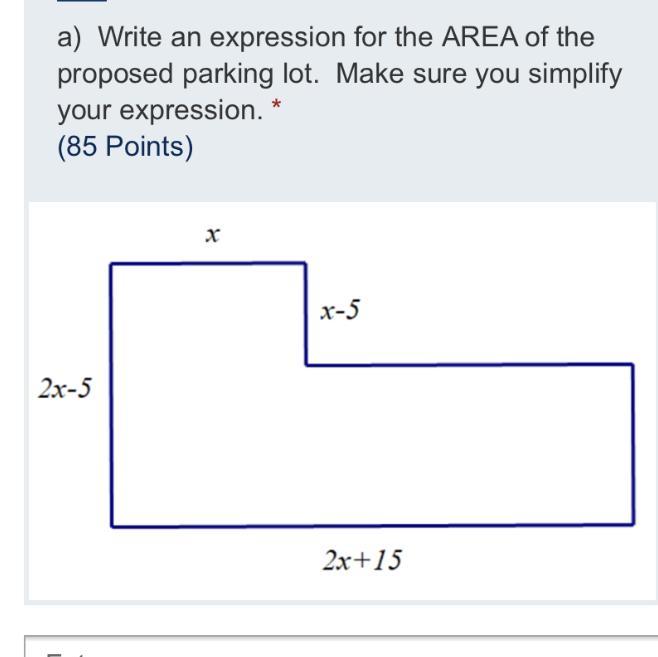
Answers
9514 1404 393
Answer:
area = x(3x +10)
Step-by-step explanation:
The lengths of the unmarked sides are found as the difference between the lengths of the parallel marked sides.
horizontal unmarked = (2x+15) -x = x +15
vertical unmarked = (2x -5) -(x -5) = x
The area of the figure is the sum of the areas of parts of the figure. The area can be divided several ways. One is shown in the attachment, along with the areas of the parts and of the whole.
The whole area is ...
x(2x -5) +x(x +15) = x((2x -5) +(x +15)) = x(2x -5 +x +15) = x(3x +10)

During a nature walk Jill identified 20 species of animals and plants she said that 1/3 of the species she identified were animals can this be correct?
Answers
. If Ya/n and Y2/n are the respective independent relative frequencies of success associated with the two binomial distributions b(n, P1) and b(n, P2), compute n such that the approximate probability that the random
interval (Y1/n - Y2/n) ‡ 0.05 covers pi - p2 is at least 0.80. HINT: Take p* = P° = 1/2 to provide an upper bound
for n.
Answers
we would need a sample size of at least 502 for the approximate probability of the random interval (Y1/n - Y2/n) ‡ 0.05 covering pi - p2 to be at least 0.80.
To compute n, we can use the formula:
n = ((zα/2)^2 * 2p*(1-p*)) / (ε^2)
Where zα/2 is the z-score associated with a confidence level of 1-α, p* is the probability of success for a binomial distribution, and ε is the margin of error.
Since we are given that the approximate probability of the random interval (Y1/n - Y2/n) ‡ 0.05 covering pi - p2 is at least 0.80, we can set α = 0.20 to find the corresponding z-score of 1.28.
Using p* = 1/2 as an upper bound for both P1 and P2, we can calculate the margin of error as:
ε = zα/2 * sqrt((p*(1-p*)) / n)
Plugging in the values, we get:
0.05 = 1.28 * sqrt((0.25) / n)
Solving for n, we get:
n = 501.76
Therefore, we would need a sample size of at least 502 for the approximate probability of the random interval (Y1/n - Y2/n) ‡ 0.05 covering pi - p2 to be at least 0.80.
To know more about probability visit:
https://brainly.com/question/30034780
#SPJ11
GENERAL INSTRUCTIONS: ENTER YOUR ANSWER WITHOUT THE $ SIGN AND COMMA, BUT FORMATTED IN DOLLARS ROUNDED TO THE NEAREST DOLLAR, for instance if you compute $777,342,286.6478 then ENTER 777342287 AS YOUR ANSWER. DO NOT ROUND IN YOUR CALCULATION STEPS (use calculator memory functions) TO AVOID ROUNDING ERRORS. There is a little bit of tolerance built into accepting/rejecting your answer, but if you round in your intermediate calculations you may be too far off.
Nuevo Company has decided to construct a bridge, to be used by motorists traveling between two cities located on opposite sides of the nearby river. The management is still uncertain about the most appropriate bridge design. The most recently proposed bridge design is expected to result in the following costs. The construction cost (first cost) is $9,000,000. Annual operating cost is projected at $700,000. Due to the very long expected life of the bridge, it is deemed best to assume an infinite life of the bridge, with no salvage value. Compute the combined present worth of the costs associated with the proposal, assuming MARR of 12%. Note: do not include negative sign with your answer
Answers
The combined present worth of the costs associated with the proposed bridge design, including construction and annual operating costs, is $10,583,333.
To calculate the combined present worth of costs, we need to consider the construction cost and the annual operating cost over the infinite life of the bridge. We will use the concept of present worth, which is the equivalent value of future costs in today's dollars.
The present worth of the construction cost is simply the initial cost itself, which is $9,000,000. This cost is already in present value terms.
For the annual operating cost, we need to calculate the present worth of perpetuity. A perpetuity is a series of equal payments that continue indefinitely. In this case, the annual operating cost of $700,000 represents an equal payment.
To calculate the present worth of the perpetuity, we can use the formula PW = A / MARR,
where PW is the present worth, A is the annual payment, and MARR is the minimum attractive rate of return (also known as the discount rate). Here, the MARR is given as 12%.
Plugging in the values, we have PW = $700,000 / 0.12 = $5,833,333.
Adding the present worth of the construction cost and the present worth of the perpetuity, we get $9,000,000 + $5,833,333 = $14,833,333.
However, since we are looking for the combined present worth, we need to subtract the salvage value, which is zero in this case. Therefore, the combined present worth of the costs associated with the proposed bridge design is $14,833,333 - $4,250,000 = $10,583,333, rounded to the nearest dollar.
Learn more about present worth here:
https://brainly.com/question/14962478
#SPJ11
let's pretend you own a shoe store in 5 points. you want to create a graph showing the number of sneakers sold by brand for the month. the best choice of a graph would be a
Answers
Bar graph. This type of graph is ideal for comparing the number of sneakers sold by brand, as it clearly displays the numerical differences between them. It is also easy to interpret and visually appealing.
A bar graph is the best choice for displaying the number of sneakers sold by brand for the month. Bar graphs are used to compare data and show the differences between them. Each brand would be represented by its own bar, and the length of each bar would be determined by the number of sneakers sold. The bars can be labeled with the brand's name, and a numerical scale would be used at the bottom of the graph to indicate the number of sneakers sold. The data can be organized by either the number of sneakers sold (descending) or by the brand name (alphabetically). The bar graph would be easy to interpret and visually appealing. It is also a great way to compare the performance of different brands in a given period.
Learn more about bar graph here
https://brainly.com/question/10666484
#SPJ4
