A square flower garden is surrounded by a brick walkway that is 1.8 m wide. The area of the walkway is equal to the area of the garden. Determine the dimensions of the garden to the nearest tenth of a metre.
Answers
The length of the garden is 4.3 m while the width is 4.3 m
Let x represent the length of the garden. Therefore:
The area of the garden = x × x = x²
The width of the walkaway is 1.8 m, hence the length of the garden and walkaway = x + 1.8. The width of the garden and walkaway = x + 1.8.
Hence the area of the garden and walkaway = (x + 1.8) × (x + 1.8) = x² + 3.6x + 3.24
The area of walkaway = (x² + 3.6x + 3.24) - x² = 3.6x + 3.24
Since the area of the walkway is equal to the area of the garden, hence:
3.6x + 3.24 = x²
x² -3.6x - 3.24 = 0
x= 4.34 m or -0.74 m
Since the length cannot be negative, hence x = 4.3 m
Therefore the garden is 4.3 m by 4.3 m
Find out more at: https://brainly.com/question/2077866
Related Questions
PLS HELP ASAP ILL GIVE BRAINLIEST THANKS

Answers
Answer:
Adding 16 on both sides
Step-by-step explanation:
The first step would be adding 16 on both sides to isolate the 5r and find the value of r
Which means you were correct, good job!
How do you find the inverse of a 3 matrix?
Answers
Answer:
Inverse of a 3 by 3 Matrix
MM-1 = M-1 M = I.
Step 1: The first step while finding the inverse matrix is to check whether the given matrix is invertible. ...
Step 2: Calculate the determinant of 2 × 2 minor matrices.
Step 3: Formulate the cofactor matrix.
Step-by-step explanation:
The inverse matrix formula, A-¹ = (1/|A|) Adj A, is used to determine the inverse of a 3×3 matrix.
What is Inverse of Matrix ?The multiplicative identity is obtained by multiplying the provided matrix by the other matrix which serves as the inverse of a matrix. A matrix's inverse is A-1, since the I is the identity matrix, A A-1 = A-1 A = I.
How can I determine the 3X3 Matrix's inverse?The formula A-1 = (adj A)/(det A), where det A is in the denominator, is used to determine the inverse of a 3x3 matrix A.
• adj A = The adjoint matrix of A
• det A = determinant of A
dAs a result, det A should not be 0 for A-1 to exist. i.e.,
• A-1 exists when det A ≠ 0 (i.e., when A is nonsingular)
• A-1 does not exist when det A = 0 (i.e., when A is singular).
To know more about inverse matrix :
https://brainly.com/question/4017205
#SPJ4
(3c-5d)²EXPAND IT PLEASE
Answers
Answer:
9c^2 - 30cd + 25d^2
Step-by-step explanation:
(3c - 5d)^2
= (3c - 5d) (3c - 5d)
= [3c * 3c] + [3c * -5d] + [-5d * 3c] + [-5d * -5d]
= 9c^2 - 30cd + 25d^2
7. Ifa = 3an * db = - 2 . find the values of: (a + b)ab
Answers
The Values of (a+b)ab are undefined.
Given that, a = 3an and db = -2We need to find the values of (a+b)
Now, we have a = 3an... equation (1)Also, we have db = -2... equation (2)From equation (1), we get: n = 1/3... equation (3)Putting equation (3) in equation (1), we get: a = a/3a = 3... equation (4)Now, putting equation (4) in equation (1), we get: a = 3an... 3 = 3(1/3)n = 1
From equation (2), we have: db = -2=> d = -2/b... equation (5)Multiplying equation (1) and equation (2), we get: a*db = 3an * -2=> ab = -6n... equation (6)Putting values of n and a in equation (6), we get: ab = -6*1=> ab = -6... equation (7)Now, we need to find the value of (a+b).For this, we add equations (1) and (5),
we get a + d = 3an - 2/b=> a + (-2/b) = 3a(1) - 2/b=> a - 3a + 2/b = -2/b=> -2a + 2/b = -2/b=> -2a = 0=> a = 0From equation (1), we have a = 3an=> 0 = 3(1/3)n=> n = 0
Therefore, from equation (5), we have:d = -2/b=> 0 = -2/b=> b = ∞Now, we know that (a+b)ab = (0+∞)(0*∞) = undefined
Therefore, the values of (a+b)ab are undefined.
For more questions on Values .
https://brainly.com/question/843074
#SPJ8
100 pts
Question 6
A grid shows the positions of a subway stop and your house. The subway stop is located at (-2,8)
and your house is located at (-8,0). What is the distance, to the nearest unit, between your house
and the subway stop?
O 15
010
07
0 20
Next >
< Previous
Answers
100PT! ABCD is a square. Given only the choices below, which properties would you use to prove AEB ≅ DEC by ASA? (You can choose more than one!)
Opposite angles are congruent.
The diagonals bisect each other.
All sides are congruent.
Opposite sides are parallel.

Answers
To prove AEB ≅ DEC by ASA (Angle-Side-Angle), the properties that can be used are:
1. Opposite angles are congruent: This property is necessary to establish that ∠AEB ≅ ∠DEC, as both angles are opposite each other in the square ABCD.2. All sides are congruent: This property is necessary to establish that AE ≅ DE, as both sides are corresponding sides of the square ABCD.So, the properties to use for proving AEB ≅ DEC by ASA are "Opposite angles are congruent" and "All sides are congruent."
\(\huge{\mathcal{\colorbox{black}{\textcolor{lime}{\textsf{I hope this helps !}}}}}\)
♥️ \(\large{\textcolor{red}{\underline{\texttt{SUMIT ROY (:}}}}\)
Answer:
To prove AEB ≅ DEC by ASA (Angle-Side-Angle), the properties that can be used are:
1. Opposite angles are congruent: This property is necessary to establish that ∠AEB ≅ ∠DEC, as both angles are opposite each other in the square ABCD.
2. All sides are congruent: This property is necessary to establish that AE ≅ DE, as both sides are corresponding sides of the square ABCD.
So, the properties to use for proving AEB ≅ DEC by ASA are "Opposite angles are congruent" and "All sides are congruent."
Step-by-step explanation:
Consider and angle with its vertex at the origin, one side on the x axis and one side passing through the point (x,y). The cosine of the angle is 21/29. Find the since and tangent of the angle.

Answers
Therefore , the solution of the given problem of angle comes out to be tangent is approximately 0.952 and its sine is roughly 0.729.
An angle meaning is what?An angle is a shape in Euclidean geometry made up of two angles, or circular faces, that divide at the centre of the wall and the wall's apex. At their intersections, two rays can join to form an angle. Angle is another outcome of two entities interacting. They are known as dihedral angles. A two-dimensional curve can be created by arranging two line beams in various ways at their ends.
Here,
We must ascertain the values of y and x in order to determine the sine and tangent of the angle. We are aware of:
=> √(x² + y²) * cos(θ) = x/h * 21/29
When we square both edges, we obtain:
=> x² / (x^2 + y²) = 441/841
The result of multiplying both parts by (x² + y²) is:
=> x²= (441/841) (x²+ y²)
If we simplify, we get:
=> (400/841) x² = (441/841) y²
The result of dividing both parts by (441/841) is:
=> (400/441) x²/ y² = 1
=> (y/x)² = 400/441
When we square the two edges, we obtain:
=> y/x = ±(20/21)
=> y/x = 20/21
Therefore,
sin(θ) = y/√(x² + y²) = (20/21) / √(1 + (20/21)²) ≈ 0.729
=> tan(θ) = y/x = 20/21 ≈ 0.952
As a result, the angle's tangent is approximately 0.952 and its sine is roughly 0.729.
To know more about angles visit:
https://brainly.com/question/14569348
#SPJ1
2. Use Laplace transform to solve the ODE f"(t) + 6ƒ' (t) + 13ƒ(t) = 48(t – 5) with initial conditions ƒ(0) = 0, ƒ'(0) = 0, where 8(t) is the Dirac delta function. [10]
Answers
The final solution in terms of the inverse Laplace transforms:
\(ƒ(t) = L^{-1}[F(s)]\)
We have,
To solve the given ordinary differential equation (ODE) using Laplace transform, we'll follow these steps:
Step 1: Take the Laplace transform of both sides of the equation.
Step 2: Solve for the Laplace transform of the function ƒ(t).
Step 3: Take the inverse Laplace transform to obtain the solution in the time domain.
Let's go through these steps:
Step 1: Taking the Laplace transform of the ODE, we have:
L[f"(t)] + 6L[f'(t)] + 13L[f(t)] = 48L[t - 5]
Step 2: Applying the Laplace transform properties and using the initial conditions, we get:
\(s^2F(s) - sf(0) - f'(0) + 6(sF(s) - f(0)) + 13F(s) = 48(e^{-5s}/s)\)
Simplifying, we have:
\(s^2F(s) + 6sF(s) + 13F(s) - s(0) - 0 + 6sF(s) - 6(0) + 13F(s) = 48(e^{-5s}/s)\)
Combining like terms, we obtain:
\((s^2 + 6s + 13)F(s) = 48(e^{-5s}/s)\)
Step 3: Solving for F(s), we have:
\(F(s) = 48(e^{-5s}/s) / (s^2 + 6s + 13)\)
To find the inverse Laplace transform of F(s), we can use partial fraction decomposition, but the expression involves complex roots.
Therefore,
The final solution in terms of the inverse Laplace transforms:
\(ƒ(t) = L^{-1}[F(s)]\)
Learn more about differential equations here:
https://brainly.com/question/32538700
#SPJ4
A line segment that goes from one side of the circle to the other side of the circle and doesn't go through the center is:
Answers
A line segment that goes from one side of the circle to the other side of the circle and doesn't go through the center is called chord of circle.
Since,
The chord of a circle can be defined as the line segment joining any two points on the circumference of the circle.
The diameter is the length of the line through the center that touches two points on the edge of the circle.
So, we can say that every diameter of a circle is always called chord (longest chord) but every chord of the circle is not a diameter because the diameter passes to the circle's center but it not necessay that evey chord will pass through the center of the circle. Some, line segment goes from one side of the circle to the other side and doesn't pass through centre then for this case the line segment is called chord of the circle.
Hence, a line segment that goes from one side of the circle to the other side of the circle and doesn't go through the center is called chord of the circle.
Find out more information about chord of circle here:
https://brainly.com/question/1654080
#SPJ4
6. Use the Distributive Property to write an
expression equivalent to 5 X (3 + 4)
Answers
Answer:
35
Step-by-step explanation:
5(3+4)
5x7=35
a(b + c) = ab + ac
(a + b)c = ac + bc
Therefore, the equivalent expression of 5 (3 + 4) is:
5 (3 + 4)= (5*3) + (5*4) = 35
Or
(3 + 4) 5 = (3*5) + (4*5) = 35
Hayden bought a necklace with a gemstone in the shape of a triangular prism. The base is an equilateral triangle measuring 2 centimeters on each side and has a base height of 1.7 centimeters. The height of the gemstone is 4 centimeters. Find the total surface area of the gemstone.
Answers
Answer:78
Step-by-step explanation:
Triangles P Q R and S T U are shown. Angles P R Q and T S U are right angles. The length of P Q is 20, the length of Q R is 16, and the length of P R is 12. The length of S T is 30, the length of T U is 34, and the length of S U is 16. Using the side lengths of △PQR and △STU, which angle has a sine ratio of Four-fifths?
Answers
Answer:
<p
Step-by-step explanation:
Answer: <p
Step-by-step explanation: I did the quiz
A cube is painted so that one side is blue, two sides are red, and three sides are green. How many different such cubes can be painted
Answers
There are 240 different ways to paint a cube such that one side is blue, two sides are red and three sides are green.
In the given problem, we can imagine the different possible configurations for painting different sides of a cube. We can consider the number of cubes that would be painted with one blue face, two red faces, and three green faces. In total, we could paint any of the six faces with blue, any of the remaining five faces with red, and any of the remaining four faces with green.
Therefore, the total number of different cubes that can be painted with one blue face, two red faces and three green faces is the product of the number of ways to choose one blue face from the six faces, times the number of ways to choose two red faces from the remaining five faces, times the number of ways to choose three green faces from the remaining four faces.
So, the number of possible cubes can be calculated as:
6C1 x 5C2 x 4C3 = 6 x 10 x 4 = 240
For such more questions on cube
https://brainly.com/question/1972490
#SPJ8
The Arizona Department Real Estate takes its rules and regulations from three basic sources: The Arizona State Constitution, Arizona Revised Statutes, and the Arizona ____________ Code.
Answers
The Arizona Department of Real Estate takes its rules and regulations from three basic sources: The Arizona State Constitution, Arizona Revised Statutes, and the Arizona Administrative Code.
The Arizona Department of Real Estate derives its rules and regulations from three primary sources. Firstly, the Arizona State Constitution serves as a fundamental legal document that outlines the framework and principles upon which the state operates.
Secondly, the Arizona Revised Statutes (ARS) provide the statutory laws enacted by the Arizona State Legislature. These statutes specifically address real estate matters and govern the activities and conduct of individuals and organizations involved in the real estate industry.
Lastly, the Arizona Administrative Code (AAC) contains the administrative rules and regulations established by various state agencies, including the Arizona Department of Real Estate. The AAC provides detailed guidelines and procedures for implementing and enforcing the laws set forth in the Arizona Revised Statutes, ensuring compliance and facilitating the proper functioning of the real estate sector within the state.
Learn more about Arizona Department of Real Estate here:
https://brainly.com/question/30404299
#SPJ11
according to a survey, 59% of parents say their teens are addicted to using their cell phones. write this percent as a fraction and do not simplify.
Answers
The answer in fractions is 59/100
When a statistic is given as a percentage, we assume that the sample size of the survey is
100
persons.
So, in the aforementioned question, we simply need to express the percentage as a fraction where the
numerator
represents the number of people who are in favor of the argument that their teens are addicted to cell phones which is
59
the denominator of the fraction represents the total number of people in the sample survey size which is
100.
simply using the above logic we can deduce the percentage into a fraction which is
59/100
.
to learn more about
Fractions
, use:
https://brainly.com/question/29271734
https://brainly.com/question/28985850
I really need help real fast please help with these three

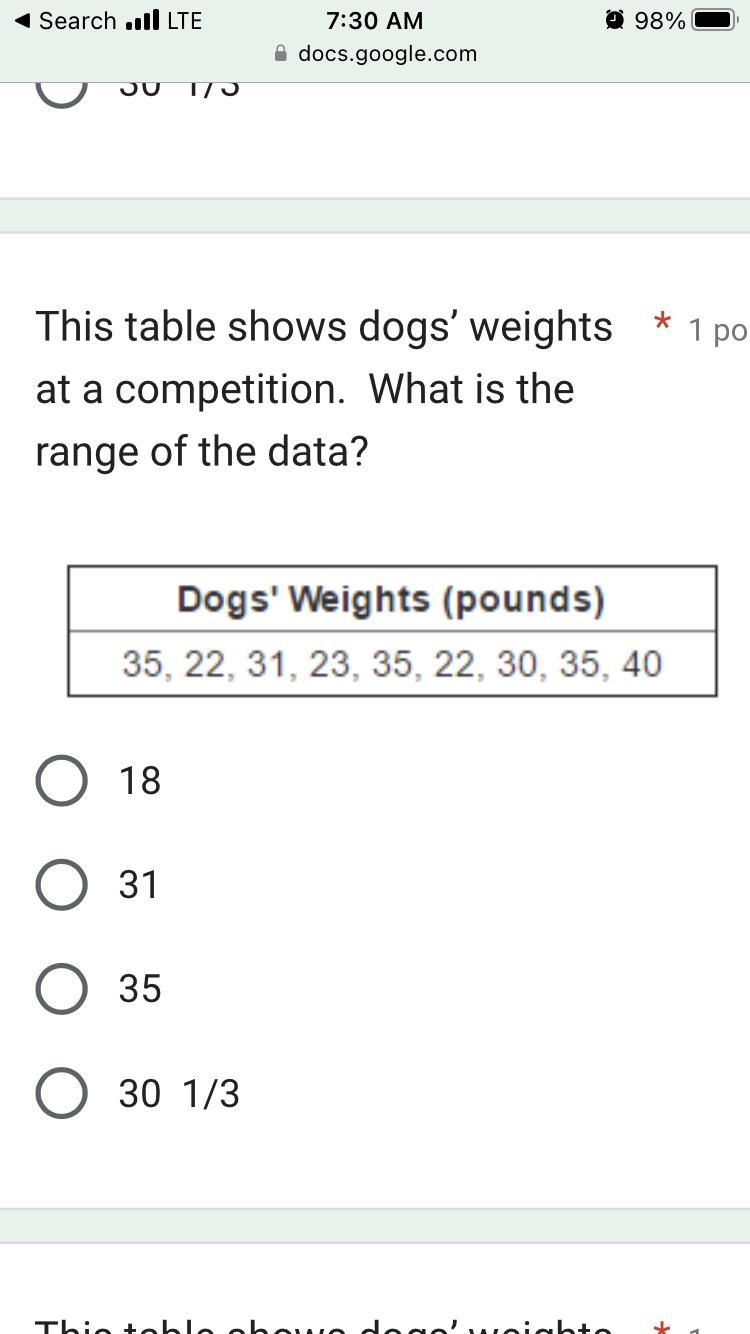

Answers
The range is 18 since it is the largest number minus the smallest number.
The mode of the data is 35 since it is the most common number.
solve for y 9=y/4-2
pleas help need to submit soon
Answers
Answer:
Y = 44
Step-by-step explanation:
44/4 - 2 = 9
11 - 2 = 9
Y = 44
<3
What is one ten to the zeroth power?
Answers
Answer:
1
Step-by-step explanation:
hope this helps!
1. A relation contains the ordered pairs shown. One of the ordered pairs is missing an x-coordinate. {(-1,4),(0,4),(2,5),(3,-6),(?,7)} What could be the missing x-coordinate if the relation is not a function?
Answers
Answer:
There are 4 possible scenarios where given relation could not be a function:
i) \((-1, 4), (0,4), (2,5), (3,-6), (-1, 7)\)
ii) \((-1, 4), (0,4), (2,5), (3,-6), (0, 7)\)
iii) \((-1, 4), (0,4), (2,5), (3,-6), (2, 7)\)
iv) \((-1, 4), (0,4), (2,5), (3,-6), (3, 7)\)
Step-by-step explanation:
From Function Theory, we remember that a relation is not a function when at least one element from domain (x-coordinate) is related to one or more elements from range (y-coordinate). Hence, there are four possibilities of making the relation not a function:
i) \((-1, 4), (0,4), (2,5), (3,-6), (-1, 7)\)
ii) \((-1, 4), (0,4), (2,5), (3,-6), (0, 7)\)
iii) \((-1, 4), (0,4), (2,5), (3,-6), (2, 7)\)
iv) \((-1, 4), (0,4), (2,5), (3,-6), (3, 7)\)
Can I Have The Answer? It’s a disgusting hard homework, and I would really appreciate it if you helped me.

Answers
Answer:
-2c + 8d - 6
-6 + 5d - 2c + 3d
Step-by-step explanation:
When multiplying terms in a bracket, you need to multiply the term outside the bracket to all the terms in the bracket.
-\(\frac{2}{5}\)(15-20d+15c) = (-\(\frac{2}{5}\) × 15) + (-\(\frac{2}{5}\) × -20d) + (-\(\frac{2}{5}\) × 15c)
Two minuses make a plus. One minus and one plus make a minus.
(-\(\frac{2}{5}\) × 15) + (-\(\frac{2}{5}\) × -20d) + (-\(\frac{2}{5}\) × 15c) = (-6) + (8d) + (-2c)
Note that the terms can be presented in any order and still be correct.
(-6) + (8d) + (-2c) = -6 + 8d - 2c
Let us go through the options to see which ones are correct:
-2c + 8d - 6: Since all the terms are there, this option is correct!
-c + 40d - 8: Since none of the terms is there, this option is wrong.
-4c + 40d - 8: Since none of the terms is there, this option is wrong.
-3c + 8d - 3: Since (-3c) and (-3) are not the correct terms, this option is wrong.
-6 + 5d - 2c + 3d: If we evaluate the like terms (5d and 3d), the final answer would be -6 + 8d - 2c so this option is also correct!
A certain box has a width that is 2 inches more than its length and a height that is 5 inches less than its length. If each of the two smallest faces of the box has an area of 36 square inches, what is the volume if the box?
Answers
Answer:
V = 396 cubic inches
Step-by-step explanation:
width = w; length = l; height = h; volume = V; area of smallest face = a
base units are inches
w = 2 + l
h = l - 5
Height is smallest and length is second smallest (h = l -, l = l, w = l +), so a is for h and l.
a = h × l = (l - 5) × l
36 = l^2 - 5l ==> l^2 - 5l - 36 = 0
Factor ==> (l - 9) × (l + 4) = 0
l = 9 and l = -4
Since length cannot be negative, 9 is the only Real answer.
l = 9
h = l - 5 = 9 - 5 = 4
w = 2 + l = 2 + 9 = 11
Volume of rectangular prism/box is length times width times height.
V = l × w × h = 9 × 11 × 4 = 396
The volume of the box with the given dimensions is;
Volume = 396 in³
Let us denote the properties of the box as follows;
Length of box = l
Width of box = w
Height of box = h
Area of the smallest face of box = a
We are told that the width is 2 inches more than the length. Thus;
w = 2 + l
We are told that the height is 5 inches less than its length. Thus;
h = l - 5
Since length is smaller than width but bigger than height, the height and length are the 2 smallest faces
Thus,
a = h × l
plugging in the relevant values gives;
a = (l - 5) × l
a = l² - 5l
We are told that the area of the two smallest faces is 36 in². Thus;
l² - 5l = 36
l² - 5l - 36 = 0
Using online quadratic equation solver, we have; l = 9 inches
Plugging in 9 for h in; h = l - 5
h = 9 - 5
h = 4
Also, plugging in 9 for l into; w = 2 + l, we have;
w = 2 + 9
w = 11
Volume of box is given by;
Volume = length × width × height
Volume = 9 × 11 × 4
Volume = 396 in³
Read more at;https://brainly.com/question/13973603
What’s the answer to this question?

Answers
what are the answer choices?
Sooo the answer is y=3x-7
Hope this helps.
A hybrid car averages 630 miles on a 122-gallon tank of gas. Ramona has enough money to put 7.4 gallons of gas in her hybrid. She is planning on driving her brother home from college for the weekend.
If their home is 320 miles away, should she be able to make it home before she runs out of gas?
The hybrid car will travel ___ miles on 7.4 gallons of gas, therefore, she (will,will not) be able to make it home.
(Round to the nearest whole number as needed.)

Answers
The hybrid car will travel 38 miles on 7.4 gallons of gas, therefore, she will not be able to make it home.
How to solve the problem?The problem is solved applying the proportions in it's context.
A hybrid car averages 630 miles on a 122-gallon tank of gas, hence the number of miles per gallon is given as follows:
630/122 = 5.16 miles per gallon
Ramona has enough money to put 7.4 gallons of gas in her hybrid, hence the distance that she can travel is obtained as follows:
7.4 x 5.16 = 38 miles.
Hence she will not be able to make it home.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1
Solve for f
-4 + 2 + 4f = 8 - 3f
f = ____
Answers
Step-by-step explanation:
-4 + 2 + 4f = 8 - 3f
-2+4f=8-3f
7f=10
f=10/7
Step-by-step explanation:
\( - 4 + 2 + 4f = 8 - 3f \\ - 2 + 4f = 8 - 3f \\ - 4f - 3f = - 2 - 8 \\ \frac{ - 7f }{ - 7} = \frac{ - 10}{ - 7} \\ f = \frac{10}{7} \)
use the intermediate value theorem to show that the polynomial has a real zero between the given integers? f(x)= x^3-x-4; between 1 and 7.
Answers
We have demonstrated using the Intermediate Value Theorem that the polynomial function has a real zero between 1 and 7.
To apply the Intermediate Value Theorem to the polynomial function f(x) = x^3 - x - 4 and show that it has a real zero between the integers 1 and 7, we need to verify that f(1) and f(7) have opposite signs.
Let's evaluate f(1) and f(7) to determine their signs:
f(1) = (1)^3 - (1) - 4 = 1 - 1 - 4 = -4
f(7) = (7)^3 - (7) - 4 = 343 - 7 - 4 = 332
From the calculations, we can see that f(1) = -4 and f(7) = 332.
Since f(1) is negative (-4) and f(7) is positive (332), they have opposite signs.
According to the Intermediate Value Theorem, if a continuous function changes sign between two points, then it must have at least one real zero between those points.
Since f(1) = -4 (negative) and f(7) = 332 (positive), the polynomial function f(x) = x^3 - x - 4 must have a real zero between the integers 1 and 7.
Therefore, we have demonstrated using the Intermediate Value Theorem that the polynomial function has a real zero between 1 and 7.
for such more question on Intermediate Value
https://brainly.com/question/24015908
#SPJ8
Solve:
4c + 9f = 120
Answers
Answer:
c=30 -9over 4 f f= 40 over 3 negative 4 over 9 c
Step-by-step explanation:
hopefully that helps you
Type the correct answer in the box. use numerals instead of words. what is the solution of this equation?
Answers
The resultant answer of the given equation [-1/2n² + 18 = 0] is n = 6.
What do we mean by equations?A mathematical statement known as an equation is made up of two expressions joined together by the equal sign. The definition of an equation in algebra is a mathematical statement that demonstrates the equality of two mathematical expressions. For instance, the equation 3x + 5 = 14 consists of the two equations 3x + 5 and 14, which are separated by the 'equal' sign.So, -1/2n² + 18 = 0:
Solve for 'n' as follows:
-1/2n² + 18 = 0-1/2n² = -18-n² = -36n = √36n = 6Therefore, the resultant answer of the given equation [-1/2n² + 18 = 0] is n = 6.
Know more about equations here:
https://brainly.com/question/28937794
#SPJ4
The complete question is given below:
Type the correct answer in the box. use numerals instead of words. what is the solution to this equation?

A person standing at the edge of a cliff 48 feet tall throws a ball up and just off the cliff with an initial upward velocity of 8 feet per second. What is the maximum height of the ball? When will the ball hit the ground?
The ball will be at its highest at ___
feet.
The ball will hit the ground at t =___
second(s)
Answers
Check the picture below.
since the cliff is 48 feet tall, thus that its initial height.
\(~~~~~~\textit{initial velocity in feet} \\\\ h(t) = -16t^2+v_ot+h_o \quad \begin{cases} v_o=\textit{initial velocity}&8\\ \qquad \textit{of the object}\\ h_o=\textit{initial height}&48\\ \qquad \textit{of the object}\\ h=\textit{object's height}&\\ \qquad \textit{at "t" seconds} \end{cases} \\\\\\ h(t)=-16t^2+8t+48\)
well, as you can see in the picture, its maximum is at its vertex, so
\(\textit{vertex of a vertical parabola, using coefficients} \\\\ y=\stackrel{\stackrel{a}{\downarrow }}{-16}x^2\stackrel{\stackrel{b}{\downarrow }}{+8}x\stackrel{\stackrel{c}{\downarrow }}{+48} \qquad \qquad \left(-\cfrac{ b}{2 a}~~~~ ,~~~~ c-\cfrac{ b^2}{4 a}\right) \\\\\\ \left(-\cfrac{ 8}{2(-16)}~~~~ ,~~~~ 48-\cfrac{ (8)^2}{4(-16)}\right)\implies \left(\cfrac{1}{4}~~,~~48-\cfrac{64}{-64} \right)\)
\(\left( \frac{1}{4}~~,~~48+1 \right)\implies \stackrel{\textit{the ball is at highest}}{\left(\frac{1}{4}~~,~~\stackrel{\downarrow }{49} \right)}\)
well, it hits the ground when y = 0.
\(\stackrel{h(t)}{0}=-16t^2+8t+48\implies 0=8(-2t^2+t+6)\implies 2t^2-t-6=0 \\\\\\ (2t+3)(t-2)=0\implies t= \begin{cases} -\frac{3}{2}\\\\ 2~~\textit{\large \checkmark} \end{cases}\)
notice, we didn't use the negative value, since "t" must be greater than 0.

a unit of measurement equal to one thousand meters is_____
Answers
A unit of measurement which is equal to one thousands meters is known as one kilometers.
Different units of measurements are meters , kilometers, centimeters, millimeters and so on.Measuring small length basically we use centimeters and very small length in millimeters.Measuring medium length we use unit known as meters.When we have measure long distance unit which is used is known as kilometers.Relation between different units of length are:1centimeters is equal to 10 millimeters1 meter is equal to 100 centimeters1 kilometers is equal to 1000 metersTherefore, the given units of measurement equal to one thousand meters is called one - kilometers.
Learn more about units here
brainly.com/question/10433377
#SPJ4
Verify that the intermediate value theorem applies to the indicated interval and find the value of c guaranteed by the theorem. f(x) = x2 4x 2, [0, 9], f(c) = 23 c =
Answers
The intermediate value theorem applies to the indicated interval and the importance of c guaranteed by the theorem is c=2,3.
Especially, he has been credited with proving the following five theorems: a circle is bisected via any diameter; the bottom angles of an isosceles triangle are the same; the other (“vertical”) angles are shaped by means of the intersection of two traces are same; two triangles are congruent (of identical form and size.
In mathematics, a theorem is an announcement that has been proved or may be proved. The evidence of a theorem is a logical argument that makes use of the inference guidelines of a deductive system to set up that the concept is a logical result of the axioms and formerly proved theorems.
In line with the Oxford dictionary, the definition of the concept is ''a rule or principle, especially in arithmetic, that may be proved to be true''. For example, in arithmetic, the Pythagorean theorem is a theorem and is maximum extensively used in the domain of science.
2-1and interval = [4]
since function text is continuous in a given interval. And also
+(4) = 42+4 = 4-1
20 = 6667
$(5/4) = ($145/2
stone-1
= 5.833
simple, f(4) > $(5/2), hence Intermediate
Theorem & applies to the indicated proved.
Now,
= 6 C-1
C-5c +6 = 0
C=2 or c=3
1=3 or
C= 2, 3
<= 2
Learn more about theorem here https://brainly.com/question/26594685
#SPJ4