An airplane leaves new york city and heads toward los angeles. as
it climbs, the plane gradually increases its speed until it reaches
cruising altitude, at which time it maintains a constant speed for
several hours as long as it stays at cruising altitude. after flying for
32 minutes, the plane reaches cruising altitude and has flown 192
miles. after flying for a total of 92 minutes, the plane has flown a
total of 762 miles. assuming that the plane maintains its speed at
cruising altitude, determine the total number of miles the plane has
flown 2 hours into the flight.

The linear transformations d and d2 are defined by taking the first derivative of a function in the space of smooth functions c[infinity](r). In other words, given a function f in c[infinity](r), d(f) is the function that represents the rate of change of f at each point in r, while d2(f) represents the rate of change of d(f).

To understand this concept better, consider an example of a function f(x) = x² in the interval r = [0, 1]. The derivative of f is f'(x) = 2x, which represents the slope of the tangent line to the curve of f at each point x in the interval. Thus, d(f)(x) = 2x. Similarly, the second derivative of f is f''(x) = 2, which represents the curvature of the curve of f at each point x in the interval. Thus, d2(f)(x) = 2.

These linear transformations are important in the study of differential equations and calculus. They allow us to represent the behavior of functions in terms of their rates of change, and to derive new functions from existing ones based on these rates of change. Additionally, these transformations have applications in physics, engineering, and other areas of science where the study of rates of change is essential.

To know more about linear transformations, refer to the link below:

https://brainly.com/question/13595405#

#SPJ11

The difference between the number of students who earned a score of 90 or greater in class 2 and those who earned a 90 or greater in class 1 is 1.

The test scores for the students in the two classes are summarized in these box plots. To find the difference between the number of students who earned a score of 90 or greater in class 2 and the number of students who earned a 90 or greater in class 1, we need to count the number of students that earned 90 or greater in each class and take the difference.

The answer to this question is the difference between the number of students who earned a score of 90 or greater in class 2 and those who earned a 90 or greater in class 1. We can get this by counting the number of students who score 90 or greater in each class and then taking the difference between the two. The box plot for class 1 shows that there is only one student who has a score of 90 or greater.

The box plot for class 2 shows that two students scored 90 or greater. Thus, the difference between the number of students who earned a score of 90 or greater in class 2 and those who earned a 90 or greater in class 1 is 2 - 1 = 1. Therefore, the correct option is A: 1.

To find the difference between the number of students who earned a score of 90 or greater in class 2 and the number of students who earned a 90 or greater in class 1, we need to count the number of students that earned 90 or greater in each class and take the difference. A box plot is a graphical dataset representing the median, quartiles, and extreme values. It is used to depict data distribution visually. In the question, two box plots represent the data of two different classes.

The box plot for class 1 shows that there is only one student who has a score of 90 or greater. The box plot for class 2 shows that two students scored 90 or greater. We can see that the box plot of class 1 is short and has only one whisker pointing up, indicating that there is only one student who scored higher than the median. The box plot of class 2, on the other hand, is longer and has two whiskers pointing up, indicating that two students scored higher than the median.

Therefore, the difference between the number of students who earned a score of 90 or greater in class 2 and those who earned a 90 or greater in class 1 is 1.

To know more about the median, visit:

brainly.com/question/300591

#SPJ11

Answer:

  arc BC = 26°

  arc AB = 90°

  arc CAB = 334°

Step-by-step explanation:

The angles of a linear pair total 180°. This fact lets us write an equation for x that helps us find the measures of all the angles and arcs. The arcs of a circle total 360°.

__

∠APE +∠EPD = 180°

  (25x +15)° +(33x -9)° = 180°

  58x = 174 . . . . . . divide by °, subtract 6

  x = 3 . . . . . . . . divide by 58

Angle APE is ...

  ∠APE = (25x +15)° = (25·3 +15)° = 90°

Then ...

  ∠EPD = 180° -90° = 90°

  ∠DPC = (20·3 +4)° = 64°

  ∠CPB = 90° -64° = 26°

  arc BC = ∠BPC = 26°

  arc AB = ∠APB = 180° -90° = 90°

  arc CAB = 360° -arc AB = 334°

The Intersecting Chords theorem states that the products of the line segments on each chord are equal. In other words,

We already know that UM = 26, UJ = 13, and UK = 39. Putting these values in the above equation gives us

Dividing both sides by 26 gives

which is our answer!

The point that belongs in the inverse variation along with (3, -4) is option A, (-2, 6).

To determine which point belongs in the inverse variation along with (3, -4), we can use the concept that in inverse variation, the product of the x-coordinate and the y-coordinate remains constant.

By multiplying the x-coordinate (3) and the y-coordinate (-4), we get -12.

Let's check the product for each option:

A. (-2, 6): -2 * 6 = -12 (matches the constant product)

B. (6, -8): 6 * -8 = -48 (does not match)

C. (3, 4): 3 * 4 = 12 (does not match)

D. (-4, -3): -4 * -3 = 12 (does not match)

Learn more about inverse variation on:

https://brainly.com/question/13998680

#SPJ1

Answer:

2 x-intercepts at 3 and 9

Step-by-step explanation:

x^2-12x+27=0

(factoring)

(x-3)(x-9)=0

this tells us that there is going to be a x-intercept at both 3, and 9, and that there is no stretching of it. It would look like this.

Answer:

x=1

Step-by-step explanation:

(5x-3)3=8

taking the cube of both sides we get

5x-3=2

adding three to both sides we get

5x=5

dividing by five on both sides we get

x=1

if you found my answer helpful please mark as brainliest.

Answer:

(-3, 19)

Step-by-step explanation:

In conclusion, none of the provided statements are correct as they do not hold true based on the rules and properties of exponentiation.

Let's examine each statement:

1. \(x^-a = x^a\): This statement is incorrect. The exponent of -a implies the reciprocal of x raised to the power of a, which is not equivalent to x raised to the power of a. Therefore, the statement is false.

2. \((x^a)(y^a) = (xy)^2^a\): This statement is incorrect. The product of \(x^a\)and \(y^a\) is not equal to the result of raising xy to the power of 2a. In general, the exponent rule for multiplying exponents would yield \((xy)^a\), not \((xy)^2^a\). Hence, the statement is false.

3. \(x^0\) = x: This statement is incorrect. Any non-zero number raised to the power of 0 is equal to 1, not x. Therefore, \(x^0\) is not equal to x. Thus, the statement is false.

4. \((1/x)^a\) = \(x^-^a\): This statement is incorrect. The reciprocal of x raised to the power of a is not equal to x raised to the power of -a. The negative exponent signifies the reciprocal of x raised to the power of a, but it does not change the sign of x itself. Thus, the statement is false.

To learn more about reciprocal click here: https://brainly.com/question/15590281

#SPJ11

Answer:

Absolute Value

Step-by-step explanation:

– The distance a number is from the zero on a number line. (Absolute value is always positive). Acute Angle - An angle with a measure > than 0° and < 90˚. Acute Triangle– A triangle that has all three angles that are acute.

1. The equation y + 3/4(y+30) = 478 can be used to find y, Elena's score in her first game. False

2. The difference between the score in Elena's first game and her second game is 34. True

3. The equation z/4y + 30 = 478 can be used to find y, Elena's score in her first game. False

4.  Elena's scores 222 in her first game

To find Elena's scores in the game, we used the equation:

x = 3/4y + 30 since x + y = 478

3/4y + 30 + y = 478

7/4y + 30 = 478

7/4y = 478 - 30 = 448

448 x 4 /7 = 256

It means that Elena's scored 256 in her first game and 222 in her second game.

The above answer is based on the questions below as seen in the picture

Elena bowls two games on Saturday. Her serve in the second game is 30 more than 3/4 of her score in the first game. Elena's total score for the two games is 478.

Determine with each statement about Elena's bowling games is true;

1 The equation y + 3/4(y+30) = 478 can be used to find y, Elena's score in her first game.

2. The difference between the score in Elena's first game and her second game is 34.

3. The equation z/4y + 30 = 478 can be used to find y, Elena's score in her first game.

4. Elena's scores 222 in her first game.

Find more exercises on  equation;

https://brainly.com/question/1529522

#SPJ1

Answer:x should equal 7/13

Step-by-step explanation: it has been solved by a pro

Answer: slope = 6

Step-by-step explanation:

Slope is rise over run.

Find two perfect points and see how many up/down you go and how many left/right you go.

On this one, you go up 6, right 1.

6/1 is 6.

Hope this helps!

Answer:

6

Step-by-step explanation:

m=y2-y1/x2-x1

take two points from the graph (0, -1) and (1, 5)

5-(-1)=6

1-0=1

6/1=6

hope this helps :3

The equation p=4+3y is maximised at (4,5) and the maximised value is 31.

Given equation p=4x+3y and constraints x>0,x<4, y<5.

We have to maximise the equation p=4x+3y with constraints x>0,x<4,y<5.

To find out the points we have to make graphs of the constraints first.

From the graph we have found that points are (0,0),(0,5),(4,0),(4,5).

The above points are common points of graphs of all constraints.

The value of equations are as under:

At (0,0) , p=4*0+3*0=0

At (0,5), p=4*0+3*5=15

At (4,0),p=4*4+3*0=16

At (4,5),p=4*4+3*5=16+15=31

Maximum value of p is 31 and that is for (4,5).

Hence the equation is maximised at (4,5) and the maximum value is 31.

Learn more about equation at https://brainly.com/question/2972832

#SPJ4

Answer:

y=1/6x+4

Step-by-step explanation:

m=(y2-y1)/(x2-x1)

m=(4-3)/(0-(-6))

m=1/(0+6)

m=1/6

y-y1=m(x-x1)

y-3=1/6(x-(-6)(

y-3=1/6(x+6)

y=1/6x+6/6+3

y=1/6x+1+3

y=1/6x+4

Answer:

Step-by-step explanation:

Subtract the discount from 100 to get the percentage of the original price.

Multiply the final price by 100.

Divide by the percentage in Step One

Answer:

1.7 or square root of 2.9

Step-by-step explanation:

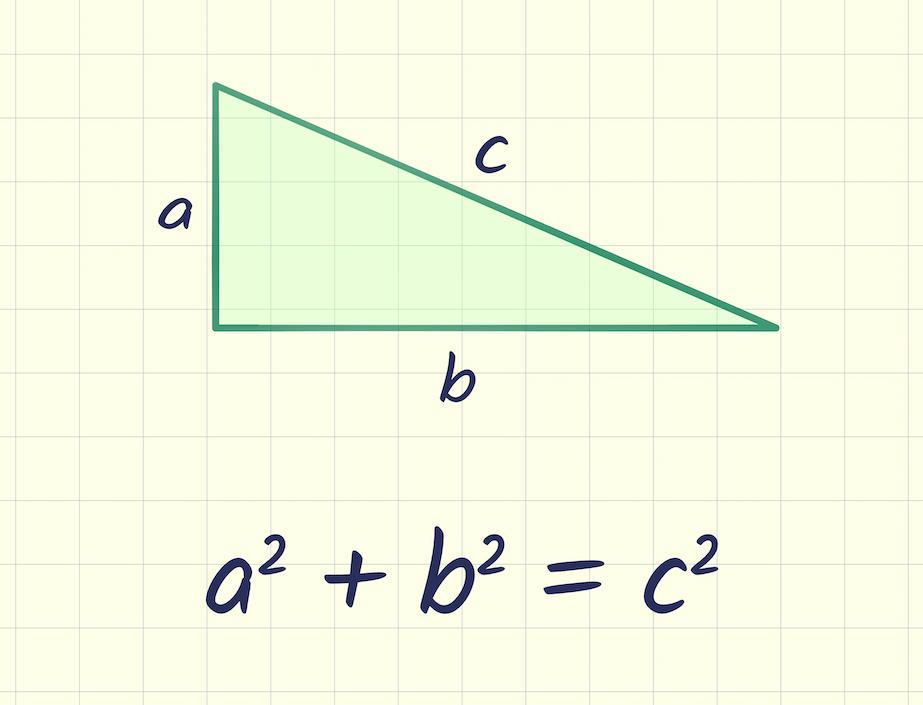
i remember how to do this its kind of simple really you just use the pythagorean theorem

Answer: 12

Step-by-step explanation:

Answer:

\(f'(x)= \frac{13}{(2x+3)^2}\\\)

Step-by-step explanation:

\(f(x)= \frac{3x-2}{2x+3} \\\)

\(f'(x)=\frac{dy}{dx} = \frac{d}{dx}(\frac{3x-2}{2x+3})\\ f'(x)= \frac{(2x+3)\frac{d}{dx}(3x-2)-(3x-2)\frac{d}{dx}(2x+3) }{(2x+3)^{2} } \\f'(x)= \frac{(2x+3)(3)-(3x-2)(2)}{(2x+3)^{2} } \\\)

\(f'(x)= \frac{6x+9-6x+4}{(2x+3)^{2} }\\ f'(x)= \frac{13}{(2x+3)^2}\\\)

Domains of function:

1) Interval Notation : (−∞,∞)

2) Interval Notation:(−∞,∞)

3) Interval Notation:(−∞,0)∪(0,∞)

4) Interval Notation:(−∞,∞)

5) Interval Notation:(−∞,∞)

1)

The domain of the expression is all real numbers except where the expression is undefined. In this case, there is no real number that makes the expression undefined.

Interval Notation:(−∞,∞)

Set-Builder Notation:{x|x∈R}

2)

The domain of the expression is all real numbers except where the expression is undefined. In this case, there is no real number that makes the expression undefined.

Interval Notation:(−∞,∞)

Set-Builder Notation:{x|x∈R}

3)

The domain by finding where the expression is defined.

Interval Notation:(−∞,0)∪(0,∞)

Set-Builder Notation:{x|x≠0}

4)

The domain by finding where the expression is defined.

Interval Notation:[−5,∞)

Set-Builder Notation:{x | x≥−5}

4)

The domain of the expression is all real numbers except where the expression is undefined. In this case, there is no real number that makes the expression undefined.

Interval Notation:(−∞,∞)

Set-Builder Notation:{x| x∈R}

Know more about domain,

https://brainly.com/question/29056382

#SPJ4

The slope of the tangent line to `f(x) = 2x + 1` at `x = 2` is `2`.

The given function is `f(x) = 2x + 1`.To find the slope of the tangent line at `x = 2`, we need to take the derivative of the function `f(x)` and then substitute `x = 2` into the derivative.Let's first take the derivative of `f(x)` with respect to `x`.

Using the power rule, we have: `f'(x) = 2`.

This means that the slope of the tangent line to `f(x)` is always `2` no matter what value of `x` we plug in.

However, we are interested in the slope of the tangent line at `x = 2`.

So, we substitute `x = 2` into the derivative to get the slope of the tangent line at `x = 2`.

Hence, the slope of the tangent line to `f(x) = 2x + 1` at `x = 2` is `2`.  

This is a answer since the question only requires a simple calculation.

To know more about tangent line visit:
brainly.com/question/31528951

#SPJ11

Let x be an even integer then x+2 is the next consecutive even integer.

We need to look at \((x+2)^2 - x^2\), since that's the difference between the squares of any two consecutive even integers.

\((x+2)^2 - x^2 = x^2 + 4x + 4 - x^2\)

                     \(=4x+4\)

                     \(=4(x+1)\)

Since that difference is 4 times something, it is a multiple of 4.

Answer:

1

Step-by-step explanation:

I used DESMOS and to find this answer

Hope this Helped

We can Long Division method to solve quotients step by step

What is long division method and its steps ?

When splitting huge numbers, the task is divided into several sequential parts using the long division approach. The dividend is divided by the divisor, just as in conventional division problems, and the result is known as the quotient; occasionally, it also produces a remainder.

We need to comprehend a few stages in order to divide. A vinculum or right parenthesis separates the dividend from the quotient, while a vertical bar separates the divisor from the dividend. Let's now go through the long division stages listed below to comprehend the procedure.

1. Take the dividend's first digit starting from the left . Verify if this digit exceeds or is equal to the divisor.

2. Next, divide it by the divisor, and write the result as the quotient on top.

3. Subtract the outcome from the digit, and then put the difference below.

Step 4: Decrease the dividend's subsequent digit (if present).

Step 5: Carry out Step 4 again.

Learn more about Long division from the link below

https://brainly.com/question/28824872

#SPJ4

Answer: its not 22 its 25

Step-by-step explanation: if it not correcnt than sorry wrong answer

Answer:

Range: 15

Step-by-step explanation:

This is a box and whisker plot, right? The first number is 17, then, and the last is 32. To find the range, we subtract 17 from 32. This gets us 15. The range of the data is 15. Also on this chart, it says that the median is 22, the upper quartile is 27, and the lower quartile is 20. I hope this helps.

To calculate John's BMI, we need to use the formula BMI = weight (kg) / height (m)^2. When we calculate this, we get a BMI of 36.1.

First, we need to convert John's height and weight to the metric system. His height is 1.75 meters and his weight is 110.5 kilograms.
Next, we can plug those values into the formula: BMI = 110.5 / (1.75)^2.
According to the Centers for Disease Control and Prevention, a BMI of 30 or above is considered obese. Therefore, John falls into the obese category based on his BMI.
It's important to note that BMI is just one measure of health and does not take into account muscle mass or other factors that can affect weight. It's always best to speak with a healthcare professional to determine a healthy weight and lifestyle plan.

For more questions on BMI

https://brainly.com/question/2064513

#SP11

Answer:

8 tickets

Step-by-step explanation:

x = 10 in the given equation.

We have then:

C (x) = 17.5x - 10

C (10) = 17.5 * (10) - 10

C (10) = 165

Answer:

to buy 10 tickets cost:

$ 165

b. How many tickets can you buy with $ 130?  

For this case we must make the substitution: C (x) = 130

We have then:

C (x) = 17.5x - 10

130 = 17.5x - 10

Clearing x we have:

17.5x = 130 + 10

17.5x = 140

x = (140) / (17.5)

x = 8

Answer:

You can buy 8 tickets with $ 130