Given:
P: 3x + 7 = -5
Q: 4x + 12 = -4
Answers
Answer:
A(8)-F5+6
Step-by-step explanation:
Answer:
P: x= -4
Q: x= -4
Step-by-step explanation:
Related Questions
Please need it in 5 mins

Answers
Answer:
70,110,110,110
Step-by-step explanation:
I'm Asian and I was less than 5 minutes
Can anyone help I would really appreciate it I’m failing everything and need a boost

Answers
Answer:
2,870 and 30
Step-by-step explanation:
. Suppose the weight of Chipotle burritos follows a normal distribution with mean of 450 grams, and variance of 100 grams2 . Define a random variable to be the weight of a randomly chosen burrito. (a) What is the probability that a Chipotle burrito weighs less than 445 grams? (3 points) (b) 20% of Chipotle burritos weigh more than what weig
Answers
Complete Question
Suppose the weight of Chipotle burritos follows a normal distribution with mean of 450 grams, and variance of 100 grams2 . Define a random variable to be the weight of a randomly chosen burrito.
(a) What is the probability that a Chipotle burrito weighs less than 445 grams? (3 points)
(b) 20% of Chipotle burritos weigh more than what weight
Answer:
a
\(P(X < 445 )= 0.3085\)
b
\(k = 458.42\)
Step-by-step explanation:
From question we are told that
The population mean is \(\mu = 450 \ g\)
The variance is \(var = 100 \ g^2\)
The consider weight is \(x = 445 \ g\)
The standard deviation is mathematically represented as
\(\sigma = \sqrt{var}\)
substituting values
\(\sigma = \sqrt{ 100}\)
\(\sigma = 10\)
Given that weight of Chipotle burritos follows a normal distribution the the probability that a Chipotle burrito weighs less than x grams is mathematically represented as
\(P(X < x ) = P ( \frac{X - \mu }{\sigma } < \frac{x - \mu }{\sigma } )\)
Where \(\frac{X - \mu }{\sigma }\) is equal to z (the standardized values of the random number X )
So
\(P(X < x ) = P (Z < \frac{x - \mu }{\sigma } )\)
substituting values
\(P(X < 445 ) = P (Z < \frac{445 - 450 }{10} )\)
\(P(X < 445 ) = P (Z <-0.5 )\)
Now from the normal distribution table the value for \(P (Z <-0.5 )\) is
\(P(X < 445 ) = P (Z <-0.5 ) = 0.3085\)
=> \(P(X < 445 )= 0.3085\)
Let the probability of the Chipotle burritos weighting more that k be 20% so
\(P(X > k ) = P ( \frac{X - \mu }{\sigma } > \frac{k - \mu }{\sigma } ) = 0.2\)
=> \(P (Z> \frac{k - \mu }{\sigma } ) = 0.2\)
=> \(P (Z> \frac{k - 450}{10 } ) = 0.2\)
From the normal distribution table the value of z for \(P (Z> \frac{k - \mu }{\sigma } ) = 0.2\) is
\(z = 0.8416\)
=> \(\frac{k - 450}{10 } = 0.8416\)
=> \(k = 458.42\)
PLEASE HELP AS SOON AS POSSIBLE

Answers
A) The Slope is: 2
B) The interpretation of the slope is that there are two races for each number of track meet.
C) The y-intercept from the graph is at: y = 4
D) Equation of the line is: y = 2x + 4
How to find the slope of the linear graph?The general form of equation of a line in slope intercept form is:
y = mx + c
where:
m is slope
c is y-intercept
From the given graph, we can see that the slope is gotten from the formula:
A) Slope = (y₂ - y₁)/(x₂ - x₁)
Thus:
Slope = (10 - 4)/(3 - 0)
Slope = 6/3
Slope = 2
B) The interpretation of the slope is that there are two races for each number of track meet.
C) The y-intercept from the graph is at: y = 4
D) Equation of the line is:
y = 2x + 4
Read more about Slope at: https://brainly.com/question/3493733
#SPJ1
Brainlyest to first correct. Report to incorrect
Add.
(−5x^4+6x^3−43)+(6x^5−x^2+12x+12)
Express the answer in standard form.
Enter your answer in the box.
Answers
Answer:
6x^5-5x^4+6x^3-x^2+12x-31
Step-by-step explanation:
(−5x^4+6x^3−43)+(6x^5−x^2+12x+12)
To add, combine like terms
6x^5-5x^4+6x^3-x^2+12x-43+12
6x^5-5x^4+6x^3-x^2+12x-31
Standard form means from the highest power of x to the lowest power of x
64 players started how many left after 3 rounds
Answers
Answer: 8
Step-by-step explanation:
I do not understand the question. But if my interpretation is correct, then:
If there are 64 players and 3 rounds, 32 will get out in the first round. 16 will get out in the second, and 8 will get out on the third. Thus, the answer is 64-(32+16+8) or just simply 8.
Simplifica combinando términos semejantes. 4x²-9xy-4y²-6x² - xy + 6y²
2 4x² − 9xy − 4y² − 6x² - xy + 6y² = ___ -
(Simplifica tu respuesta. No descompongas en factores). ?
Answers
Answer:
-2x² - 10xy + 2y²
Step-by-step explanation:
4x² - 9xy - 4y² - 6x² - xy + 6y² =
= 4x² - 6x² - 9xy - xy - 4y² + 6y²
= -2x² - 10xy + 2y²
Pls help me this is for my final text and I’m struggling really bad!!!

Answers
I say this because the line on your graph is a y=mx+b equation.
For every unit it moves over on the X axis it moves the same unit on the Y axis.
I hope this helps.
Please help answer this

Answers
The specified scale factor of the dilation from S to M is 3/2 therefore, the scale factor of the dilation transformation from M to S is 2/3
What is a dilation transformation?A dilation is a transformation in which the lengths of the sides of the image are increased or decreased in the proportion, specified by a scale factor to the dimensions of the pre-image.
The specified scale factor from triangle S to triangle M = 3/2
3/2 = 1.5
Therefore, the length of the sides of triangle M are 1.5 times the lengths of the sides of triangle S
Let L represent the length of a side of triangle S, we get;
The length of the corresponding side on triangle M = 1.5 × L
The scale factor from triangle M to triangle S is found by taking the ratio of the corresponding sides of triangle S and M as follows;
Scale factor, SF, from M to S = Length of a side on triangle S ÷ Length of the corresponding side on triangle
Therefore;
\(SF = \dfrac{L}{1.5\cdot L} = \dfrac{1}{1.5}\)
1.5 = 3/2, therefore;
\(SF = \dfrac{1}{1.5} = \dfrac{1}{\frac{3}{2} } =\dfrac{2}{3}\)
Therefore, the scale factor from M to S is 2/3
Learn more about scale factor of a dilation here:
https://brainly.com/question/13005407
#SPJ1
Do you think living in Delaware is expensive? ( you have to live in Delaware to answer please)
Answers
Answer:
it is as expensive as the other states in the us
Step-by-step explanation:
I am not from delaware but i know this
Niko has 3 friends he gives his friends 1/4 pieces of candy each. How much does each person get?
Answers
Answer:
12
Step-by-step explanation:
Please help! will make brainliest

Answers
Answer:
59,582π/375 (approximately 499.153) square centimeters
Step-by-step explanation:
Volume:
\( \frac{1}{3} \pi {(6.2)}^{2} (12.4)\)
\( \frac{1}{3} {( \frac{31}{5}) }^{2} ( \frac{62}{5} )\pi\)
\( \frac{59582\pi}{375} \)
What is the sum?
46 mins left please help!!!!!

Answers
need help with geometry problem number 12 ( ignore my writing )

Answers
Given
Height of man = 6ft
Shadow of man = 9ft
Shadsw of building = 322.5ft
Find
Height of building
Explanation
At a particular time in the day the proportion of the shadow of man will be equal to that of the building
So triangle ABC will be similar to PQR
Hence the ratio of their corresponding sides will be equal
\(\begin{gathered} \frac{AB}{BC}=\frac{PQ}{QR} \\ \frac{6}{9}=\frac{PQ}{322.5} \\ PQ=322.5\times\frac{2}{3} \end{gathered}\)Therefore,
PQ = 215ft
which is the required length
Final Answer
The height of the building is 215ft

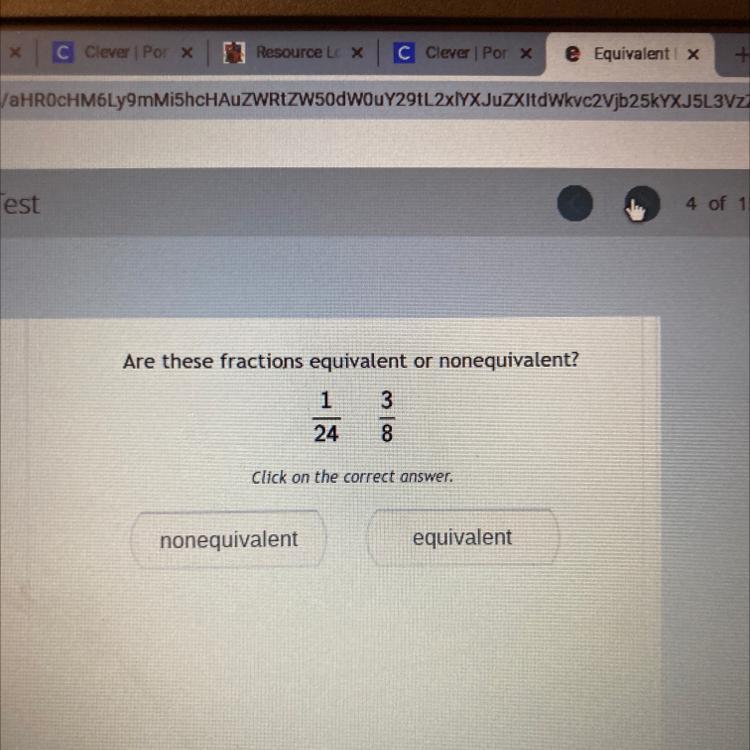
Are these fractions equivalent or nonequivalent?
1 3
24 8
Click on the correct answer.
nonequivalent
equivalent
Answers
Answer: Easy, hope this helps!
Nonequivalent
Step-by-step explanation:
1/24 = 1/24
3/8 = 9/24
1/24 and 9/24 is nonequivalent
Nevia spent 0.75 on 1.5 pounds of bananas. At, this rate, how much will 6 pounds of bananas cost?
Answers
Answer:
3.00
Step-by-step explanation:
6.0 / 1.5 = 4
0.75 * 4 = 3.00
i need the answer ASAP please help

Answers
The width of the garden with an area of 70 square feet is 10 feet
What is an equation?An equation consists of numbers and variables linked together by mathematical operations to form an expression.
The area of a field the amount of space occupied by the field. The area is the product of its length and width. It is given by:
Area = length * width
Let w represent the width. The length is 3 feet longer, hence:
Length = w + 3
Area = length * width
Area = (w + 3)(w)
But the area is 70 ft², hence:
70 = (w + 3)(w)
w² + 3w = 70
w² + 3w - 70 = 0
w = 7 feet
Length = w + 3 = 7 + 3 = 10 feet
The width is 10 feet
Find out more on equation at: https://brainly.com/question/2972832
#SPJ1
Expand and simplify x(3x + 5)-5(2x - 1)
PLEASE HELP ME AND ANSWER THIS QUESTION !! I need this for my assessment revision

Answers
The simplified expression of x(3x + 5)-5(2x - 1) is 3x² - 5x + 5
How to expand and simplify the expressionFrom the question, we have the following parameters that can be used in our computation:
x(3x + 5)-5(2x - 1)
Open the brackets
So, we have
3x² + 5x - 10x + 5
Evaluate the like terms
So, we have
3x² - 5x + 5
Hence, the simplified expression is 3x² - 5x + 5
Read more about expression at
https://brainly.com/question/31819389
#SPJ1
* 9. Two fifths of what number is 120?
2/5
Answers
Answer:
300
Step-by-step explanation:
120 ÷ 2/5 = 300
Let X represent the number we are looking for
2/5 x X=120
2/5X=120
Divide both sides by 2/5
X= 300
L
if m<xyz = 58 and m<wxz = 51 find m<wzx
Answers
Answer:
m<wzx = 71
Step-by-step explanation:
Assuming these are interior angles of a triangle.
The sum of all three interior angles of a triangle is always 180 degrees, therefore:
m<xyz + m<wxz + m<wzx = 180
Substitute our values:
58 + 51 + m<wzx = 180
m<wzx = 180 - 58 - 51
m<wzx = 71
A - on the po boyds at a emase the foot, 1, of building. He. Observes an obje- et on the top, P of the building at an angle of ele- building of 66 Aviation of 66 Hemows directly backwards to new point C and observes the same object at an angle of elevation of 53° · 1P) |MT|= 50m point m Iame horizontal level I, a a
Answers
Answer:
53\(x_{123}\) == 134 cf
Step-by-step explanation:
A - on the po boyds at a emase the foot, 1, of building. He. Observes an obje- et on the top, P of the building at an angle of ele- building of 66 Aviation of 66 Hemows directly backwards to new point C and observes the same object at an angle of elevation of 53° · 1P) |MT|= 50m point m Iame horizontal level I, a a
The height of the building is approximately 78.63 meters.
The following is a step-by-step explanation of how to solve the problem. We'll need to use some trigonometric concepts and formulas to find the solution.
Draw a diagram of the situation described in the problem to get a better understanding of the problem. The diagram would have a right-angled triangle with angle of elevation of 66° at the bottom left vertex and another angle of elevation of 53° at the bottom right vertex. The object on top of the building is at the vertex of the triangle. Point M and I on the diagram are points on the horizontal line of sight and on the ground respectively. We can label the diagram with the following values:Angle of elevation from point A = 66°Angle of elevation from point P = 53° Length of line segment AM = h Length of line segment MP = x Length of line segment IP = y Length of line segment MT = 50m. We'll use these values to calculate the length of h, which is the height of the building.Use the tangent ratio to find x:tan 66° = h / x => x = h / tan 66°. Use the tangent ratio to find y:tan 53° = h / y => y = h / tan 53°.We know that x + y = 50, so substituting the expressions for x and y from step 3 gives:h / tan 66° + h / tan 53° = 50h = 50 tan 66° tan 53° / (tan 53° + tan 66°) ≈ 78.63 m.Therefore, the height of the building is approximately 78.63 meters.
For more such questions on height, click on:
https://brainly.com/question/28122539
#SPJ8
Katy swim team is having an end-of-season pizza party when the party started doing 50 slices of pizza now there's 20 sizes love which of the following shows how much pizza has been eaten select all that apply
Answers
Answer:
B
Step-by-step explanation:
20/50
Work for E and F- 20/50=20X2=40 40 divided by 100 is 40%
50X2=100 and 40/100
Answer:
A, C, and D.
Step-by-step explanation:
-0.6
-60/100
-60%
1. 50-20=30
2. There are 30/50 slices left.
3. 30/50 = 60/100
4. 60/100 written as a decimal is 0.6.
Find the solution to the system of equations.
3x + y + z = 6
3x - y + 2z=9
y+ Z=3
O A. x = 2, y=-1, z = 5
O B. x = 1, y = 0, z = 3
O C. x = 5, y = -2, z = 6
O D. x = 2, y = 1, z = 4
Answers
Answer:
B. x = 1, y = 0, z = 3
Step-by-step explanation:
Systems of linear equations are readily solved by a suitable calculator or spreadsheet. The attached calculator display shows the solution to be ...
x = 1, y = 0, z = 3
__
There are several ways a calculator can solve these equations. The method shown here is to input the equations as an augmented matrix of their coefficients, and reduce it to row-echelon form. The right-most column of the reduced matrix is the set of variable values in the same order as the coefficients.
A graphing calculator can also be used to graph the equations. You may have to write one variable in terms of the others. (Here, it is convenient to let z=3-y.) The second attachment shows the solution (x, y) = (1, 0). This is sufficient to choose the correct answer. Or, you can finish the solution by substituting into the expression for z: z = 3 -y = 3 -0 = 3.
Many calculators will solve the system of equations after you enter it as presented in the problem statement.


The ratio of boys to girls on Rowan’s swim team is 2:3. If there are 24 boys on the team, how many girls are there?
Answers
Answer:
36 girls
Step-by-step explanation:
2x=24
x=12
3(12) = y
y= 36
Riley put £450 into a savings account which
gathered simple interest at a rate of 2% per month.
After 6 months, Riley used some of the money in
the account to buy a bike costing £480.
How much money did Riley have left?
Answers
Answer:
£26.77
Step-by-step explanation:
450(100% + 2%)^6
= 450 (1.02)^6
= 506.77.
506.77 - 480 - 26.77.
Riley had £26.77 left.
Identify the correct trigonometry formula to use to solve for x.11Х55°

Answers
From the right-angled triangle given, let us identify the given sides with reference to the angle 55°
\(\begin{gathered} x\text{ is the hypotenus(the longest side opposite the right angle)} \\ 11istheadjacentsidetotheangle55^0 \end{gathered}\)The correct trigonometry that has both the adjacent and the hypotenuse is the cosine
To solve for x, we will then use cosine as follows
\(\begin{gathered} \cos A=\frac{Adjacent}{\text{Hypotenus}} \\ \cos 55^0=\frac{11}{x} \end{gathered}\)\(undefined\)Urn 1 contains 4 blue tokens and 9 red tokens; urn 2 contains 12 blue tokens and 5 red tokens. You flip a coin twice and if you see head two times, then you pick urn 2 else (if you see at least once the tail) you pick urn 1 and draw out a token at random from that urn. Given that the token is blue, what is the probability that the token came from urn 2
Answers
Answer:
0.433
Step-by-step explanation:
From the given information;
Let represent Urn 1 to be Q₁ ;
Urn 2 to be Q₂
and the event that a blue token is taken should be R
SO,
Given that:
Urn 1 comprises of 4 blue token and 9 red tokens,
Then, the probability of having a blue token | urn 1 picked is:
\(P(R|Q_1) = \dfrac{4}{4+9}\)
\(= \dfrac{4}{13}\)
Urn 2 comprises of 12 blue token and 5 red tokens;
Thus \(P(R| Q_2) = \dfrac{12}{12+5}\)
\(=\dfrac{12}{17}\)
SO, if two coins are flipped, the probability of having two heads = \(\dfrac{1}{4}\)
(since (H,H) is the only way)
Also, the probability of having at least one single tail = \(\dfrac{3}{4}\)
(since (H,T), (T,H), (T,T) are the only possible outcome)
Thus: so far we knew:
\(P(Q_2) = \dfrac{1}{4} \\ \\ P(Q_2) = \dfrac{3}{4}\)
We can now apply Naive-Bayes Theorem;
So, the probability P(of the token from Urn 2| the token is blue) = \(P(Q_2|R)\)
\(P(Q_2|R) = \dfrac{P(R \cap Q_2)}{P(R)} \\ \\ = \dfrac{P(R|Q_2) * P(Q_2)}{P(R|Q_2) \ P(R_2) + P(R|Q_1) \ P(Q_1)} \\ \\ \\ \\ = \dfrac{\dfrac{12}{17} \times \dfrac{1}{4} }{\dfrac{12}{17} \times \dfrac{1}{4} + \dfrac{4}{13} \times \dfrac{3}{4}} \\ \\ \\ = \dfrac{13}{30}\)
= 0.433
The initial size of a culture of bacteria 1100. After 1 hour, the bacteria count is 9500.
Find the function n(t)=no
that models the population after
hours.
Find the population after 1.5 hours.
Then Find the number of hours when the number of bacteria will reach 20,000.
Sketch the graph of the population function.
Answers
To find the function n(t) that models the population of bacteria after t hours, we can use the exponential growth formula:
n(t) = n0 * e^(kt)
Where:
n(t) is the population after t hours,
n0 is the initial population size,
e is the base of the natural logarithm (approximately 2.71828),
k is the growth rate constant.
We can determine the value of k using the given information. After 1 hour, the bacteria count is 9500, which is the population at time t = 1. Plugging these values into the equation:
\(9500 = 1100 * e^(k*1)\)
To find k, we can divide both sides by 1100:
9500/1100 = e^k
Now, we can solve for k by taking the natural logarithm (ln) of both sides:
ln(9500/1100) = ln(e^k)
ln(9500/1100) = k
Now we have the value of k. We can plug it back into the exponential growth formula to obtain the function n(t):
\(n(t) = 1100 * e^(ln(9500/1100) * t)\)
To find the population after 1.5 hours, we can substitute t = 1.5 into the equation:
\(n(1.5) = 1100 * e^(ln(9500/1100) * 1.5)\)
To find the number of hours when the number of bacteria will reach 20,000, we can set n(t) equal to 20,000 and solve for t:
\(20,000 = 1100 * e^(ln(9500/1100) * t)\)
Finally, to sketch the graph of the population function, plot the values of t on the x-axis and the corresponding values of n(t) on the y-axis using the equation n(t) = 1100 * e^(ln(9500/1100) * t). The resulting graph will show the exponential growth of the bacteria population over time.
For more such questions on exponential
https://brainly.com/question/30166689
#SPJ8
Find the volume of the prism.
8 cm
12 cm
6 cm

Answers
Area of base triangle = 1/2×base×height
Area of base= 8×6×1/2= 24cm²
Area of prism = Bh = 24 × 12 = 288cm³
Answer:
Solution given:
base[b]=6cm
height [h]=8cm
length[l]=12cm
volume of a prism=area of triangle *length
=½*b*h*l=½*6*8*12=288cm³
is a required answer.
How to get a tutor please I don’t know
Answers
Answer:
You could ask a school principal and he/she will tell you most likely.
Umm, You could visit websites like Club.Z?
Brainliest?
thx U3U <3