jamie used a microscope to measure the diameter of a hair. she found that the diameter of the hair was 0.000072 meter. how is this number written in scientific notation
Answers
Answer:
7.2x10^-5
Step-by-step explanation:
you count to the right 5 zero's making your number a negative and you have five zeros making it 7.2x10 to the -5th power
Answer:
is A
Step-by-step explanation:
i did the test and i got it wrong but have the answer
Related Questions
a hunter is practicing his aim using a practice target. he takes 5 shots. all 5 shots hit the target, but they do not hit or surround the bullseye. in addition, all 5 shots are very spread apart on the target. classify the hunter's accuracy and precision.
Answers
The answer is low accuracy and low precision.
Given:
A hunter is practicing his aim using a practice target. he takes 5 shots. all 5 shots hit the target, but they do not hit or surround the bullseye. in addition, all 5 shots are very spread apart on the target.
From the given statements :
All 5 shots hit the target, but they do not hit or surround the bullseye here no shots hit or surround the bullseye means there is no closeness to true value,Hence low accuracy.
All 5 shots are very spread apart on the target here all shots are very spread apart means there is no closeness between the shots,Hence low precision.
Therefore the answer is low accuracy and low precision.
Learn more about the accuracy and precision here:
https://brainly.com/question/15276983
#SPJ1
Miss Gonzales opened an account with a deposit of $2,000,
The account earned annual simple interest.
She did not make any additional deposits or withdrawals.
At the end of 6 years, the balance of the account was $2,696.
What is the annual interest rate on this account?
Answers
Answer:
22.5
Step-by-step explanation:
Can someone please help me!
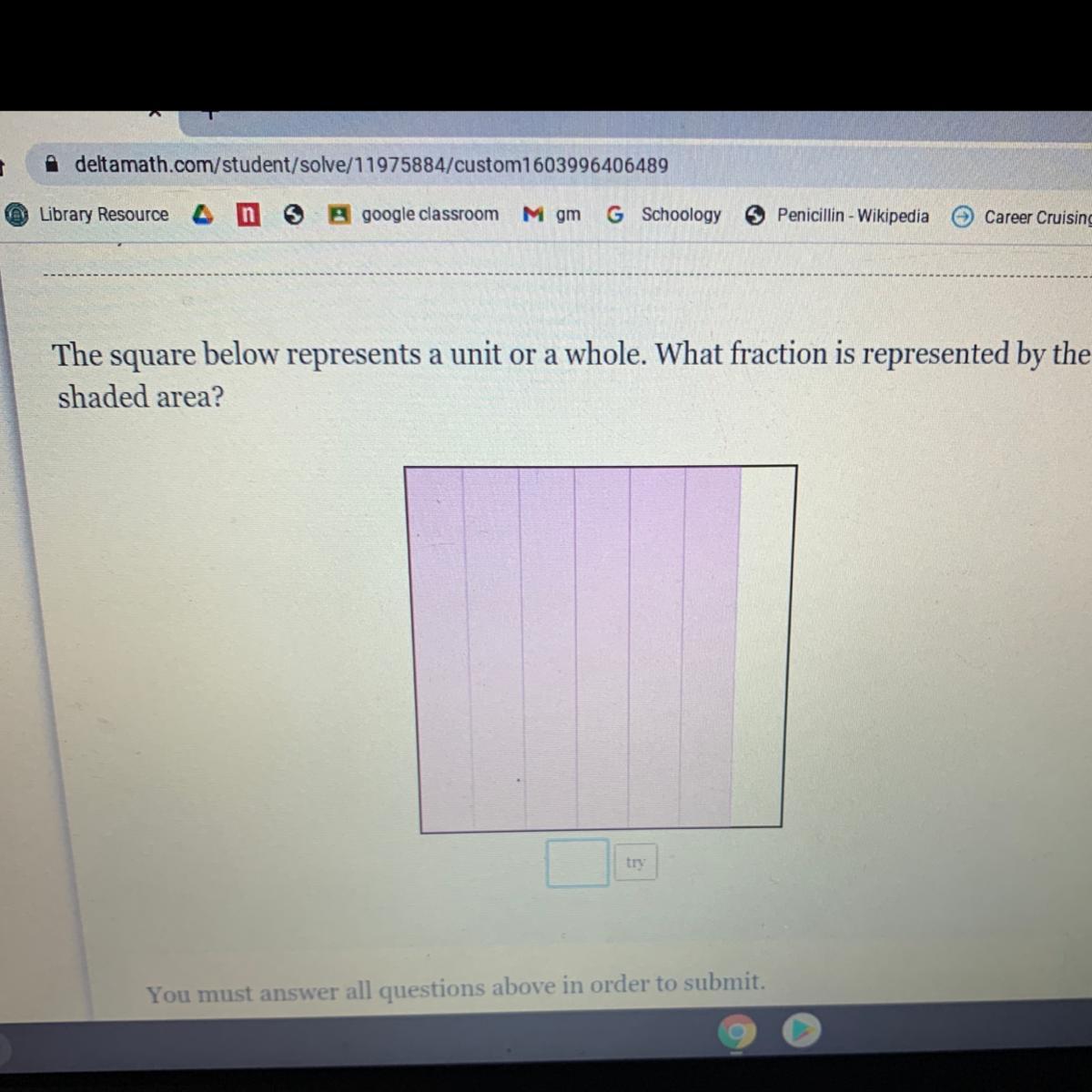
Answers
Answer:
7/6
Step-by-step explanation:
There is 7 total bars and only 6 are shaded, therfore your answer would be 7/6. :)
Answer:
6/7
Step-by-step explanation:
6 out of the 7 areas are shaded.
Have a good day!
which of the following must always be false? e > 12 or f > 12 e = 10 or e = 20 e > 12 and e < 15 e > 10 and e < 7
Answers
The final answer is "e > 10 and e < 7."
The statement that must always be false from the given options is "e > 10 and e < 7.
Statement1: e > 12 or f > 12
This statement is true when e is greater than 12 or f is greater than 12, or both. It is false when both e and f are less than or equal to 12.
Statement 2: e = 10 or e = 20
This statement is true when e is either 10 or 20. It is false when e is any number other than 10 or 20.
Statement 3: e > 12 and e < 15This statement is true when e is greater than 12 and less than 15.
It is false when e is less than or equal to 12 or greater than or equal to 15.
Statement 4: e > 10 and e < 7This statement cannot be true because e cannot be simultaneously greater than 10 and less than 7.
Therefore, this statement must always be false.
The final answer is "e > 10 and e < 7."
https://brainly.com/question/2285414?referrer=searchResults
#SPJ11
The expression that must always be false is e > 12 and e < 15. If e > 12, then it cannot be less than 15, so this expression is contradictory.
Inequalities are mathematical statements that use symbols such as <, >, ≤, or ≥ to compare two values. Inequalities are used to describe relationships between numbers and variables, just like equations.
Inequalities do not require that the values on either side of the inequality be equal.
A logical contradiction is a statement or proposition that is self-contradictory. A statement is contradictory when it asserts that something is true and false at the same time, or that something cannot be true and false at the same time. A logical contradiction is impossible to be true, and therefore must always be false.
For instance, the expression "e > 12 and e < 15" is contradictory since if e is greater than 12, it cannot be less than 15. Therefore, the expression must always be false.
We can determine the expression that must always be false by analyzing each expression given. We will find the expression that contains contradictory values, which is impossible to be true. Here are the four expressions given: e > 12 or f > 12 e = 10 or e = 20 e > 12 and e < 15 e > 10 and e < 7Out of these four expressions, only the third expression e > 12 and e < 15 is contradictory. If e is greater than 12, then it cannot be less than 15. Therefore, e > 12 and e < 15 must always be false.
To learn more on logical contradiction:
https://brainly.com/question/31061612
#SPJ11
C(Q)=aQ^3+bQ^2+cQ+d where a>0,b<0,c>0&d>0 Find the Marginal cost of the given function. Use FOC to find critical Q^∗ at which cost gets minimised. Also state the condition on a,b,c,d such that the cost is minimised.
Answers
The marginal cost of the function C(Q) = aQ^3 + bQ^2 + cQ + d is given by MC(Q) = 3aQ^2 + 2bQ + c.
To find the marginal cost, we need to differentiate the cost function C(Q) with respect to Q. Let's differentiate each term separately:
d/dQ (aQ^3) = 3aQ^2
d/dQ (bQ^2) = 2bQ
d/dQ (cQ) = c
Adding these differentials together, we get the marginal cost:
MC(Q) = 3aQ^2 + 2bQ + c
The critical Q* at which the cost is minimized can be found by setting the marginal cost MC(Q) equal to zero and solving for Q. However, to determine the condition on a, b, c, and d for cost minimization, we would need more information or constraints related to the problem.
To know more about marginal cost follow the link:
https://brainly.com/question/32068966
#SPJ11
If <1 = 140°, then
<2=

Answers
Answer:
∠ 2 = 140°
Step-by-step explanation:
∠ 1 and ∠ 2 are alternate exterior angles and are congruent , so
∠ 2 = ∠ 1 = 140°
About how many students do not prefer non- fiction?
x
A. 60
B. 75
C. 100
D. 150
E. 180
Help

Answers
Answer:
B. 75%
Step-by-step explanation:
If about a quarter ( 25% ) do like non-fiction, then we know that the rest do not prefer it ( 75% )
75 + 25 = 100
If the diagonal of a square is 6 inches long, what is the
area of the square?
Answers
Answer:
18in^2
Step-by-step explanation:
A=1/2d^2=1/2*6^2=18in^2
What is the 1st mistake...

Answers
Answer:
\(\huge\boxed{Step \ 3}\)
Step-by-step explanation:
In Step # 3, We need to divide rather than to subtract. So, the first mistake is done in step 3.

Answer:
\(\Large \boxed{\mathrm{Step \ 4}}\)
Step-by-step explanation:
\(20 +20 \div 4-2\)
Division should be performed first, not subtraction.
\(20+5-2\)

A researcher plans to conduct a significance test at the 0.01 significance level. She designs her study to have a power of 0.90 at a particular alternative value of the parameter of interest. The probability that the researcher will commit a Type II error for the particular alternative value of the parameter at which she computer the power is'
Answers
Answer: 0.10
Step-by-step explanation: The type 2 error is committed when the alternative hypothesis is rejected when it should have been accepted causing the researcher to accept the null hypothesis which is false.
Power is the probability of avoiding a type 2 error. That is ;
Power = 1 - P(type 2 error)
Given that power = 0.90 ; P(type 2 error) = probability of committing a type 2 error.
P(type 2 error)' = 1 - P(type 2 error) = Probability of not committing or avoiding a type 2 error
0.90 = 1 - P(type 2 error)
P(type 2 error) = 1 - 0.90
P(type 2 error) = 0.10
The probability that the researcher will commit a Type II error for the particular alternative value of the parameter at which she computer the power is 0.10.
Calculation of the probability:Since The type 2 error should be committed at the time when the alternative hypothesis should be rejected
So, it can be like
Power = 1 - P(type 2 error)
Given that power = 0.90 ;
P(type 2 error) = probability of committing a type 2 error.
Now
P(type 2 error)' = 1 - P(type 2 error) = Probability of not committing
0.90 = 1 - P(type 2 error)
P(type 2 error) = 1 - 0.90
P(type 2 error) = 0.10
Learn more about probability here: https://brainly.com/question/10695144
Answer this math question for 10 points

Answers
Measure of angle:
∠A = 36.86°
∠B = 90°
∠C = 53.13 °
Measure of side ,
AB = 28
BC = 21
CA = 35
Given triangle ABC.
Right angled at B.
Now, using trigonometric ratios to find angle A , B , C .
Right angled at B : ∠B = 90°
Angle A,
SinA = 21/35
∠A = 36.86
Angle C,
SinC = 28/35
∠C = 53.13
Now measures of side.
To find the length of side use sine rule .
Sine rule:
a/sinA = b/sinB = c /sinC
a = opposite side of angle A .
b = opposite site of angle B .
c = opposite side of angle C.
AB = 28
BC = 21
CA = 35
Hence the sides and angles of the triangles are measured .
Know more about sine rule,
https://brainly.com/question/30339239
#SPJ1
2. in the figure, x is an exterior angle to the triangle below. (
a) explain why x is equal to the sum of the measures of the two nonadjacent interior angles. You need to present the equation with the steps/work that proves this theorem.
Step 1: __
Step 2: __
Step 3: __
(b) what is the measurement of

Answers
Answer:
< x = 120°
Step-by-step explanation
Step 1:
According to angle sum property,
y + 50 + 70 = 180
Step 2:
x and y are linear pairs, forming a supplementary angle.
Therefore,
x + y = 180
Step 3:
Since they are both equal to 180, equate the values
y + 50 + 70 = x + y
x = 70 + 50
x = 120
find the rate of change of a cylinder whnen its radius is 6 feet if its height is always 3/2 times its radius and its increasing a the rate of 2 feet per minute
Answers
324π ft³/ min the rate of change of a cylinder.
What is the volume of the cylinder?
The volume of a cylinder is the density of the cylinder which signifies the amount of material it can carry or how much amount of any material can be immersed in it. Cylinder's volume is given by the formula, πr2h, where r is the radius of the circular base and h is the height of the cylinder.Volume is a three-dimensional quantity that is used to measure the capacity of a solid shape. It means the amount of three-dimensional space a closed figure can occupy is measured by its volume.Find dV/dt
h = 3r/2
Volume of cylinder V = πr²h
v = πr²(3r/2)
v = 3/2 πr³
differentiate both side
\(\frac{dv}{dt} = 3 . \frac{3}{2} \pi r^{2} dr/dt\)
\(\frac{dv}{dt} = 3 . \frac{3}{2} \pi 6^{2} (2)\) = 324π ft³/ min
Learn more about volume of a cylinder
brainly.com/question/16134180
#SPJ4
on tuesday norachai bought seven boxes. on wednesday half of the boxes that he had were destroyed. on tuesday there were only 13 left. how many did he have on monday?
Answers
Answer:
19
Step-by-step explanation:
First you figure out x/2=13
Then you find out x=26
So then you figure out x+7=26
Which is then x=19
The number of boxes Norachai had on Monday is 19 boxes.
Given that, on Tuesday Norachai bought seven boxes.
What is an equation?In mathematics, an equation is a formula that expresses the equality of two expressions, by connecting them with the equals sign =.
Let the number of boxes had on Monday be x.
Now, the number of boxes had on Tuesday
= x+7
Now, on Wednesday half of all the boxes that he had were destroyed
= (x+7)/2
As, given on Thursday he had left boxes = 13
So, (x+7)/2 = 13
⇒ x+7=26
⇒ x=19 boxes
Therefore, the number of boxes Norachai had on Monday is 19 boxes.
To learn more about an equation visit:
https://brainly.com/question/14686792.
#SPJ2
If y* = y1/2(y2 + 1), then what is -3(16* - 9*)?
Answers
If y*=y1/2(y2+1) then -3(16* - 9*) = -10.5y1(y2 + 1), called polynomial.
What is a polynomial?A polynomial is an expression that consists of variables, numbers, and operations (like addition, subtraction, multiplication, and division). It is similar to a mathematical equation, but instead of an equals sign, it uses coefficients and variables in a power form. The power of a polynomial is determined by the highest power of the variables in the expression.
For example, if the polynomial is x2 + 5x + 2, then the power is 2. Polynomials are used in many areas of mathematics, such as calculus, algebra, and number theory.
If y*= y1/2(y2+1) then -3(16* - 9*)
= -3(16(y1/2(y2 + 1)) - 9(y1/2(y2 + 1)))
= -3(8y1(y2 + 1) - 4.5y1(y2 + 1))
= -3(3.5y1(y2 + 1))
= -10.5y1(y2 + 1)
In this problem, 16* is equal to 16(y1/2(y2 + 1)) and 9* is equal to 9(y1/2(y2 + 1)). When -3 is multiplied to 16* - 9*, the result is -3 times the difference of 16* and 9*. The expression then simplifies to -10.5y1(y2 + 1).
To know more about Polynomials, visit:
https://brainly.com/question/2833285
#SPJ1
for tires with a lifetime below 30,000 miles, Grear will refund a customer $1 per 100 miles short of 30,000 . (a) For each tire sold, what is the average cost of the promotion (in $ )? (Use at least 1,000 trials. Round your answer to two decimal places.) $ (b) What is the probability that Grear will refund more than $25 for a tire? (Use at least 1,000 trials. Round your answer to three decimal places.)
Answers
(a) The average cost of the promotion per tire sold by Grear can be calculated by simulating the refund amounts for a large number of trials. (b) To determine the probability that Grear will refund more than $25 for a tire, we again simulate a large number of trials.
(a) The average cost of the promotion per tire sold by Grear can be calculated by simulating the refund amounts for a large number of trials. Assuming each trial represents a tire sold, we can calculate the refund amount for each trial based on the difference between the tire's lifetime and 30,000 miles. By averaging the refund amounts over the trials, we can determine the average cost of the promotion. Let's simulate at least 1,000 trials to obtain a reliable estimate.
(b) To determine the probability that Grear will refund more than $25 for a tire, we again simulate a large number of trials. For each trial, we calculate the refund amount as we did in part (a). Then, we count the number of trials where the refund amount exceeds $25 and divide it by the total number of trials. This will give us the probability of refunding more than $25. By simulating at least 1,000 trials, we can obtain a reasonably accurate estimation of the probability.
Due to the constraints of the current text-based interface, I'm unable to perform the simulations and calculations required to provide you with the specific numerical answers. However, you can apply the described methodology to conduct the simulations using programming or spreadsheet software to obtain the desired results.
Learn more about probability here: brainly.com/question/31828911
#SPJ11
Question 1(Multiple Choice Worth 2 points)
(01.06)
3
Determine the quotient of 1-4
35
O
119
0 23
29
I
2
415
Answers
Answer:
ion know dis one
Step-by-step explanation:
HURRY ITS DUE TODAY!!!
A living room measures 24 feet long and 14 feet wide. A scale drawing is made using a scale factor of 118.
What is the length of the living room in the scale drawing?
Drag and drop a number to correctly complete the statement.
The length of the living room in the scale drawing is Response area ft.
options: 7/9, 4/3, 19/9.
Answers
Using proportions, it is found that the length of the living room in the scale drawing is of 4/3 ft.
What is a proportion?A proportion is a fraction of a total amount, and the measures are related using a rule of three.
The scale factor of 1/18 means that on the drawing, each ft represents 18 ft. Hence how many ft represent 24 feet?
\(f = \frac{1}{18} \times 24 = \frac{24}{18} = \frac{4}{3}\)
Hence, the length of the living room in the scale drawing is of 4/3 ft.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1
Answer:
The guy above is right its 4/3
Step-by-step explanation:
Hope this helped!!!
Please correct me if i'm wrong! :D
What is the smallest positive Integer value of X such that the value of f(x)=2^x+2 exceeds the Value of g(x)=12x+8
Answers
The smallest positive integer value of x for which\(f(x) = 2^x + 2\) exceeds \(g(x) = 12x + 8\) is x = 4.
To find the smallest positive integer value of x for which the value of\(f(x) = 2^x + 2\) exceeds the value of g(x) = 12x + 8, we need to compare the two functions and determine when the inequality is satisfied.
Setting up the inequality, we have:
\(2^x + 2 > 12x + 8\)
First, let's simplify the inequality by subtracting 8 from both sides:
\(2^x - 6 > 12x\)
Now, we can try to solve this inequality by considering different values of x.
However, it is challenging to find an exact solution by hand due to the exponential nature of \(2^x.\)
Therefore, let's graph the two functions,\(f(x) = 2^x + 2\) and g(x) = 12x + 8, to visually determine the point of intersection.
Upon graphing the functions, we observe that the graphs intersect at some point.
We can see that the value of f(x) starts to exceed g(x) as x increases.
To find the smallest positive integer value of x for which f(x) exceeds g(x), we need to analyze the graph and determine the first integer value after the intersection point where f(x) is greater than g(x).
Examining the graph, we find that the smallest positive integer value of x for which f(x) exceeds g(x) is x = 4.
Therefore, the answer is x = 4.
For similar question on positive integer value.
https://brainly.com/question/30522847
#SPJ11
Find the product using the correct number of significant digits.
0.025 x 4.07 =
Answers
Answer: 0.10175
Step-by-step explanation:
First, bring the decimal points to the right for both numbers, to be a total of 5 decimal points to the right. Then, with the numbers 25 and 407, multiply them, and we get 10175. Then, we must bring the 5 decimal points back, and we end up with 0.10175.
Answer: 0.10
Step-by-step explanation:
on time4llearning
Given the regression equation y-hat = 15.6 - 3.8x, the predicted y for x = 3 is ___________.
Answers
Work Shown:
y = 15.6 - 3.8x
y = 15.6 - 3.8*3
y = 4.2
Write an algebraic expression that represents
“8 minus the quantity b divided by 6.”
Answers
Someone help me pls
47 as the sum of ______

Answers
9514 1404 393
Answer:
(a) 6² +3² +1² +1² = 47
(b) 5² +4² +2² +1² +1² = 47
(c) 3³ +4² +2² = 47
Step-by-step explanation:
It can work reasonably well to start with the largest square less than the target number, repeating that approach for the remaining differences. When more squares than necessary are asked for, then the first square chosen may need to be the square of a number 1 less than the largest possible.
The approach where a cube is required can work the same way.
(a) floor(√47) = 6; floor(√(47 -6^2)) = 3; floor(√(47 -45)) = 1; floor(√(47-46)) = 1
__
(b) floor(√47 -1) = 5; floor(√(47-25)) = 4; ...
__
(c) floor(∛47) = 3; floor(√(47 -27)) = 4; floor(√(47 -43)) = 2
Match each pair of inverse operations.
1. cubing
subtraction
2. multiplication
division
3. squaring
taking the square root
4. addition
taking the cube root
Answers
squaring- taking the square root
addition- subtraction
multiplication- division
Solve for x on the interval 0
Separate answers by commas, arranged from lowest to highest
2sin(x)cos(x)=−cos(x)
Question 14 Solve for x on the interval 0 < x < 2π Separate answers by commas, arranged from lowest to highest 2 sin(x) cos(x) - cos(x) 10 pts
Answers
To solve the equation 2sin(x)cos(x) = -cos(x) on the interval 0 < x < 2π, we can simplify the equation and solve for x.
First, let's factor out cos(x) from both terms:
cos(x)(2sin(x) - 1) = 0
Now, we have two possible cases:
Case 1: cos(x) = 0
On the interval 0 < x < 2π, cos(x) is equal to 0 at x = π/2 and x = 3π/2.
Case 2: 2sin(x) - 1 = 0
Solving for sin(x), we get sin(x) = 1/2. On the interval 0 < x < 2π, sin(x) is equal to 1/2 at x = π/6 and x = 5π/6.
Therefore, the solutions to the equation 2sin(x)cos(x) = -cos(x) on the interval 0 < x < 2π are x = π/2, x = 3π/2, x = π/6, and x = 5π/6. Arranged from lowest to highest, the solutions are:
π/6, π/2, 5π/6, 3π/2
Learn more about equation here:
https://brainly.com/question/29657992
#SPJ11
People In town voted for a new councillor
Answers
Answer:
what's your question???
I can't understand:-//
Robbie made a scale drawing of a neighborhood park. A Soccer field in the park is 14 inches long
in the drawing. The actual field is 98 yards long. What is the scale of the drawing? 1 inch = _ yards
Answers
Answer:
14 it is not 14
Step-by-step explanation:
Answer:
ITS NOT 14
Step-by-step explanation:
I got it wrong anything besides that
Helppppp with this please

Answers
Answer:180
Step-by-step explanation:
hope this helps
Answer:
0.8 mm
Step-by-step explanation:
Given that you require the actual size.
The ant has been magnified by 15 X
To find the actual size divide magnified size by 15
actual size = 12 mm ÷ 15 = 0.8 mm
an economist wondered if people who go grocery shopping on weekdays go more or less often on fridays than any other day. she figured that if it were truly random, 20% of these shoppers would go grocery shopping on fridays. she randomly sampled 75 consumers who go grocery shopping on weekdays and asked them on which day they shop most frequently. of those sampled, 24 indicated that they shop on fridays more often than other days. the economist conducts a one-proportion hypothesis test at the 1% significance level, to test whether the true proportion of weekday grocery shoppers who go most frequently on fridays is different from 20%. (a) which answer choice shows the correct null and alternative hypotheses for this test?
Answers
The true percentage of grocery shoppers that shop most frequently on Fridays from Monday to Friday, which is 20%, cannot be determined statistically. The value of p is 0.0130.
To solve this problem, we run a hypothesis test about the population proportion.
Proportion in the null hypothesis (p0) = 0.2
Sample size (n) = 75
Sample proportion (sp) = 24/75 = 0.32
Significance level = 0.01
\(H_{o}\) : p = 0.2
\(H_{a}\) : p ≠ 0.2
Test statistic percentage = ( \(sp\) - \(p_{o}\) ) / \(\sqrt{\frac{(p_{o})(1-p_{o} )}{n} }\)
Left critical \(z_{0.01}\) = -2.5758
Right critical \(z_{0.01}\) = 2.5758
Calculated statistic = \(\frac{0.32-0.2}{\sqrt{\frac{(0.32)(1-0.32)}{75} } }\) = 2.2278
Since, -2.5758 < Test statistic < 2.5758, the null hypothesis cannot be rejected. There is not enough statistical evidence to state that the true proportion of grocery shoppers from Monday to Friday that goes most frequently on Fridays is different from 20%. The p - value is 0.0130.
Therefore,
The true percentage of grocery shoppers that shop most frequently on Fridays from Monday to Friday, which is 20%, cannot be determined statistically. The value of p is 0.0130.
To learn more about Percentage visit :
brainly.com/question/14869182
#SPJ4
Plzz I need help asap 45 points Find The missing side


Answers
Answer:
4x^2
Step-by-step explanation:
Call the unknown side y
The area of the figure is
8x^3 *y = 32 x^5
Divide each side by 8 x^3
8x^3 *y / 8x^3 = 32 x^5 / 8x^3
y = 4 x^2
The unknown side is 4 x^2
Answer:
4x^2
Step-by-step explanation:
32x^5 / 8x^3 = 4x^2
or 4x^2 * 8x^3 = 32x^5
so 4x^2