Kiemanh is solving the equation 15 r minus 6 r = 36. What is the value of r? 2 4 15 27
Answers
Answer:
the actual answer is B.) 4
Step-by-step explanation:
just took the topic test
Answer:
B
Step-by-step explanation:
Related Questions
Maxine needs to rent a car for a week. She has $500 to cover the rental. If the car costs $45 a day and $0.25 a mile, how many miles can she drive for $500?
Answers
Answer:
220 miles
Step-by-step explanation:
For 1 week=7 days
So that means $35*7=245
300-245=55
$55/.25= 220 miles
Answer:
2,000 miles
Step-by-step explanation:
All you need is how many miles Maxine can drive for 500 dollars, so you can disregard the 45 dollar charge. you would just do $500 divided by $0.25, which gets you 2,000.
A regular pentagon has perimeter 42.5 cm.
What is the length of each side?
Answers
Answer:
8.5 cm
Step-by-step explanation:
A regular pentagon has 5 congruent sides , then
length of each side = perimeter ÷ 5 = 42.5 ÷ 5 = 8.5 cm
You are solving a measurement problem where the numbers 4.0286 × 109 and 3.1 × 10−4 are divided. How many significant digits should the quotient have?
Answers
The quotient will have 2 significant figures.
The digits in the number , that contribute to the degree of accuracy can be defined as Significant Figures.
For Example ; 2.096 has 4 significant Figures
0.069 has 2 significant figures.
Rule Of Significant for Multiplication & Division :
Rule says in multiplication or division , the final result should retain as many significant figures as there are in the original number with the least significant figures.
In the given question
4.0286 × 10⁹ = 5 significant figures
3.1 × 10⁻⁴ = 2 significant figures
The quotient on dividing the numbers is 1.2995 x 10¹³ will have 2 significant figures as the least significant from 5 and 2 is 2.
Therefore , the quotient will have 2 significant figures.
The given question is incomplete , the complete question is
You are solving a measurement problem where the numbers 4.0286×10⁹ and 3.1×10⁻⁴ are divided. How many significant digits should the quotient have?
Learn more Significant Figures here https://brainly.com/question/24491627
#SPJ1
Consider a continuous-time Markov chain with three states 1, 2, 3, 4, 5 and transition rates q12=1, q13 = 2, q21 = 0, q23 = 3, q31 = 0, q32 = 0. (1) Write the system of ODEs for the corresponding transition probabilities Pᵢⱼ (t) . (2) Suppose that the initial state is 1. What is the probability that after the first transition, the process X(t) enters state 2?
Answers
the probability of transitioning from state 1 to state 2 after the first transition is:
P(X(t) enters state 2 after the first transition | X(0) = 1) = 1 / 3
To write the system of ordinary differential equations (ODEs) for the transition probabilities Pᵢⱼ(t) of the given continuous-time Markov chain, we need to consider the rate at which the system transitions between different states.
Let Pᵢⱼ(t) represent the probability that the Markov chain is in state j at time t, given that it started in state i at time 0.
The ODEs for the transition probabilities can be written as follows:
dP₁₂(t)/dt = q₁₂ * P₁(t) - q₂₁ * P₂(t)
dP₁₃(t)/dt = q₁₃ * P₁(t) - q₃₁ * P₃(t)
dP₂₁(t)/dt = q₂₁ * P₂(t) - q₁₂ * P₁(t)
dP₂₃(t)/dt = q₂₃ * P₂(t) - q₃₂ * P₃(t)
dP₃₁(t)/dt = q₃₁ * P₃(t) - q₁₃ * P₁(t)
dP₃₂(t)/dt = q₃₂ * P₃(t) - q₂₃ * P₂(t)
where P₁(t), P₂(t), and P₃(t) represent the probabilities of being in states 1, 2, and 3 at time t, respectively.
Now, let's consider the second part of the question: Suppose that the initial state is 1. We want to find the probability that after the first transition, the process X(t) enters state 2.
To calculate this probability, we need to find the transition rate from state 1 to state 2 (q₁₂) and normalize it by the total rate of leaving state 1.
The total rate of leaving state 1 can be calculated as the sum of the rates to transition from state 1 to other states:
total_rate = q₁₂ + q₁₃
Therefore, the probability of transitioning from state 1 to state 2 after the first transition can be calculated as:
P(X(t) enters state 2 after the first transition | X(0) = 1) = q₁₂ / total_rate
In this case, the transition rate q₁₂ is 1, and the total rate q₁₂ + q₁₃ is 1 + 2 = 3.
Therefore, the probability of transitioning from state 1 to state 2 after the first transition is:
P(X(t) enters state 2 after the first transition | X(0) = 1) = 1 / 3
Learn more about probability here
https://brainly.com/question/32117953
#SPJ4
Find the smallest natural number that is divisible by all of the natural numbers from 1 to 12.
Answers
Answer:
The smallest number divisible by the natural numbers 1 to 12 is 1
Any point on the parabola can be labeled (x,y) as shown.
1. What are the distances from the point (x,y)(x,y) to the focus of the parabola and the directrix?
Select two answers.
distance to the focus: √(x+3)2+(y−3)2
distance to the directrix: |y−4|
distance to the directrix: |x−4|
distance to the focus: √(x−2)2+(y+3)2
distance to the focus: √(x+3)2+(y−2)2
distance to the directrix: |y+4|
2. What is the correct standard form of the equation of the parabola?

Answers
Answer:
Part A)
2nd and 5th choice.
Part B)
\(\displaystyle y=-\frac{1}{4}x^2-\frac{3}{2}x+\frac{3}{4}\)
Step-by-step explanation:
For the given parabola, our focus is (-3, 2), and our directrix is given by y = 4.
Part A)
We are given a point (x, y) on the parabola.
Since our directrix is an equation of y, the distance from (x, y) to the directrix will simply be the absolute value of the difference in y-values. So:
\(d_1=|y-4|=|4-y|\)
Recall the distance formula:
\(d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2\)
Our distance from (x, y) to the focus (-3, 2) can be determined using the distance formula. Let (x, y) be (x₂, y₂) and let our focus (-3, 2) be (x₁, y₁). Therefore:
\(d_2=\sqrt{(x-(-3))^2+(y-2)^2}=\sqrt{(x+3)^2+(y-2)^2\)
Hence, for Part A, our answers are the 2nd and 5th choices.
Part B)
Recall that by the definition of a parabola, any point (x, y) on it is equidistant to the directrix and focus. Hence:
\(\sqrt{(x+3)^2+(y-2)^2}=|y-4|\)
Solve for y. Square both sides. We may remove the absolute value since anything squared is positive:
\((x+3)^2+(y-2)^2=(y-4)^2\)
Square:
\(x^2+6x+9+y^2-4y+4=y^2-8y+16\)
Rearrange:
\((x^2+6x+13)+(-16)=(y^2-y^2)+(-8y+4y)\)
Combine like terms:
\(x^2+6x-3=-4y\)
Divide both sides by -4. Hence, our equation is:
\(\displaystyle y=-\frac{1}{4}x^2-\frac{3}{2}x+\frac{3}{4}\)
Heyo! :D
Distance to the directrix is |y - 4|.
Distance to the focus is \(\sqrt{(x+3)^2+(y-2)^2}\).
Hope this helps! If so, please lmk! Tysm and good luck!
Please help me solve this problem ASAP
I have a huge test coming up and I need to understand the questions
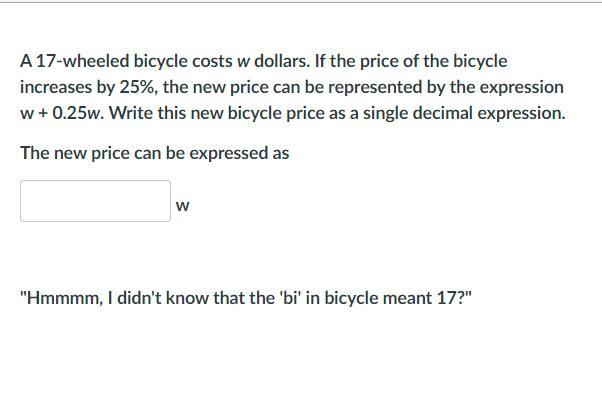
Answers
Answer:
1.25w
Step-by-step explanation:
w+0.25 is really 1w+0.25, therefore adding the two would equal 1.25w, here is how to solve it:
\(w+0.25w\\1*w+0.25w\\1w+0.25w\\1.25w\)
Evaluate5! 7!____3! 6!Simple as much as possible
Answers
ANSWER:
140
EXPLANATION:
Given:
\(\frac{5!7!}{3!6!}\)We can go ahead and simplify as seen below;
\(\frac{5!7!}{3!6!}=\frac{(5*4*3*2*1)(7*6*5*4*3*2*1)}{(3*2*1)(6*5*4*3*2*1)}=5*4*7=140\)Therefore the answer is 140
What are like terms examples?
Answers
Step-by-step explanation:
Examples of like terms in math are x, 4x, -2x, and 7x. These are like terms because they all contain the same variable, x. The terms 8y2, y2, and -2y2 are like terms as well. These all contain the same variable, y, raised to the second power.
Show transcribed dataSuppose that a particular NBA player makes 94% of his free throws. Assume that late in a basketball game, this player is fouled and is awarded two free throws. a. What is the probability that he will make both free throws? (to 4 decimals) b. What is the probability that he will make at least one free throw? (to 4 decimals) c. What is the probability that he will miss both free throws? (to 4 decimals) d. Late in a basketball game, a team often intentionally fouls an opposing player in order to stop the game clock. The usual strategy is to intentionally foul the other team's worst free-throw shooter. Assume the team's worst free throw shooter makes 56% of his free throws. Calculate the probabilities for this player as shown in parts (a), (b), and (c), and show that intentionally fouling this player who makes 56% of his free throws is a better strategy than intentionally fouling the player who makes 94% of his free throws. Assume as in parts (a), (b), and (c) that two free throws will be awarded. What is the probability that this player will make both throws? (to 4 decimals) What is the probability that will make at least one throw? (to 4 decimals) What is the probability that this player will miss both throws? (to 4 decimals)
Answers
the probability of him making at least one free throw is lower (0.8044 vs. 0.9964), which means the opposing team has a better chance of preventing the other team from scoring points.
a. To find the probability that the player will make both free throws, we simply multiply the probability of making one free throw by itself: 0.94 * 0.94 = 0.8836 (to 4 decimals).
b. To find the probability that he will make at least one free throw, we can use the complement rule. The complement of making at least one free throw is missing both free throws, so we can find the probability of missing both free throws and subtract that from 1: 1 - 0.06 * 0.06 = 0.9964 (to 4 decimals).
c. To find the probability that he will miss both free throws, we multiply the probability of missing one free throw by itself: 0.06 * 0.06 = 0.0036 (to 4 decimals).
d. For the team's worst free throw shooter who makes 56% of his free throws, the probabilities are as follows:
a. The probability of making both free throws is 0.56 * 0.56 = 0.3136.
b. The probability of making at least one free throw is 1 - the probability of missing both free throws: 1 - 0.44 * 0.44 = 0.8044.
c. The probability of missing both free throws is 0.44 * 0.44 = 0.1936.
Comparing the probabilities for the two players, we can see that intentionally fouling the worst free throw shooter is a better strategy. This is because the probability of him missing both free throws is higher (0.1936 vs. 0.0036), which means the opposing team has a greater chance of gaining possession of the ball. In addition, the probability of him making at least one free throw is lower (0.8044 vs. 0.9964), which means the opposing team has a better chance of preventing the other team from scoring points.
To know more about complement rule click here:
brainly.com/question/29146128
#SPJ4
r is a relation on the set of all nonnegative integers. (a,b) is in r if a and b have the same remainder when divided by 5
Answers
The relation accepts reflexive, symmetry, and transitive.
Recall that a relation R is reflexive if the element (x, x) belongs to R for all elements X in the domain of R.
If (x, y) belongs to R, then follows that (y, x) must likewise belong to R, making the situation symmetric.
And it is transitive if (x, y) and (y, z) belongs to R necessarily implies that (x, z) belongs to R.
Given r is a relation on the set of all nonnegative integers R(a,b)
Reflexive - YES. A given number a will always have the same remainder when divided by 5.
Symmetric - YES. If a and b have the same remainder when divided by 5, then b and a are the same pair, so again they will have the same remainder.
Transitive - YES. If a and b as well as b and c have the same remainder when divided by 5, this is possible if both a and c also have the same remainder when divided by 5.
Therefore the relation accepts reflexive, symmetry, and transitive.
To learn more about reflexive & symmetry visit
https://brainly.com/question/3014815
#SPJ4
Which expression is equivalent to the following complex fraction? 1 StartFraction 1 Over y EndFraction divided by 1 minus StartFraction 1 Over y EndFraction StartFraction (y 1) (y minus 1) Over y squared EndFraction StartFraction y 1 Over y minus 1 EndFraction StartFraction y minus 1 Over y 1 EndFraction StartFraction y squared Over (y 1) (y minus 1) EndFraction.
Answers
The expression that is equivalent to (1 + (1/y))/(1 - (1/y)) is; (y + 1)/(y - 1)
We want to find the expression that is equivalent to;
(1 + (1/y))/(1 - (1/y))
Let us first simplify the numerator (1 + (1/y))
By using LCM method, we can express the numerator as;
(y + 1)/y
Similarly, for the denominator we have;
(1 - (1/y)) = (y - 1)/y
Thus the original expression is now;
((y + 1)/y)/((y - 1)/y)
y will cancel out to give us;
(y + 1)/(y - 1)
Read more about simplification of fractions at; https://brainly.com/question/17083350
Answer:
B. y+1/y-1
Step-by-step explanation:
edge 2022
PLEASE HELP ASAP!!!!!!!!

Answers
Answer:
The lie is #3.
Step-by-step explanation:
1 divided by 7^-3 is not equal to 7^-3. 7^-3/1 is equal to 7^-3 though.
In order to make a profit, a retailer will mark up the cost of an item. If the cost of the item is $42 but it is sold for
$89, what is the mark up rate for the item?
Round your answer to the whole percent.
Answers
I need help with 2 questions
The one in the picture and this one
In Parallelogram EFGH, diagonals HF and EG intersect at point D. Give HD = x? - 34 and
DF = x7 - 4x. What is HF? Show your work
WILL GIVE BRAINLIEST!

Answers
The value of HF is= 76.5
What is a Parallelogram?A parallelogram is a straightforward quadrilateral with two sets of parallel sides in Euclidean geometry. In a parallelogram, the opposing or confronting sides are of equal length, and the opposing angles are of equal size.
The diagonals of a parallelogram bisect each other (divide into two equal parts). Therefore, point D is the midpoint of diagonal HF, and so HD = DF.
Find the value of x by equating the expressions for HD and DF and solving for x:
HD = HF
x^2 - 34 = x^2 - 4x
x = 8.5
To find the length of HF, substitute the found value of x into the sum of the expressions of HD and DF:
HF = HD + DF
HF = x^2 - 34 + x^2 - 4x
HF = 76.5
Therefore, the length of HF is 76.5 units.
Read more about parallelograms here:
https://brainly.com/question/3050890
#SPJ1

I need help with this math question please I don’t understand? And also what is this kind of math called ?

Answers
Armand and Jill are 247.5 miles apart, and driving toward each other. Armand is driving at a rate of 50 miles per hour and Jill is driving at a rate of 60 miles per hour.
A table showing Rate in miles per hour, Time in hours, and Distance in miles. The first row shows Armand, and has 50, t and 50 t. The second row shows Jill, and has 60, t, and 60 t
Use the scenario and table to determine which statements are true. Check all that apply.
Armand and Jill will each travel the same distance to meet.
Armand and Jill will travel the same amount of time to meet.
The equation used to find the time, t, it will take for them to meet is 50t + 60t = 247.5.
It will take 4.5 hours for them to meet.
Jill will travel 135 miles to meet Armand.
Answers
Answer:
B.C.E.
just did it on edge 2021
Answer:
yes the other person is right its B.C.E
Step-by-step explanation:
A competitive firm has a short-run total cost curve STC (q)= 0.1q^2 +10q +40
a. Identify SVC and SFC.
b. Find and plot the SAC and SAVC curves.
c. For this function, the SMC curve is given by SMC (q)= 0.2q +10.
Answers
To plot the SMC curve, we can take the derivative of the AC curve with respect to q:
dAC/dq = -40/q^2 + 0.
a. The short-run total cost (STC) is the sum of variable costs (SVC) and fixed costs (SFC). In this case, the function for STC is given by:
STC(q) = 0.1q^2 + 10q + 40
To find the variable cost (SVC), we need to subtract the fixed cost (SFC) from STC. Since the fixed cost is constant, it is equal to the STC at zero output. Therefore:
SFC = STC(0) = 0.1(0)^2 + 10(0) + 40 = 40
To find the variable cost, we subtract SFC from STC:
SVC(q) = STC(q) - SFC = 0.1q^2 + 10q
Therefore, SVC(q) = 0.1q^2 + 10q and SFC = 40.
b. The average cost (AC) is the total cost per unit of output. It is the sum of the average fixed cost (AFC) and the average variable cost (AVC):
AC(q) = AFC(q) + AVC(q)
The average fixed cost (AFC) is the fixed cost per unit of output. It decreases as the output increases. In this case, AFC is:
AFC(q) = SFC / q = 40 / q
The average variable cost (AVC) is the variable cost per unit of output. It increases as the output increases due to diminishing marginal returns. In this case, AVC is:
AVC(q) = SVC(q) / q = (0.1q^2 + 10q) / q = 0.1q + 10
Therefore, the average cost (AC) is:
AC(q) = AFC(q) + AVC(q) = 40/q + 0.1q + 10
To plot the curves, we need to find the points where the average cost (AC) is minimized, and then plot the average fixed cost (AFC), average variable cost (AVC), and average cost (AC) curves passing through that point.
To find the minimum point of AC, we take the derivative of AC with respect to q and set it equal to zero:
dAC/dq = -40/q^2 + 0.1 = 0
Solving for q, we get:
q = 20
Therefore, the minimum point of AC is at q = 20. Plugging this into the equations for AFC and AVC, we get:
AFC(20) = 2
AVC(20) = 12
Now we can plot the curves. Note that AFC decreases as output increases, and AVC increases as output increases.
The AC curve is U-shaped because the AFC curve decreases more rapidly than the AVC curve increases, up to the minimum point, and then the opposite happens. The curves are:
AFC(q) = 40/q
AVC(q) = 0.1q + 10
AC(q) = 40/q + 0.1q + 10
Note that the curves intersect at q = 20, AFC = 2, AVC = 12, and AC = 22.
c. The short-run marginal cost (SMC) is the additional cost of producing one more unit of output. In this case, the SMC is given by:
SMC(q) = dSTC/dq = 0.2q + 10
To plot the SMC curve, we can take the derivative of the AC curve with respect to q:
dAC/dq = -40/q^2 + 0.
To learn more about output, refer below:
https://brainly.com/question/13736104
#SPJ11
1/3 x4 need help asap
Answers
Answer:
4/3 or 1 1/3
Step-by-step explanation:
a³+ 1 + 2a² + 2a, a3 - 1 and a²+a2 +1
Answers
HOPE IT HELPS YOU
MARK AS BRAINLIEST

1)) Tammy treated her sister to lunch while visiting her in Somerville. Their lunch cost $350,
and the sales tax in Somerville is 4%. If Tammy left a 20% tip on the $350, how much in
total did she pay?
Answers
Answer:
the other amount of cash she pays equals to 24 percent so times 350 by 1.24 which equals to $434
Answer:434
Step-by-step explanation:
Total price with $350
Sales tax 4% = $14
And tip 20%= $70
Total paid= $434
what is m
P-Q (3x-6)
Q-R (x+2)

Answers
Answer:
132 degrees.
Step-by-step explanation:
Line PQS is 180 degrees and angle PQR and angle PRS are supplementary. Since you know this, both of these equations must equal 180 degrees.
(3x-6) +(x+2) = 180
Above is your equation to find X. This is only one part because you need to find the missing value, X. So now you need to solve for x. Assuming you know how to isolate x, you should get:
x = 46
Now the question asks for the angle of line PQR. As you can see in the graph, line PQR is the (3x-6). Since you now know what x is, you can plug in x with 46.
3(46)-6 = 132
Angle PQR is 132 degrees.
a storage bin in the shape of a rectangular prism has a volume of 10x3 9x2 2x. what linear expressions can represent possible dimensions of the bin?
Answers
For a storage bin of rectangular prism and volume of prism, 10x³ + 9x² + 2x, then the linear expressions can represent possible dimensions of the bin is x( 2x + 1)(5x+2).
The volume of a rectangular prism is also called the capacity that it can hold or the space occupied by it. The formula used to calculate the volume of a rectangular prism is written as Volume (V) = height of the prism × width × length--(1). It is represented in cubic units such as cm³, m³etc. We have a storage bin in the shape of a rectangular prism.
Volume of prism, V = 10x³ + 9x² + 2x.
We have to determine the linear expression which represent possible dimensions of bin.
Now, Volume expression is V = 10x³ + 9x² + 2x.
Using factorization, for determining dimensions,
= x( 10x² + 9x + 2) ( taken out x )
Using the the middle term splitting in Quadratic form,
= x( 10x² + 5x + 4x + 2)
= x( 5x( 2x + 1) + 2( 2x + 1)) ( making pairs and factorize)
V = x( 2x + 1)(5x+2)
from equation (1), height of the prism × width × length that is dimensions = x( 2x + 1)(5x+2). Hence, requird expression is x ( 2x + 1)(5x+2).
For more information about rectangular prism, visit :
https://brainly.com/question/30490370
#SPJ4
Overbooking of passengers on intercontinental flights is a common practice among airlines, Aircraft which are capable of carrying 300 passengers are booked to carry 320 passengers. If on average 10% of passengers who have a booking fail to turn up for their flights, what is the probability that at least one passenger who has a booking will end up without a seat on a particular flight? a. 0.0888 1.0.0099 C. 0.0257 d.0.7560
Answers
The probability that at least one passenger who has a booking will end up without a seat on a particular flight is 0.0257. The answer to this question is Option C)
What is overbooking?
Overbooking is the practice of making more reservations for a flight than there are seats available on the plane. Airlines frequently overbook in an attempt to compensate passengers who do not show up for their scheduled flights.
The airline may select to request some passengers to give up their seats in exchange for compensation if everyone shows up. This compensation is frequently more than the cost of the ticket and may include cash, vouchers, or even a free flight.
Overbooking of passengers on intercontinental flights is a common practice among airlines, Aircraft which are capable of carrying 300 passengers are booked to carry 320 passengers.
If on average 10% of passengers who have a booking fail to turn up for their flights, the probability that at least one passenger who has a booking will end up without a seat on a particular flight is as follows:
Let’s consider the scenario: On a particular flight, there are 320 seats available, and the airlines had sold 320 tickets. However, there is a 10% chance that a passenger might not show up.
Thus, there are two probabilities, either a passenger may not show up or all passengers will show up.
On average, 10% of the passengers do not show up, and 90% show up for the flight. The probability of all the passengers showing up is given by: P (All passengers show up) = 0.9^320 ≈ 0.
Thus, the probability of a passenger not showing up is:P (At least one passenger not showing up) = 1 – P (All passengers show up) = 1 – 0 = 1.
This indicates that there is a probability of 1 that a passenger will not show up and hence there will be a seat available for every passenger who has a booking.
Thus, the probability that at least one passenger who has a booking will end up without a seat on a particular flight is given by:P (At least one passenger will end up without a seat) = 1 – 1 = 0. Hence, the probability is 0.
Therefore, the correct answer is option C.
To know more about overbooking refer here:
https://brainly.com/question/28250329#
#SPJ11
HELPPPPPPP ! LOOK AT ATTACHMENT Where does the horizontal asymptote lie for ?
A.
B.
C.
D.

Answers
Answer:
D
Step-by-step explanation:
The topic is about secant tangle angles

Answers
Answer:
41
Step-by-step explanation:
360-(104+104+111)=41
Please help on all 5 of these as soon as you can

Answers
Answer:
1. no 2. no I think I'm not sure if this is right
Four drivers recorded the distance they drove each day for a week. Which driver's data set has a mode that is greater than the mean or median AND a median with the lowest value of the three measures?
a
Kadisha: 8, 17, 23, 16, 17, 18, 125
b
Cole: 14, 26, 34, 22, 47, 22, 45
c
Fabian: 7, 12, 11, 23, 13, 23, 30
d
Ling: 52, 36, 41, 31, 31, 37, 59
Answers
Driver's data set that has a mode that is greater than the mean or median is Fabian and a median with the lowest value of the three measures is Kadisha.
Data of Fabian: 7, 12, 11, 23, 13, 23, 30
Mean = sum of all observation / total no. of observation
Mean = (7+ 12+ 11+ 23+ 13+ 23+ 30) / 7
Mean = 17
Mode = most repeating observation
Mode = 23
For median we have to write observation in ascending order
7,11,12,13,23,23,30
Median = (N+1)/2
Where N = No. of observation
Median = (7+1)/2
Median = 4th observation
Median = 13
Here mode that is greater than the mean or median.
similarly for,
Kadisha: 8, 17, 23, 16, 17, 18, 125
Mean = 32
Median = 17
Mode = 17
Here median with the lowest value of the three measures.
To know more about mode click her
https://brainly.com/question/30891252
#SPJ4
A cooking group made 240 oz of spaghetti sauce to sell at a county fair. The group can pour the sauce into small jars that hold 8 oz and large jars that hold 16 oz. The group leader made a sketch to show the line representing the different combinations of jar sizes the group can use. The x-axis represented the number of small jars and the y-axis represented the number of large jars. The group leader labeled only the intercepts.
Answers
The points that the cooking group leader did label are (0,15) and (30,0). Thus, the correct answer is C.
As your first step, find the function that best represents x and y:
x is the number of 8-ounce jars x.y is the number of 16-ounce jars.Hence, the function is:
8x + 16y = 240The group leader labeled (0,15) on the y-axis, which means that when the number of small jars is 0, the group can use 15 large jars. This means that 15 large jars can hold 240 oz of spaghetti sauce, which is true since:
8x + 16y = 240(15x0) + (16x15) = 2400 + 16 x 15 = 24015 x 15 = 240The group leader also labeled (30,0) on the x-axis, which means that when the number of large jars is 0, the group can use 30 small jars. This means that 30 small jars can hold 240 oz of spaghetti sauce, which is true since:
8x + 16y = 240(8x30) + (16x0) = 2408 x 30 + 0 = 2408 x 30 = 240The function that represents this line is 8x + 16y = 240, where x is the number of small jars and y is the number of large jars. The line passes through (0,15) and (30,0) as the two intercepts.
This question should be written as:
A cooking group made 240 oz of spaghetti sauce to sell at a county fair. The group can pour the sauce into small jars that hold 8 oz and large jars that hold 16 oz. The group leader made a sketch to show the line representing the different combinations of jar sizes the group can use. The x-axis represented the number of small jars and the y-axis represented the number of large jars. The group leader labeled only the intercepts. Which points did the group leader label?
A. (0,8) and (16,0) B. (0,30) and (15,0) C. (0,15) and (30,0) D. (0,16) and (8,0)The correct answer is C.
Learn more about x axis here: brainly.com/question/27946240
#SPJ4
€50,
Assignment
find the nth term of a GP vouch
first term = 5, commora_roteo=2
51
first term=16 commonratio=3
Second, term = 44, common ratio = 2/₂
Sixth term = 243, common ratio = -3
Answers
The common ratio (r) = 8.8 ÷ 5 = 1.76The nth term is given by a × r^(n-1) = 5 × (1.76)^(n-1)2. First term = 16, common ratio = 3The second term is given by a × r^(1-1) = a = 16 × 3^(1-1) = 16 × 1 = 16To find the nth term, we use the formula a × r^(n-1) = 16 × 3^(n-1)3. Sixth term = 243, common ratio = -3The first term is given by a = 243 ÷ (-3)^(6-1) = 3The nth term is given by a × r^(n-1) = 3 × (-3)^(n-1)Therefore, the nth terms for the three given scenarios are:5 × (1.76)^(n-1)16 × 3^(n-1)3 × (-3)^(n-1)
A geometric progression (GP) is a sequence of numbers in which each term after the first is obtained by multiplying the preceding term by a fixed number known as the common ratio (r).The nth term of a geometric progression (GP) with first term (a) and common ratio (r) is given by a × r^(n-1). In order to find the nth term of the given geometric progression, we need to first determine the value of the common ratio (r) and the first term (a) for each of the three different scenarios given. Once we have these values, we can use the formula above to calculate the nth term for each scenario. 1. First term = 5, common ratio = 2/5To find the common ratio (r) we divide the second term by the first term.44 ÷ 5 = 8.8 (approx.)
for more such question on common ratio
https://brainly.com/question/1509142
#SPJ8