Answers
Answer:
\(x = \frac{y - 4}{7}\)
Step-by-step explanation:
\(y = 7x + 4 \\\\y - 4 = 7x\\\\\frac{y - 4}{7} = \frac{7x}{7}\\ \\ \frac{y - 4}{7} = x\)
Related Questions
what is the long division work of the problem of 337 divided by 2.
Answers
Step-by-step explanation:
168 <-- quotient --- 337 2 - 13 12 -- 17 16 -- 1 <-- remainder
Answer:
168.5
Step-by-step explanation:
₁ ₁ ₁
2 ) 3 3 7. 0
1 6 8.5 <--- Quoitient
first part is 2 divide once into 3 with remainder 1
- and this 1 goes in front of the 3 to give 13.
2 into 13 goes 6 times with remainder 1
- then we get 2 into 17 giving 8 remainder 1 and so on....
ILL GIVE BRAINLY
Zeros placed on the right
of the last
non-zero digit after the decinal point
Answers
Answer:
• All non-zero digits in a number are significant.
Example: Numbers 0.0000216, 0.0216, 21.6 and 216 have the same number of significant figures namely three (2, 1, 6).
• All zeros between two non-zero digits are significant, no matter where the decimal point is, if at all.
Example : In the numbers 0.0000206, 0.0206, 20.6 and 206, the zero lying between the digits 2 and 6 is only significant.
• If the number is less than 1, the zeroes on the right of decimal point but to the left of the first non-zero digit are not significant.
Example : In 0.0000206, the four zeros after decimal and before the digit 2 has no significance. Similarly, in 0.0206, the zero after decimal and before the digit 2 has no significance. So the number of significant figures of these numbers are three (2, 0 and 6).
Hope this helps, have a nice day/night! :D
The accompanying technology output was obtained by using the paired data consisting of foot lengths (cm) and heights (cm) of a sample of 40 people. Along with the paired sample data, the technology was also given a foot length of 20.4cm to be used for predicting height. The technology found that there is a linear correlation between height and foot length. If someone has a foot length of 20.4 cm, what is the single value that is the best-predicted height for that person?
Answers
The best-predicted height for someone with a foot length of 20.4 cm is approximately 65.83 cm.
To find the best-predicted height for someone with a foot length of 20.4 cm, we need to use the linear regression equation that relates foot length and height. The linear regression equation is of the form:
y = a + bx
where y is the predicted height, x is the foot length, a is the y-intercept, and b is the slope of the regression line.
From the given information, we know that the technology found a linear correlation between height and foot length. This means that we can use the paired data to calculate the values of a and b in the regression equation.
Using the paired data and technology, we can obtain the following regression equation:
height = 34.774 + 1.4966 (foot length)
Now we can substitute the given foot length of 20.4 cm into the equation to obtain the predicted height:
height = 34.774 + 1.4966 (20.4)
height = 65.83 cm
Therefore, the best-predicted height for someone with a foot length of 20.4 cm is approximately 65.83 cm.
Know more about linear regression here:
https://brainly.com/question/29665935
#SPJ11
What is the area of this figure?

Answers
Answer:
75.398
Step-by-step explanation:
0,2,4,6,8 write a function f(n) that represents the nth term of the sequence, where f(1)=0
Answers
The function f(n) = 2n – 2 represents the nth term of the sequence 0, 2, 4, 6, 8.
What is sequence?
In mathematics, a sequence is an ordered list of numbers, usually written in a specific pattern. Each number in the sequence is called a term, and the position of a term in the sequence is called its index or subscript.
A function f(n) that represents the nth term of the sequence given above can be written as follows:
f(n) = 2n – 2
This function can be explained as follows:
The function f(n) represents the nth term of the given sequence. The first term of the sequence is 0, which is equal to f(1). The second term of the sequence is 2, which is equal to f(2). This pattern can be seen throughout the sequence, as each subsequent term is two more than the preceding one. Thus, the general form of the function can be written as f(n) = 2n – 2, where n represents the nth term of the sequence.
For example, when n = 5, the function f(n) = 2n – 2 = 10. This is equal to the fifth term of the sequence, 8.
Therefore, the function f(n) = 2n – 2 represents the nth term of the sequence 0, 2, 4, 6, 8.
To know more about sequence click-
https://brainly.com/question/7882626
#SPJ1
The denominator of a fraction is 7 more than the numerator. If both the numerator and the denominator are decreased by 3, the resulting fraction is equivalent to 5/12. Find the fraction.
Answers
Answer: The original fraction is 8/15
Step-by-step explanation:
The part about the 7 more than the numerator is not needed. All you have to do is add 3 to the numerator and 3 to the denominator.
5+3=8
&
12+3=15
So
8/15
I hope this helps!
How many Arithmetic means must be inserted between 1 and 36 so that the
sum of the resulting arithmetic progression shall be 148 ?
Answers
The Arithmetic means will be inserted between 1 and 36 such that the
sum of the resultant arithmetic progression will be 148 is 8.
What is defined as arithmetic progression?The distinction between the 2 arithmetic orders is a constant value in Arithmetic Progression (AP). Another name for it is Arithmetic Sequence.
We'd arrive along all a few key words in AP, which were denoted as:
The first term (a)Common difference (d)Term nth (an)The total of first n terms (Sn)The AP can also be defined in terms of common distinctions, as illustrated below.
The method of calculating an AP's n-th term is as follows: an = a + (n − 1) × dThe following is the arithmetic progression sum: Sn = n/2[2a + (n − 1) × d].Common difference 'd' of an AP: d = a2 - a1 = a3 - a2 = a4 - a3 = ...... = an - an-1.Now, for the stated question;
Assume the first term is'a₁' = 1.
The last term of the series that is nth term is 'a₃₆'.
The sum of all digits between 1 and 36 is given as 148.
Thus, Sn = 148
Substitute the value in the sum formula.
Sn = (n/2)×(a₁ + a₃₆)
148 = (n/2)×(1 + 36)
148 = (n/2)×(37)
n = (148×2)/37
n = 8.
Thus, the total number of Arithmetic means that can be inserted between 1 and 36 is 8.
More information on the arithmetic sequence can be found here.
brainly.com/question/6561461
#SPJ9
Calculate the distance between the points M=(−6, 1) and F = (1, −7) in the coordinate plane.
Answers
Answer:
let M point x1, Y1 and f point X2,y2 then we know the formula of distance root (x2-x1)^2+(y2-y1)^2 then you will found the distance
equation 3x+y=0. Plot the theme points that are in the solution wet, drow a line
through the three points and then answer the questions below
(1,7) (-8,4) (-2,6) (-5,5) (2,-6) (3,-9)
Answers
Step-by-step explanation:
The equation 3x + y = 0 can be rearranged to y = -3x. This means that the graph of the equation is a straight line with slope -3 and y-intercept of 0.
The three points that are in the solution set are (-2,6), (-5,5), and (3,-9). Plotting these points on a coordinate plane and connecting them with a straight line, we can see that they all lie on the same line with slope -3:
[Graph not shown here, but a straight line with three points (-2, 6), (-5, 5), (3, -9) can be plotted with a slope of -3 and y-intercept of 0.]
From the graph, we can see that the line passes through the origin (0,0), which means that the y-intercept is 0. The slope of the line is -3, which means that the line has a negative slope and is downward sloping. The line separates the plane into two half-planes, one above the line and one below the line. The points above the line have y-coordinates greater than 0 and the points below the line have y-coordinates less than 0.
the unit rate for each option. 9. Option A: $15.40 for 3.5 lbs of gummy bears
Answers
The unit rate for Option A is $4.4 per pound of gummy bears.
The unit rate for each option is a mathematical expression that compares two quantities of different measures by dividing one quantity by the other.
The unit rate is used to determine how much an item costs per unit of measure.
To find the unit rate for each option, we can use the following formula: Unit rate = price ÷ weight Option A: $15.40 for 3.5 lbs of gummy bears Unit rate = 15.40 ÷ 3.5Unit rate = $4.4 per pound of gummy bears.
Therefore, the unit rate for Option A is $4.4 per pound of gummy bears.
For more such questions on gummy bears
https://brainly.com/question/25831000
#SPJ8
Here is the complete question given below:
The unit rate for each option. 9. Option A: $15.40 for 3.5 lbs of gummy bears. $7.65 for 1.8 Ibs of gummy bears.
50 POINTS AND BRAINILEST!!!!!!!!!!!!!!!!
Stanley runs, swims, and bikes every day. During these workouts, he runs at 9 mph, bikes at 16 mph, swims at 2.5 mph. Yesterday, he ran for half an hour longer than he swan, and his biking time was twice his running time. How long did Stanley run, swim, and bike yesterday if the total distance he covered was 64 miles. What distance did Stanley cover while swimming?
Answers
Answer: 3x-x+2=4
Step-by-step explanation:
You can put this solution on YOUR website!
running distance is 9(t3+0.5)
biking distance is 16 (2(t3+0.5))=16(2t3+1)
swimming distance is 2.5t3
9t3+4.5+32t3+16+2.5t3=64
43.5t3=43.5
t3=1 hour
He biked for 3 hours (48 miles)
He ran for 1.5 hours (13.5 miles)
He swam for 1 hour (2.5 miles)
He covered 62 miles in 5.5 hours of activity.
Answer:
He swam for 1 hour (2.5 miles)
Step-by-step explanation:
The relation between time, speed, and distance is,
distance = speed × time
Here is a picture of some sea animals. The number line on the left shows the vertical position of each animal above or below sea level, in meters.
1. How far above or below sea level is each animal? Measure to their eye level.
2. A mobula ray is 3 meters above the surface of the ocean. How does its vertical position compare to the
height or depth of:
The jumping dolphin?
The flying seagull?
The octopus?
3. An albatross is 5 meters above the surface of the ocean. How does its vertical position compare to the
height or depth of:
The jumping dolphin?
The flying seagull?
The octopus?
4. A clownfish is 2 meters below the surface of the ocean. How does its vertical position compare to the
height or depth of:
The jumping dolphin?
The flying seagull?
The octopus?
5. The vertical distance of a new dolphin from the dolphin in the picture is 3 meters. What is its distance from the surface of the ocean?

Answers
Answer:
How far above or below sea level is each animal? Measure to their eye level.The jumping dolphin is approx. 6 meters under sea level
The flying seagull is approx. 8 meters under sea level
The mobula ray is approx. 2 meters under sea level
The clownfish is approx.t 4 meters under sea level
The octopus is approx. 8 meters under sea level
A mobula ray is 3 meters above the surface of the ocean. How does its vertical position compare to the height or depth of:The jumping dolphin: The mobula ray is 9 meters lower than the jumping dolphin.
The flying seagull: The mobula ray is 11 meters lower than the flying seagull.
The octopus: The mobula ray is 11 meters higher than the octopus.
An albatross is 5 meters above the surface of the ocean. How does its vertical position compare to the height or depth of:The jumping dolphin: The albatross is 1 meter lower than the jumping dolphin.
The flying seagull: The albatross is 3 meters lower than the flying seagull.
The octopus: The albatross is 13 meters higher than the octopus.
A clownfish is 2 meters below the surface of the ocean. How does its vertical position compare to the height or depth of:The jumping dolphin: The clownfish is 8 meters lower than the jumping dolphin.
The flying seagull: The clownfish is 10 meters lower than the flying seagull.
The octopus: The clownfish is 6 meters higher than the octopus.
The vertical distance of a new dolphin from the dolphin in the picture is 3 meters. What is its distance from the surface of the ocean?If the new dolphin is above the dolphin in the picture, then its distance from the surface of the ocean is 6 - 3 = 3 meters.
If the new dolphin is below the dolphin in the picture, then its distance from the surface of the ocean is 6 + 3 = 9 meters.
✧☆*: .。. Hope this helps, happy learning! (✧×✧) .。.:*☆✧
4) Students in Ms. Udon
science class planted 13
conifers in different places
around the school yard. They
measured the heights (in inches)
of the conifers one month after
planting them. How many
conifers are greater than 6 1/4
inches tall but less than 8 1/2
inches tall?
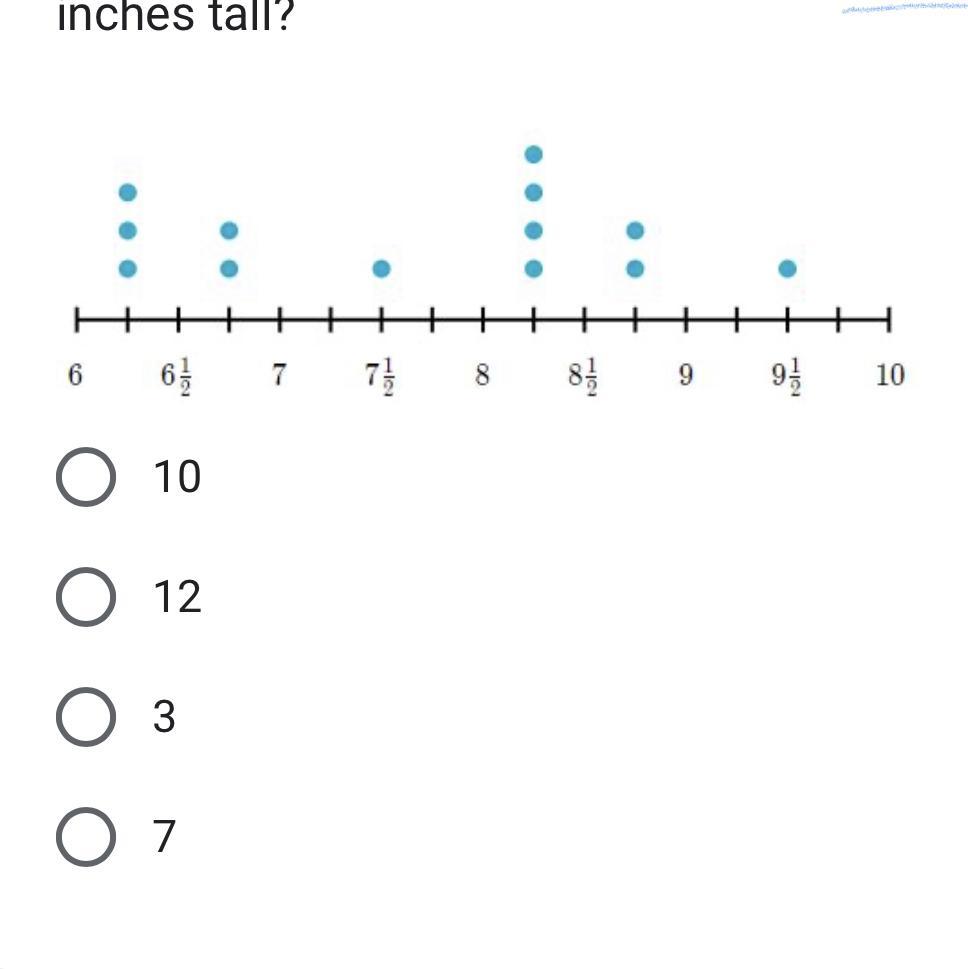
Answers
Considering the dot plot, the tallest conifer is 3.25 inches taller than the shortest conifer.
This shows, with dots, the number of times that each of the measures appears in a data set.
For finding the dot plot, we have that:
The shortest conifer measures 6 and 1/4
= 6.25 inches.
The tallest conifer measures 9.5 inches.
The difference is:
9.5 - 6.25 = 3.25 inches.
Therefore the tallest conifer is 3.25 inches taller than the shortest conifer.
More can be learned about a dot plot at;
brainly.com/question/24912483
#SPJ1
On Monday, Mia spent 3 1/2 hours studying. On Tuesday she spent another 1 3/5 hours studying. What is the total time she spent studying?
pls help me, srry not college
Answers
Answer:
5 1/10
Step-by-step explanation:
let me know if you need an explanation
If Brianna can read 1/4 of her book in 1/2 hour, how much of her book will she read in 1 hour?
Answers
if you guys can make it out can you help me find the length of the small right triangle? sorry its really bad drawn

Answers
Answer:
32.0
Step-by-step explanation:
i really can't explain it but comment if need more help
and can u take better picture
Julie has 300ml of juice, She gives of the juice away.
How much juice does she give away?
Answers
Answer: Up to 300 ml because that is all she has in total. I think the question is missing parts.
Step-by-step explanation:
c) I use the 4 triangles to make a trapezium. What is the area of the trapezium?
Answers
Answer:
1/2=10
Step-by-step explanation:
it depends upon the area of the triwngle
According to a report from the United States Environmental Protection Agency, burning one gallon of gasoline typically emits about
8.9
kg
8.9 kg8, point, 9, start text, space, k, g, end text of
CO
2
CO
2
start text, C, O, end text, start subscript, 2, end subscript. A fuel company wants to test a new type of gasoline designed to have lower
CO
2
CO
2
start text, C, O, end text, start subscript, 2, end subscript emissions. Their hypotheses are
�
0
:
�
=
8.9
kg
H
0
:μ=8.9 kgH, start subscript, 0, end subscript, colon, mu, equals, 8, point, 9, start text, space, k, g, end text and
�
a
:
�
<
8.9
kg
H
a
:μ<8.9 kgH, start subscript, start text, a, end text, end subscript, colon, mu, is less than, 8, point, 9, start text, space, k, g, end text, where
�
μmu is the mean amount of
CO
2
CO
2
start text, C, O, end text, start subscript, 2, end subscript emitted by burning one gallon of this new gasoline.
Suppose that
�
0
H
0
H, start subscript, 0, end subscript is actually true.
Which situation below would have the lowest probability of a Type I error?
Answers
The option that would be a Type II error in this setting is B. The mean amount of CO2 emitted by the new fuel is actually lower than 89 kg but they fall to conclude it is lower than 89 kg
What is the error about?The type 1 error occurs if we get false positive conclusion (false positive means we accuse null hypothesis being wrong when it was actually correct).
The type 2 error occurs if we get false negative conclusion (false negative means we accept null hypothesis when it was actually false).
Hence, the mean amount of CO2 emitted by the new fuel is actually lower than 89 kg but they fall to conclude it is lower than 89 kg is a type II error.
Learn more about Type II error on
https://brainly.com/question/16012410
#SPJ1
According to a report from the United States Environmental Protection Agency, burning one gallon of gasoline typically emits about 8.9 kg of CO2. A fuel company wants to test a new type of gasoline designed to have lower CO2 emissions. Here are their hypotheses:
H0: μ = 8.9 kg
Ha: μ < 8.9 kg (where μ is the mean amount of CO2 emitted by burning one gallon of this new gasoline).
Which of the following would be a Type II error in this setting?
A. The mean amount of CO2 emitted by the new fuel is actually 89 kg but they conclude it is lower than 89 kg
B. The mean amount of CO2 emitted by the new fuel is actually lower than 89 kg but they fall to conclude it is lower than 89 kg
C. The mean amount of CO2 emitted by the new fuel is actual 89 kg and they alto conclude it is lower than 89 kg and they conclude it is lower than 9 kg
D. The mean amount of CO2 emitted by the new fuels actually lower than 8.9
given a circle with center (-5,3) and radius sqrt 17 what is the slope of the tangent line to the circle that passes through the point (-1,2)
Answers
The slope of the tangent line that passes through the point (-1, 2) is 4, and the equation of the line is: y - 2 = 4(x + 1) or y = 4x + 6
To find the slope of the tangent line to a circle, we first need to find the equation of the circle.
Given the center and radius, the equation of a circle can be written as:
(x + 5)^2 + (y - 3)^2 = 17
Next, we find the equation of the line that passes through the point (-1, 2) and is tangent to the circle.
We can use the slope-point form of the equation of a line:
y - 2 = m(x + 1)
where m is the slope of the line.
To find the value of m, we need to find the slope of the line that is tangent to the circle at the point (-1, 2).
The slope of a tangent line to a circle at a point is equal to the derivative of the equation of the circle at that point.
Taking the partial derivatives of x and y in the equation of the circle, we get:
2(x + 5)dx + 2(y - 3)dy = 0
Rearranging and solving for the slope:
dy/dx = -(x + 5)/(y - 3)
Evaluating the slope at the point (-1, 2), we get:
dy/dx = -(−1 + 5)/(2 − 3) = 4
For more questions on The slope of the tangent line
https://brainly.com/question/29299011
#SPJ4
what is 8 times 0.9 equal
Answers
Answer:
7.2
Step-by-step explanation:
Given m \| nm∥n, find the value of x. m n t (4x-18)° (3x-8)
Answers
Answer:
12x2
Step-by-step explanation:
The graph of a line goes through the points (-4,3) and (6,8). What is the equation of the line in slope-intercept form?
Enter the correct answer in the box by replacing m and b with the appropriate values.
음 (0) 미
Of
+
a, sin cos tan sin costan!
μ ρ Φ
CSC seccot log log. In
ΖΔ
Σ
U (0)
X
5
A
>
S
2
II
1
y = mx + b
I
Submit
Reset
Type here to search
73°F Mostly cloudy
327 PM
7/3/2001

Answers
Answer:
\(y=\frac{1}{2}x+5\)
Step-by-step explanation:
Hi there!
Slope intercept form: \(y=mx+b\) where m is the slope and b is the y-intercept (the value of y when x is 0)
1) Determine the slope (m)
\(m=\frac{y_2-y_1}{x_2-x_1}\) where two points that lie on the line are \((x_1,y_1)\) and \((x_2,y_2)\)
Plug the given points (-4,3) and (6,8) into the equation
\(m=\frac{8-3}{6-(-4)}\\m=\frac{8-3}{6+4}\\m=\frac{5}{10}\\m=\frac{1}{2}\)
Therefore, the slope of the line is \(\frac{1}{2}\). Plug this into \(y=mx+b\) :
\(y=\frac{1}{2}x+b\)
2) Determine the y-intercept (b)
\(y=\frac{1}{2}x+b\)
Plug in one of the given points and solve for b
\(8=\frac{1}{2}(6)+b\\8=3+b\)
Subtract 3 from both sides to isolate b
\(8-3=3+b-3\\5=b\)
Therefore, the y-intercept is 5. Plug this back into \(y=\frac{1}{2}x+b\):
\(y=\frac{1}{2}x+5\)
I hope this helps!
Pls help I’m failing

Answers
Answer:
(172-45)x2=n
Step-by-step explanation:
(172-45)x2=n
Answer:
(172-45)×2=n
n= 254
...........
Helppp
The half-life of Radium-226 is 1590 years. If a sample contains 500 mg, how many mg will remain after 1000 years?---------
Answers
Using the exponential decay equation, we can see that after 1000 years we will have 178.43 mg of Ra₂₂₆
How much will remain after 1000 years?The decay of a radioactive substance, such as Radium-226, can be modeled by the exponential decay equation:
N(t) = N₀ * (1/2)^(t / T)
where:
N(t) is the amount of the substance remaining after time t
N₀ is the initial amount of the substance
t is the time elapsed
T is the half-life of the substance
Given that the half-life of Radium-226 is 1590 years and the initial amount is 500 mg, we can plug in these values into the equation and solve for N(1000), which represents the amount remaining after 1000 years.
N(1000) = 500 * (1/2)^(1000 / 1590)
N(1000) ≈ 178.43 mg
Learn more about half life at:
https://brainly.com/question/11152793
#SPJ1
FILL IN THE BLANK eigenvalues and eigenvectors of a matrix (optional) 0 points possible (ungraded) let , ____ and ____, where unanswered_____- , where unanswered . therefore, is an eigenvector of with eigenvalue , and is an eigenvector of with eigenvalue .
Answers
Let A be a matrix, v and λ be vectors. Therefore, Av = λv, where v is an eigenvector of A with eigenvalue λ, and if there is another non-zero vector w such that Aw = μw, then w is an eigenvector of A with eigenvalue μ. Therefore, v is an eigenvector of A with eigenvalue λ, and w is an eigenvector of A with eigenvalue μ.
Allow a to be a matrix, and v and λ to be vectors. Consequently, av = λv, wherein v is a non-zero vector and λ is a scalar. This means that v is an eigenvector of a with eigenvalue λ.
Further, if there may be every other non-0 vector w such that aw = μw, then w is an eigenvector of a with eigenvalue μ. The eigenvectors and eigenvalues of a matrix are vital in lots of packages, including fixing systems of linear equations, studying dynamical systems, and studying facts using strategies along with main thing evaluation.
By computing the eigenvectors and eigenvalues of a matrix, we will benefit perception of its shape and conduct, and use these records to solve problems and make predictions.
Learn more about eigenvalue:
brainly.com/question/29749542
#SPJ4
Suppose y varies directly as x. If x = 24 when y = 8, then what is the constant of variation
Answers
Direct variation is represented by the formula \(\frac{y}{x} = k\)
where k is the constant of variation.
So here, since we know that x = 24 and y = 8,
the constant of variation must be equal to 8/24.
if we write 8/24 in lowest terms, we have 1/3.
So 1/3 = k.
Use the factor theorem to determine if the given binomial is a factor of f (x).
f(x) = x4 + 8x3 + 11x2 -11x + 3; (x + 3)
Answers
Answer:
The binomial is a factor
Step-by-step explanation:
In order to show that x+3 is a factor of the polynomial, then;
f(a) = 0
Get a by equating x+3 to zero
x+3 = 0
x = -3
Get f(-3)
f(-3) = (-3)^4 + 8(-3)^3 + 11(-3)^2 -11(-3) + 3
f(-3) = 81 - 216 + 99 + 33 + 3
f(-3) = 216-216
f(-3) = 0
Since the remainder of the polynomial when divided by x+3 is zero, this shows that x+3 is a factor of the given polynomial
3 to 2 if there are 120 children who like pizza, how many total
Answers
Answer:
320
Step-by-step explanation:
Is the 3 to 2 part to part? Out of every 5 people, 3 prefer pizza and 2 do not. We can us this to set up a proportion.
3/5 = 120/x The 3 represent the part that like pizza over the whole of 5. The second ratio is showing the actual number of people that liked pizza over the total number of people.
What would be multiply 3 by to get 120? 40. So, we will multiply 5 times 40 to get the total number of people. 5 x 40 = 200
what does d equal in this equation
3.5(4.4 + d) = 28
Answers
Answer:
d= 3.6 harrryuuyyyyyytrrrrttppwkkkk
