Numerical Approximation Methods with Integrals - 12th Grade Calculus Please help me, thanks!

Answer:

\(\frac{x}{y} \frac{5x}{\frac{x^{2} -4}{\frac{8x}{x+2} } }\\\\=\frac{5x(x+2)}{8x(x^{2} -4)}\\\\=\frac{5(x+2)}{8(x+2)(x-2)}\\\\=\frac{5}{8(x-2)}\\\\=\frac{5}{8}.\frac{1}{x-2}\)

Step-by-step explanation:

Step-by-step explanation:

\( \frac{ \frac{5x}{ {x}^{2} - 4 } }{ \frac{8x}{x + 2} } = \frac{5x}{ {x}^{2} - 4 } \times \frac{x + 2}{8x} = \frac{5x + 10}{ {8x}^{2} - 32} \\ \)

hope it helps:)

Answer:

Choice B is the closest

Step-by-step explanation:

A-lateral = 2πrh

r = D/2 = 16.5/2 = 8.25

h = 14

A = 2π(8.25)(14) = 725.7 in²

The range of the function y = 1/2 x + 2 is {0, 1, 2}, if the domain of the function is {-4, -2, 0}.

An expression, rule, or law in mathematics that specifies the relationship between an independent variable and a dependent variable.

The given function is,

y = 1/2 x + 2.

Also, the domain of the function is {-4, -2, 0}.

Since, the domain of the functions defines the values of x,

And range defines the value of y in function.

The value of y at x = -4

y = 1/2(-4) + 2 = -2 + 2 = 0

At x = -2,

y = 1/2(-2) + 2 = -1 + 2 = 1

At x= 0,

y = 1/2 (0) + 2 = 2

The values of y are 0, 1 and 2.

Hence, the range of the function is {0, 1, 2}.

To know more about Function on:

https://brainly.com/question/2411204

#SPJ1

Answer:

Step-by-step explanation:

E. To find the equation of the perpendicular bisector of the segment with endpoints A(-2,8) and B(-4,-6), we need to follow these steps:

Find the midpoint of the segment AB using the midpoint formula:

Midpoint = [ (x1 + x2)/2 , (y1 + y2)/2 ]

Midpoint = [ (-2 - 4)/2 , (8 - 6)/2 ]

Midpoint = [ -3 , 1 ]

Therefore, the midpoint of AB is (-3, 1).

Find the slope of the line passing through the points A and B using the slope formula:

Slope = (y2 - y1) / (x2 - x1)

Slope = (-6 - 8) / (-4 - (-2))

Slope = (-14) / (-2)

Slope = 7

Since we need the slope of the perpendicular bisector, we can use the fact that the product of the slopes of two perpendicular lines is -1. Therefore, the slope of the perpendicular bisector is the negative reciprocal of the slope of AB:

Slope of perpendicular bisector = -1/7

Finally, we can use the point-slope form of a line to write the equation of the perpendicular bisector with slope -1/7 and passing through the midpoint (-3, 1):

y - y1 = m(x - x1)

where m is the slope and (x1, y1) is a point on the line.

Substituting m = -1/7 and (x1, y1) = (-3, 1), we get:

y - 1 = (-1/7)(x - (-3))

Simplifying, we get:

y - 1 = (-1/7)x - 3/7

y = (-1/7)x - 3/7 + 1

y = (-1/7)x + 4/7

Therefore, the equation of the perpendicular bisector of the segment with endpoints A(-2,8) and B(-4,-6) is y = (-1/7)x + 4/7

F. To find the perpendicular bisector of segment XY, we first need to find the midpoint of the segment. Using the midpoint formula, we get:

Midpoint = ( (x1+x2)/2 , (y1+y2)/2 )

Midpoint = ( (5+7)/2 , (17+3)/2 )

Midpoint = ( 6 , 10 )

So the midpoint of segment XY is (6,10). Now we need to find the slope of segment XY. Using the slope formula, we get:

Slope of XY = (y2 - y1) / (x2 - x1)

Slope of XY = (3 - 17) / (7 - 5)

Slope of XY = -7

The perpendicular bisector of XY will have a slope of the negative reciprocal of the slope of XY. So the slope of the perpendicular bisector is:

Slope of perpendicular bisector = 1/7

Now we can use the point-slope form of a line to find the equation of the perpendicular bisector. Using the midpoint (6,10) and the slope 1/7, we get:

y - y1 = m(x - x1)

y - 10 = (1/7)(x - 6)

y - 10 = (1/7)x - 6/7

y = (1/7)x + 64/7

So the equation of the perpendicular bisector of segment XY is y = (1/7)x + 64/7.

Answer:

w=25/4=6.50

Step-by-step explanation:

Answer:

Subtract 5 as you go along the pattern.

82-5=77-5=72-5=67...

Step-by-step explanation:

Answer:

eeee

Step-by-step explanation:

eeeee

The perimeter of ABCD can be calculated using the following formula: Line AB + Line BC + Line CD + Line DA = Perimeter ABCD.

Therefore,

The perimeter of ABCD can be calculated using the following formula: Line AB + Line BC + Line CD + Line DA = Perimeter ABCD.

When we substitute the provided values, we get: ABCD perimeter = 9 units plus 5 units plus 9 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units plus 5 units As a result, the perimeter of ABCD is 28 units.

To learn more about Quadrilateral refer to:

https://brainly.com/question/23935806

#SPJ2

Answer:

  an infinite number

Step-by-step explanation:

This equation has a graph that is a line. Every point on the line is a solution.

There are an infinite number of solutions.

The surface area of the sphere, to the nearest whole number, is 1318 cm².

A sphere is simply a three-dimensional geometric object that is perfectly symmetrical in all directions.

The volume of a sphere is expressed as:

Volume =  (4/3)πr³

The surface area of a sphere is expressed as:

SA = 4πr²

Where r is the radius of the sphere and π is the mathematical constant pi.

Given that the volume V is 4500 cm³.

First, we solve for the radius:

Volume =  (4/3)πr³

4500 = (4/3)πr³

4500 × 3 = 4πr³

13500 = 4πr³

r³ = 13500/4π

r = ∛( 13500/4π )

Now that we have the radius, we can calculate the surface area of the sphere using the formula:

SA = 4πr²

Substituting the radius we found:

SA = 4 × π × ∛( 13500/4π )²

SA = 1318 cm²

Therefore, the surface area is approximately 1318 cm².

Learn more about volume of hemisphere here: brainly.com/question/22886594

#SPJ1

Given:

The sum of 7 over 6 and 5 over 8

To determine the sum of 7 over 6 and 5 over 8, we rewrite the given information first into:

Next, we note that the Least Common Denominator (LCD) of 6 and 8 is 24 and to make the denominators the same as the LCD:

Then, simplify:

Therefore, the answer is 43/24.

The equation of the line is: y = 0.5x + 4 and the inequality is H > 74.25

To find the equation of a line that passes through two points, we need to use the slope-intercept form of a linear equation:

y = mx + b

The slope is calculated as

m = (y2 - y1) / (x2 - x1)

m = (11 - 4) / (14 - 0)

m = 7/14

m = 1/2

m = 0.5

Next, we have

y = mx + b

4 = 0.5(0) + b

b = 4

So the equation of the line is: y = 0.5x + 4

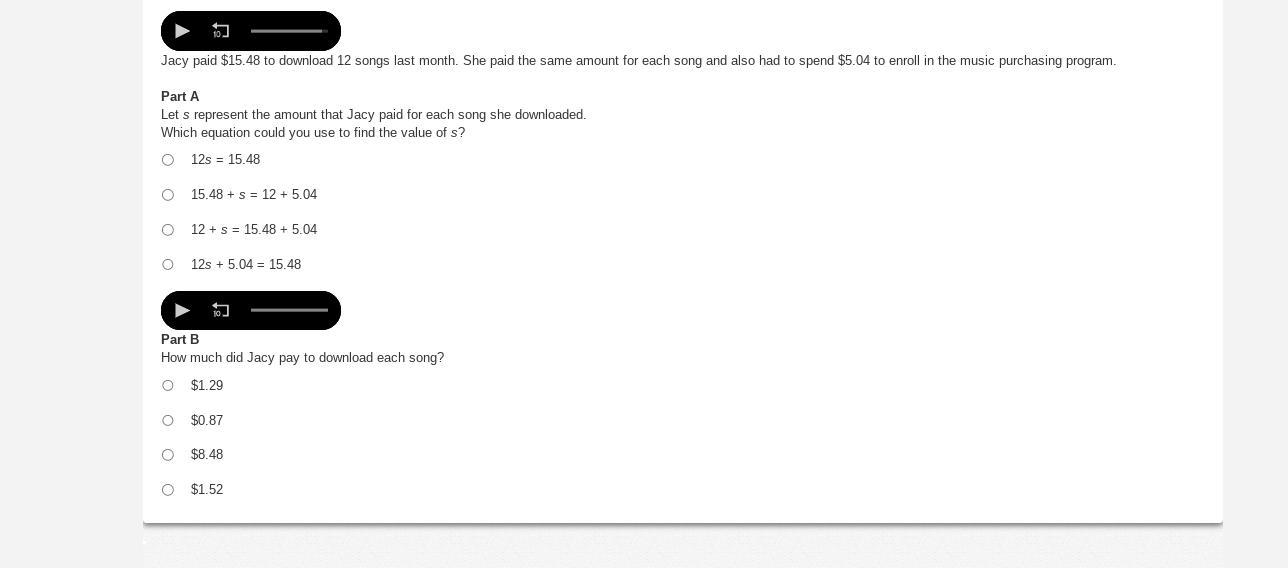
Part A: The equation that could be used to find the value of s is:

12s + 5.04 = 15.48

To find the value of s, we need to isolate the variable on one side of the equation.

We can do this by subtracting 5.04 from both sides and then dividing both sides by 12:

12s + 5.04 - 5.04 = 15.48 - 5.04

12s = 10.44

s = 10.44/12

s = 0.87

Therefore, Jacy paid $0.87 to download each song.

To represent the number of hours, h, that Tara plans to work this month as an inequality, we can use the fact that she plans to work more hours than last month.

Let H be the total number of hours that Tara works this month.

Then we can write:

H > 16.5 + 19 + 23 + 15.75

Simplifying the right-hand side, we get:

H > 74.25

Therefore, the inequality that represents the number of hours, h, Tara plans to work this month is: H > 74.25

Read more about linear relation and inequality at

https://brainly.com/question/30318449

https://brainly.com/question/30390162

#SPJ1

Answer:

2.8

Step-by-step explanation:

x2.3, x2.4, 2.8, x2.9, x2.9

i is expression is equivalent to i233.

Given i233

We know that i2 = -1 , if we square (i2) we get (-1)2 = 1

So, if i is multiplied to (i2), we get i3 = (-1).i = -i

Again if i is multiplied to i3 , we get i4 = (-i).i = -i2 = -(-1) = 1

Hence, all even powers of i after 2, will give answer as 1

The sequence will continue as a cycle as i, -1, -i, 1

So, i233 can be rewritten as i232 + 1= i232.i

i233 = (i2)116 .i = (-1)116 . i

Since the power 116 is even , the term becomes 1.i = i

Therefore, i233 = i

To learn more about mathematical expression refer to:

https://brainly.com/question/4344214

#SPJ13

Answer:

⅓

\( \frac{5}{9} \times \frac{3}{5} \)

\( = \frac{1}{3} \times \frac{1}{1} \)

\( = \frac{1}{3} \)

\( \frac{1}{3} \div \frac{3}{5} \)

\( = \frac{1}{3} \times \frac{5}{3} \)

\( = \frac{5}{9} \)

#CarryOnLearning

Hey there!

When you see the word “of” in mathematical statement, it usually means “multiply” (aka Multiplication)

“3/5 of 5/9”

3/5 • 5/9

MULTIPLY the NUMERATORS (TOP NUMBERS) from each other & also MULTIPLY the DENOMINATORS (BOTTOM NUMBERS) from each other!

3(5) / 5(9)

3(5) = 15 ⬅️ NUMERATOR

5(9) = 45 ⬅️ DENOMINATOR

15/45

The GCF (Greatest Common Factor) for both of your numbers is: 15.. so DIVIDE both the NUMERATOR and DENOMINATOR from 15

15 ÷ 15 / 45 ÷ 15

15 ÷ 15 = 1 ⬅️ NUMERATOR

45 ÷ 15 = 3 ⬅️ DENOMINATOR

Answer: 1/3 ☑️

Good luck on your assignment and enjoy your day!

~LoveYourselfFirst:)

Answer:

x = 4.33

Step-by-step explanation:

3x - 4 = 17

     -4 = -4

3x = 13

/3  = /3

x  = 4.33

4.33

could not delete my wrong answer sorry :/

Answer:

Expected value = 190

Variance = 4000

Step-by-step explanation:

Let X be the number of the trials until the third success of the bad pump.

This implies that X is a negative binomial distribution having θ = 20% = 0.2.

Now, if for example it will take X trials to use up the three pumps, then the total time is 10 min/trials + extra 10 minutes for the 3 bad pumps

This means the total time is written as;

T = 10X + (10 + 10 + 20)

T = 10X + 40

Mean which is also expected value of X is;

μ_x = 3/0.2 = 15

Variance of X is; σ²_x = 40

Thus;

Mean of T will be;

μ_T = 10μ_x + 40

μ_T = 10(15) + 40

μ_T = 190

Also, variance of T will be;

σ²_T = 10²•σ²_x

σ²_T = 100 × 40

σ²_T = 4000

When the converted costs are compared, the earlier edition of the history book is more expensive.

The consumer price index measures the changes in price of a basket of good. It is used to measure inflation.

Converted price of the earlier edition = (CPI in 1992 / CPI in 1999) x later edition of the book

(140.3 / 166.3) x $43 = $36.28

To learn more about, consumer price index, please check: https://brainly.com/question/26382640

#SPJ1

Answer:

A round pencil is sharpened at both ends the hight of the pencil is 16cm the length of the pencil is 1cm the radius of the two sharpened ends is 16cm 1.2cm

Calculate the volume using the value 22/7. 

Step-by-step explanation:

We have proven that the sum of the squares of the diagonals of a parallelogram is equal to the sum of squares of its sides.

Parallelograms are quadrilaterals with opposite sides parallel to each other. They have many interesting properties, one of which is the relationship between the sum of the squares of the diagonals and the sum of squares of their sides. In this explanation, we will prove this relationship mathematically.

Let us consider a parallelogram with diagonals AC and BD, and sides AB, BC, CD, and DA.

To begin, let us draw line segments from the midpoints of each side to the midpoint of the opposite side. This divides the parallelogram into four congruent triangles, as shown below:

We can now use the Pythagorean theorem to find the length of each line segment. Let us denote the midpoint of AB as M, the midpoint of BC as N, and the midpoint of CD as P. Then we have:

AM² = AB²/4 + BM²

BN² = BC²/4 + CN²

CP² = CD²/4 + DP²

DM² = DA²/4 + AM²

Note that the line segments AM, BN, CP, and DM are half the length of the diagonals AC and BD. We can now add all these equations together:

=> AM² + BN² + CP² + DM² = (AB² + BC² + CD² + DA²)/4 + (AC² + BD²)/4

Rearranging this equation gives us:

=> AC² + BD² = 2(AM² + BN² + CP² + DM²) - (AB² + BC² + CD² + DA²)

Now, recall that the four triangles we divided the parallelogram into are congruent. Therefore, AM = BN = CP = DM, and AB = CD, BC = DA. Substituting these equalities into the above equation, we get:

=> AC² + BD² = 4(AM² + BN²) - 4(AB² + BC²)

We can further simplify this expression using the fact that the diagonals of a parallelogram bisect each other. Therefore, AM = CP and BN = DM. Substituting these equalities gives us:

AC² + BD² = 4(AM² + BN²) - 4(AB² + BC²)

= 4(AM² + BN² - AB² - BC²)

= 4(AN² + BM²)

Recall that AN and BM are half the length of the sides of the parallelogram. Therefore, we can write:

=> AC² + BD² = 4(AN² + BM²) = 4(AB² + BC² + CD² + DA²)

This completes the proof. We have shown that the sum of the squares of the diagonals of a parallelogram is equal to the sum of squares of its sides.

To know more about parallelogram here.

https://brainly.com/question/29147156

#SPJ4

Answer:

y = 5x -1/3

Step-by-step explanation:

The slope equation form of a line is

y = mx+b where m is the slope and b is the y intercept

y = 5x -1/3

Answer:

Option C

Step-by-step explanation:

In this case, an exponential function is a function that has common ratio whereas it is called a linear function if it has common difference.

Option A:

Since the values of y in the given table have different ratio but common difference, therefore it is not a a linear and not an exponential function. Option A is is not correct.

Option B:

Since the values of y in the given table have different ratio but common difference, therefore it is not a a linear and not an exponential function. Option B is not correct.

In option C.

Since the given values of y in the table have a common ratio, it is said to be an exponential function.

Option C is correct.

In option D.

Since the values of y in the given table have different ratio but common difference, therefore it is not a a linear and not an exponential function. Option D is incorrect.

The relationship between pints and cups where p represents pints and n represents cups is n = 2p.

We have,

3 cups converts to 1.5 pints

and 5 cups converts to 2.5 pints.

We know from constant of proportionality

y = kx

k = y/x

Put y= 2.5 and x= 5

Then, k = 5/2.5

k = 2

So, the relationship between pints and cups where p represents pints and n represents cups is

n = 2p

Learn more about Equation here:

https://brainly.com/question/29538993

#SPJ1

The correct option regarding the number of bags filled by Nina, using proportions, is given as follows:

Between 20 and 30 bags.

A proportion is a fraction of an amount, and this fraction is combined with the unit rates of the problem and basic arithmetic operations, especially multiplication and division, to find the desired amounts in the context of the problem.

In this problem, a proportion is applied to find the number of bags filled by Nina, which is given by the division presented as follows:

Number of bags = Number of treats / Treats per bag.

The values of these parameters are given as follows:

Hence the numeric value of the expression is:

Number of bags = 78/3 = 26.

Which is a number between 20 and 30.

The problem states that Nina had 78 treats, and each bag has 3 treats, then it asks the correct option regarding the number of bags.

More can be learned about proportions at https://brainly.com/question/24372153

#SPJ1

Answer: y = -12x +20

Step-by-step explanation: 1. distribute the -12 into the (x-2), that equals -12+24

2. subtract the 4 from the left side to move it to the right side of the equation

3. add like terms, so 24 + (-4) or 24-4 which equals 20.

4. Answer: y=-12x+20

Answer:

9.18%

Step-by-step explanation:

The probability of getting a tail on a fair coin flip is 0.5, and the probability of getting a head is also 0.5. Since each coin flip is independent, we can use the binomial probability formula to calculate the probability of getting exactly 5 tails in 15 flips:

P(X = 5) = (15 choose 5) * (0.5)^5 * (0.5)^(15-5)

where (15 choose 5) is the number of ways to choose 5 tails out of 15 flips, which can be calculated as:

(15 choose 5) = 15! / (5! * 10!) = 3003

Substituting the values, we get:

P(X = 5) = 3003 * (0.5)^15 ≈ 0.0918

Therefore, the probability of getting exactly 5 tails in 15 coin flips is approximately 0.0918 or 9.18%.

Answer:

$37.52

Step-by-step explanation: