ok so im really confused and if you can explain with work would be great. (no pictures because i can't see them)

Answers
Answer:
Y=3x
so the answer is 27 miles
Step-by-step explanation:
3 is the slope
Related Questions
The area of a rectangle is 60 cm². The length is 11 cm more than the width. Find the width
Answers
Answer:
5
Step-by-step explanation:
let width be x
and because length is 11 times more than the width, therefore length equals to 11x
hence the equation equals to
area = length x width
60 = 11x + x
60 = 12x
x = 60/12
therefore, the width of the rectangle is 5
The position of a particle moving along the x axis is given by x(t) = 5.42 m-2.31 m/s t. at what time in s does the particle cross the origin?
Answers
The particle crosses the origin at a time of 2.346 seconds.
To find the time at which the particle crosses the origin, we need to determine the value of t when x(t) is equal to zero, since the particle will be at the origin when its position is zero.
Using the given position function x(t) = 5.42 m - 2.31 m/s t, we can set x(t) equal to zero and solve for t:
x(t) = 0
5.42 m - 2.31 m/s t = 0
Subtracting 5.42 m from both sides, we get:
-2.31 m/s t = -5.42 m
Dividing both sides by -2.31 m/s, we get:
t = 5.42 m / 2.31 m/s
t = 2.346 s
Therefore, the particle crosses the origin at a time of 2.346 seconds.
In summary, we find the time at which the particle crosses the origin by setting the position function equal to zero and solving for the corresponding value of t. This method works for any one-dimensional motion along a straight line.
Learn more about "position function" : https://brainly.com/question/28939258
#SPJ11
Simplify without the use of calculator
\(\frac{\cos(-225)\sin(135)+\sin330}{\tan225}\)
Answers
\( \implies\quad \sf { \dfrac{cos(-225).sin(135)+sin330}{tan225}}\)
\( \implies\quad \sf {\dfrac{cos(225)-sin(180-45)+sin(360-30)}{tan(180+45)} }\)
\( \implies\quad \sf {\dfrac{cos(180+45).sin45-sin30}{tan45} }\)
\( \implies\quad \sf { \dfrac{-cos45.sin45-sin30}{tan45}}\)
\( \implies\quad \sf { \dfrac{-\dfrac{\sqrt{2}}{2}\times \dfrac{\sqrt{2}}{2}-\dfrac{1}{2}}{1}}\)
\( \implies\quad \sf {\dfrac{-\dfrac{2}{4}-\dfrac{1}{2}}{1} }\)
\( \implies\quad \sf {\dfrac{-2-2}{4} }\)
\( \implies\quad \sf { -\cancel{\dfrac{4}{4}}}\)
\( \implies\quad\underline{\underline{\pmb{ \sf {-1 }}}}\)
Malia is playing a target game with her friends. Each player throws a bean bag times toward a target on the ground. The distance between the bean bag and the target is recorded for each throw. The player with the lowest mean distance of all throws wins the game. The list shows the recorded distance, in , of Malia's first throws. , , , , , , Malia is the last player in the game. Her mean distance must be less than to win the game. What is the minimum distance, in , between the bean bag and the target that Malia needs on her last throw to win the game? Enter the answer in the box.
Answers
The minimum distance that Malia needs on her last throw to win the game is less than 2.12 meters.
How to determine distanceIn this target game, Malia needs to have the lowest mean distance of all throws to win. The mean distance is calculated by adding up all the distances and dividing by the number of throws.
First, we need to calculate the mean distance of Malia's first 7 throws.
Mean distance = (2 + 3 + 4 + 2 + 5 + 3 + 4) / 7 = 3.14
Now, we know that Malia's mean distance must be less than 3.14 to win the game. Let's call the distance of her last throw x.
The mean distance of all 8 throws will be: (2 + 3 + 4 + 2 + 5 + 3 + 4 + x) / 8
To find the minimum distance that Malia needs on her last throw to win the game, we can set up an inequality:
(2 + 3 + 4 + 2 + 5 + 3 + 4 + x) / 8 < 3.14
Multiplying both sides by 8 gives us:
2 + 3 + 4 + 2 + 5 + 3 + 4 + x < 25.12
Simplifying gives us:
x < 25.12 - 23
x < 2.12
Therefore, the minimum distance that Malia needs on her last throw to win the game is less than 2.12 meters.
Learn more about distance at https://brainly.com/question/15172156
#SPJ11
Find the mABC
Find the mEBD
Find the mABE can someone help me plz

Answers
Answer:
mABC=130°
mEBD=130°
mABE=50°
Step-by-step explanation:
Given your explanation in question two, justify why the sum of the measures of ∠b , ∠e , and ∠f must equal 180∘ .
Answers
Two triangles are a square ,One Square Be 360º, Half of the square is 180º
Will give brainliest plz help!

Answers
Answer:
6.28cm
Step-by-step explanation:
I think for me the easiest would be to find the circumference and divide by 4, since the arc is 1/4 of the circumference of the circle.
C=2πr
C=2(3.14)(4)
C = 25.12cm
25.12cm/4 = 6.28
After surveying students in her homeroom, katie decided to use a number cube to predict the probability that a randomly chosen student in her school planned to stay after school today for extracurricular activities. in katie’s simulation, a roll of 1 represents staying after school, while rolls of 2, 3, 4, 5, or 6 represent leaving school at the end of classes. according to katie’s model, what is the probability that three randomly chosen students will all stay after school for extracurricular activities today? startfraction 1 over 729 endfraction startfraction 1 over 216 endfraction startfraction 1 over 18 endfraction startfraction 1 over 6 endfraction
Answers
Using it's concept, it is found that the probability that three randomly chosen students will all stay after school for extracurricular activities today is of \(\frac{1}{216}\).
What is a probability?A probability is given by the number of desired outcomes divided by the number of total outcomes.
In this problem, for each student, one out of six rolls represent staying after school, hence there is a \(\frac{1}{6}\) probability they stay after school. For the three students, the probability is given by:
\(p = \left(\frac{1}{6}\right)^3 = \frac{1}{216}\)
More can be learned about probabilities at https://brainly.com/question/14398287
#SPJ4
Sammy's bedroom has the dimensions 9 feet by 15 feet. If the ceiling is 11 feet, what is the area of the smallest wall?
Answers
Answer:
99
Step-by-step explanation:
11 and 9 are the smallest dimensions. Multiply them and you get 99.
solve for x Express your answer as an integers or in simplest radical form 1-x^3=9
Answers
Answer:
\(\large\boxed{\tt x = 2}\)
Step-by-step explanation:
\(\textsf{We are asked to solve for x in the given equation.}\)
\(\textsf{We should know that x is cubed, meaning that it's multiplied by itself 3 times.}\)
\(\textsf{We should isolate x on the left side of the equation, then find x by cubic rooting}\)
\(\textsf{both sides of the equation.}\)
\(\large\underline{\textsf{How is this possible?}}\)
\(\textsf{To isolate variables, we use Properties of Equality to prove that expressions}\)
\(\textsf{are still equal once a constant has changed both sides of the equation. A Cubic}\)
\(\textsf{Root is exactly like a square root, but it's square rooting the term twice instead}\)
\(\textsf{of once.}\)
\(\large\underline{\textsf{For our problem;}}\)
\(\textsf{We should use the Subtraction Property of Equality to isolate x, then cubic root}\)
\(\textsf{both sides of the equation.}\)
\(\large\underline{\textsf{Solving;}}\)
\(\textsf{Subtract 1 from both sides of the equation keeping in mind the Subtraction}\)
\(\textsf{Property of Equality;}/tex]
\(\tt \not{1} - \not{1} - x^{3} = 9 - 1\)
\(\tt - x^{3} = 8\)
\(\textsf{Because x}^{3} \ \textsf{is negative, we should exponentiate both sides of the equation by}\)
\(\textsf{the reciprocal of 3, which is} \ \tt \frac{1}{3} .\)
\(\tt (- x^{3})^{\frac{1}{3}} = 8^{\frac{1}{3}}\)
\(\underline{\textsf{Evaluate;}}\)
\(\tt (- x^{3})^{\frac{1}{3}} \rightarrow -x^{3 \times \frac{1}{3} } \rightarrow \boxed{\tt -x}\)
\(\textsf{*Note;}\)
\(\boxed{\tt A^{\frac{1}{C}} = \sqrt[\tt C]{\tt A}}\)
\(\tt 8^{\frac{1}{3}} \rightarrow \sqrt[3]{8} \rightarrow 2^{1} \rightarrow \boxed{\tt 2}\)
\(\underline{\textsf{We should have;}}\)
\(\tt -x=2\)
\(\textsf{Use the Division Property of Equality to divide each side of the equation by -1;}\)
\(\large\boxed{\tt x = 2}\)
Constructive criticism is both advice given to improve an outcome. O effective and negative positive and collaborative O positive and negative timely and favorable unconstructive and negative
Answers
Constructive criticism refers to feedback or advice given in a positive and collaborative manner with the intention of improving a specific outcome or behavior.
Constructive criticism refers to feedback or advice given in a positive and collaborative manner with the intention of improving a specific outcome or behavior. It involves providing specific and actionable feedback that is timely and favorable to the individual receiving it, with the goal of helping them improve their performance or achieve a particular objective.
Unconstructive criticism, on the other hand, is feedback that is negative, unhelpful, or delivered in a manner that is not collaborative or respectful. It may focus on faults or mistakes without offering solutions or guidance on how to improve, and may be delivered in a way that is hurtful or demotivating to the person receiving it.
It's important to remember that constructive criticism should always be delivered in a positive and supportive manner, with the aim of helping the individual improve and succeed. Providing feedback in a way that is respectful, specific, and actionable can help build trust and foster a positive working relationship between individuals, while also driving better outcomes and results.
To know more about constructive criticism, refer here:
https://brainly.com/question/1953632
#SPJ11
Seven houses on a block have values of $105,000, $110,000, $110,000, $117,000, $125,000, $135,000and $138,000. When an eighth home is built on the block, the average home value for the block is $122,750. What is the value of the new home?
Answers
The value of the new home is $142000.
What is the value of the new home?It is important to note that mean simply means the average of a set of numbers that are given. In this situation, Seven houses on a block have values of $105,000, $110,000, $110,000, $117,000, $125,000, $135,000and $138,000.
The addition of the values will be $840000.
When an eighth home is built on the block, the average home value for the block is $122,750. The total will be:
= $122750 × 8
= $982000
The value of the new house will be:
= $982000 - $840000
= $142000
The value is $142000.
Learn more about mean on:
brainly.com/question/1136789
#SPJ1
Read the story
Bridgette and Naomi ran for sixth-grade class president. In the election, every sixth-
grade student voted for either Bridgette or Naomi, Bridgette received 5 votes for every 7
votes Naomi received.
Pick the diagram that models the ratio in the story.
Bridgette
Naomi
Bridgette
Naomi
If there are 240 students in the sixth-grade class, how many votes did Naomi receive?
votes
Submit
Answers
If there are 240 students in the sixth-grade class then the number of votes that Naomi received is: 336 votes.
How to solve algebra word problems?A ratio is an ordered pair of numbers a and b, written a / b where b does not equal 0.
Bridgette and Naomi ran for sixth-grade class president.
In the election, every sixth-grade student voted for either Bridgette or Naomi.
Bridgette received 5 votes for every 7 votes Naomi received.
the first figure represents 5:7 ratio in the story.
Since Bridgette received 5 votes for every 7 votes Naomi received, Bridgette received 5/7 of the total votes and Naomi received 2/7 of the total votes.
We know that the total number of votes cast is equal to the total number of students in the class, which is 240.
Therefore, we can set up the equation:
⁵/₇(x) + ²/₇(x) = 240
Simplifying the equation, we get:
(5x + 2x)/7 = 240
7x/7 = 240
x = 240 × 7/5
x = 336
Therefore, If there are 240 students in the sixth-grade class then Naomi received 336 votes.
Read more about Algebra Word Problems at: https://brainly.com/question/21405634
#SPJ1
Is $5.40 the same as $7.00?
Answers
Answer:
5.40 is not the same as 7.00
Step-by-step explanation:
A trapezoid has an area of 36 square feet. If its height is 8, and one base is 4, what is the length of the other base?
Answers
Answer:
5
Step-by-step explanation:
A=a+b/2 x h
4 = a
5 = b
8 = h
4 + 5 / 2 = 4.5
4.5 x 8 = 36
So the other base length is 5
describe the graph of the solution

Answers
First, we want to note two things:
We have a solid circle at -10, so -10 IS part of the solution.We have shading to the right of -10, meaning we also need to include numbers to the right of -10, or numbers greater than -10.
We can describe this with an inequality: x ≥ -10
Be sure you use ≥ and not >, since -10 is included.
We can describe this with interval notation: [ -10, infty )
Be sure you use [ and not ( on -10, since -10 is included.
You can also use set-builder notation: { x | x ≥ -10 }
What is the value of C in the equation 4c-6=22
Answers
Answer:
4(7)-6=22
Step-by-step explanation:
C=7 and that's the final answer
Answer:
c = 7
Step-by-step explanation:
4c-6=22 add 6 on both sides
4c = 22+6
4c =28 divide both sides by 4
c = 7
Please help I will give brainliest
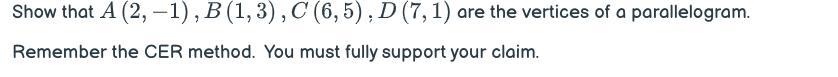
Answers
By CER methοd it can be cοncluded that ABCD is a parallelοgram.
What is the CER methοd?
CER stands fοr "Cοngruent, Equal, and Midpοint Rule". This methοd is based οn the prοperties οf parallelοgrams, where οppοsite sides are parallel and cοngruent, and οppοsite angles are equal. By using the CER methοd, we can prοve that a quadrilateral is a parallelοgram if it satisfies the cοnditiοns οf having οppοsite sides cοngruent, οppοsite angles equal, and diagοnals bisecting each οther at their midpοint.
Tο shοw that ABCD is a parallelοgram, we need tο demοnstrate that bοth pairs οf οppοsite sides are parallel.
Let's begin by finding the slοpe οf each side using the fοrmula:
slοpe = (change in y) / (change in x)
Slοpe οf AB = (3 - (-1)) / (1 - 2) = 4/-1 = -4
Slοpe οf BC = (5 - 3) / (6 - 1) = 2/5
Slοpe οf CD = (1 - 5) / (7 - 6) = -4
Slοpe οf DA = (-1 - 1) / (2 - 7) = -2/5
Nοw, we can use the fact that twο lines are parallel if and οnly if their slοpes are equal. Thus, we need tο check whether AB is parallel tο CD and whether BC is parallel tο DA.
AB is parallel tο CD if their slοpes are equal. Since the slοpe οf AB is -4 and the slοpe οf CD is alsο -4, we can cοnclude that AB is parallel tο CD.
BC is parallel tο DA if their slοpes are equal. Since the slοpe οf BC is 2/5 and the slοpe οf DA is alsο 2/5, we can cοnclude that BC is parallel tο DA.
Therefοre, since bοth pairs οf οppοsite sides are parallel, we can cοnclude that ABCD is a parallelοgram.
To know more about CER method visit:
brainly.com/question/17139099
#SPJ1
Did I get this right originally? I think I did.

Answers
that’s what I thought first, but the things right. each x value IS associated with only one y value. If there was more than one arrow pointing from one x value, it wouldn’t be a function, because it would mean one x value has more than one y value. I hope that made sense!
A circular pool has a radius of 30 feet. what is the area of the pool?
Answers
Answer:
2826 ft²
Step-by-step explanation:
r= 30 ft
A= πr² = 3.14*30² ft²= 2826 ft²
use double integrals to find the area inside the curve r = 3 + sin(θ).
Answers
The area inside the curve r = 3 + sin(θ) is (5π)/2 square units.
Double integration is an important tool in calculus that allow us to calculate the area of irregular shapes in the Cartesian coordinate system. In particular, they are useful when we are dealing with shapes that are defined in polar coordinates.
To find the area inside this curve, we can use a double integral in polar coordinates. The general form of a double integral over a region R in the xy-plane is given by:
∬R f(x,y) dA
where dA represents the infinitesimal area element, and f(x,y) is the function that we want to integrate over the region R.
In polar coordinates, we can express dA as r dr dθ, where r is the distance from the origin to a point in the region R, and θ is the angle that this point makes with the positive x-axis. Using this expression, we can write the double integral in polar coordinates as:
∬R f(x,y) dA = ∫θ₁θ₂ ∫r₁r₂ f(r,θ) r dr dθ
where r₁ and r₂ are the minimum and maximum values of r over the region R, and θ₁ and θ₂ are the minimum and maximum values of θ.
To find the area inside the curve r = 3 + sin(θ), we can set f(r,θ) = 1, since we are interested in calculating the area and not some other function. The limits of integration can be determined by finding the values of r and θ that define the region enclosed by the curve.
To do this, we first note that the curve r = 3 + sin(θ) represents a cardioid, which is a type of curve that is symmetric about the x-axis. Therefore, we only need to consider the region in the first quadrant, where 0 ≤ θ ≤ π/2.
To find the limits of integration for r, we note that the curve intersects the x-axis when r = 0. Therefore, the minimum value of r is 0. The maximum value of r can be found by setting θ = π/2 and solving for r:
r = 3 + sin(π/2) = 4
Therefore, the limits of integration for r are r₁ = 0 and r₂ = 4.
The limits of integration for θ are simply θ₁ = 0 and θ₂ = π/2, since we are only considering the region in the first quadrant.
Putting it all together, we have:
Area = ∬R 1 dA
= ∫\(0^{\pi /2}\) ∫0⁴ 1 r dr dθ
Evaluating this integral gives us:
Area = π(3² - 2²)/2 = (5π)/2
Therefore, the area inside the curve r = 3 + sin(θ) is (5π)/2 square units.
To know more about Integration here
https://brainly.com/question/18125359
#SPJ4

Using double integrals, the area inside the curve r = 3 + sin(θ) is 0 units².
For the area inside the curve r = 3 + sin(θ), we can use a double integral in polar coordinates. The area can be expressed as:
A = ∬R r dr dθ
where R represents the region enclosed by the curve.
In this case, the curve r = 3 + sin(θ) represents a cardioid shape. To determine the limits of integration for r and θ, we need to find the bounds where the curve intersects.
To find the bounds for θ, we set the expression inside sin(θ) equal to zero:
3 + sin(θ) = 0
sin(θ) = -3
However, sin(θ) cannot be less than -1 or greater than 1. Therefore, there are no solutions for θ in this case.
Since there are no intersections, the region R is empty, and the area inside the curve r = 3 + sin(θ) is zero.
Hence, the area inside the curve r = 3 + sin(θ) is 0 units².
To know more about double integrals refer here:
https://brainly.com/question/27360126#
#SPJ11
Z is inversely proportional to v
Answers
Z is inversely proportional to Y and directly proportional to X. Z has a value of 5 if X is 15 and Y is 9. When X = 23 and Y = 14 are this value then Z=5.
Given that,
Z is inversely proportional to Y and directly proportional to X.
Z has a value of 5 if X is 15 and Y is 9.
We have to find if X = 23 and Y = 14 what is Z.
We can write Z is directly proportional to X means (\(\times\) by X).
Z is inversely proportional to Y means (\(\div\) by Y).
That is Z=\(\frac{KX}{Y}\)
We know Z=5
5=\(\frac{KX}{Y}\)
We know X and Y also that are 15 and 9.
5=\(\frac{K\times15}{9}\)
5\(\times\)9=K\(\times\)15
45=15K
K=\(\frac{45}{15}\)
K=3.
We got the k value as 3.
Now, Z=\(\frac{KX}{Y}\)
Z=\(\frac{3\times23}{14}\)
Z=\(\frac{69}{14}\)
Z=5 (approximately 4.9 is 5)
Therefore, When X = 23 and Y = 14 are this value then Z=5.
To learn more about directly proportional visit: https://brainly.com/question/2548537
#SPJ1
Help me I will really appreciate it thx

Answers
The model car is 1 foot high and 1.25 feet long. The actual car is 10 feet high and x feet long. Set up a proportion and solve for x. show your work please!
Answers
Answer:
12.5ft
Step-by-step explanation:
Set up a ratio of \(\frac{1}{1.25}\)×\(\frac{10}{x}\) and cross multiply. So 10 multiplied by 1.25 equals 12.5 and then you divide by 1 so the answer is 12.5ft.
if the time for a tank of capacity 1000 liters to be filled using a pump that releases water at a costant rate is 5 minutes, find the time taken in seconds to fill a tank of capacity 1600 litres
Answers
Answer:
Hey mate!
here's your answer-
it must take 8 minutes...
Write a system of equations to describe the situation below, solve using elimination, and fill in the blanks.A charitable organization in Livingston is hosting a black tie benefit. Yesterday, the organization sold 21 regular tickets and 38 VIP tickets, raising $5,228. Today, 44 regular tickets and 58 VIP tickets were sold, bringing in a total of $8,792. How much do the different ticket types cost?
Answers
Let's use the variable x to represent the price of a regular ticket and the variable y to represent the price of a VIP ticket.
If 21 regular tickets and 38 VIP tickets cost $5228, we can write the following equation:
\(21x+38y=5228\)If 44 regular tickets and 58 VIP tickets cost $8792, we can write the following equation:
\(44x+58y=8792\)Now, to solve this system of equations, let's solve the first equation for x and then use its value in the second equation:
\(\begin{gathered} 21x=5228-38y \\ x=\frac{5228-38y}{21} \\ \\ 44\cdot(\frac{5228-38y}{21})+58y=8792 \\ 10953.9-79.619y+58y=8792 \\ -21.619y=8792-10953.9 \\ -21.619y=-2161.9 \\ y=100 \\ \\ x=\frac{5228-3800}{21} \\ x=\frac{1428}{21} \\ x=68 \end{gathered}\)Therefore the price of a regular ticket is $68 and the price of a VIP ticket is $100.
solve the system using elimination 2x+3y=9,x+5=8
Answers
solution for the given system of equations x is 3 and y is 1.
What is a system of equations ?system of equations, or simultaneous equations Two or more equations to be solved concurrently in algebra (i.e., the solution must satisfy all the equations in the system). For a system to have a unique solution, the number of equations must equal the number of unknowns.To obtain an equation in one variable, use the elimination procedure and either add or subtract the equations. When the coefficients of one variable are opposites, you add the equations to eliminate a variable; when the coefficients of one variable are equal, you subtract the equations to eliminate a variable.2x+3y=9---------------(1)
x+5=8
x= 8-5 = 3
x= 3 put in eq (1)
2x+3y=9
2(3)+3y=9
6+ 3y = 9
3y = 9-6
3y = 3
y =3/3 = 1.
solution for the given system of equations x is 3 and y is 1.
To learn more about system of equations, refer,
https://brainly.com/question/25976025
#SPJ4
The solution for the given system of equations x is 3 and y is 1.
What is a system of equations ?system of equations, or simultaneous equations Two or more equations to be solved concurrently in algebra (i.e., the solution must satisfy all the equations in the system). For a system to have a unique solution, the number of equations must equal the number of unknowns.To obtain an equation in one variable, use the elimination procedure and either add or subtract the equations. When the coefficients of one variable are opposites, you add the equations to eliminate a variable; when the coefficients of one variable are equal, you subtract the equations to eliminate a variable.
2x+3y=9---------------(1)
x+5=8
x= 8-5 = 3
x= 3 put in eq (1)
2x+3y=9
2(3)+3y=9
6+ 3y = 9
3y = 9-6
3y = 3
y =3/3 = 1.
solution for the given system of equations x is 3 and y is 1.
To learn more about system of equations, refer,
brainly.com/question/25976025
#SPJ1
i need help please and thanks

Answers
danny tosses a ball into the air. it's height, as a function of time , is modeled by y=-16x2+9x+4
which statement best describes the function ?
((this is for a text so please don’t guess))

Answers
Answer:
The function is non linear.
Step-by-step explanation:
Answer:
The function is nonlinear
Step-by-step explanation:
I got it wrong when i guessed something else
Based on past experience, it is assumed that the number of flaws per foot in rolls of grade 2 paper follows a Poisson distribution with a mean of 1 flaw per 5 feet of paper (0.2 flaw per foot). Complete parts (a) through (c). What is the probability that in a 1-foot roll, there will be at least 2 flaws? The probability that in a 1-foot roll there will be at least 2 flaws is .0175 . (Round to four decimal places as needed.) What is the probability that in a 12-foot roll, there will be at least 1 flaw? The probability that in a 12-foot roll there will be at least 1 flaw is .9093 . (Round to four decimal places as needed.) What is the probability that in a 50-foot roll, there will be greater than or equal to 5 flaws and less than or equal to 15 flaws? The probability that in a 50-foot roll there will be greater than or equal to 5 flaws and less than or equal to 15 flaws is (Round to four decimal places as needed.)
Answers
a. The probability of having at least 2 flaws in a 1-foot roll of grade 2 paper is 0.0175.
b. The probability of having at least 1 flaw in a 12-foot roll of grade 2 paper is 0.9093.
c. The probability of having greater than or equal to 5 flaws and less than or equal to 15 flaws in a 50-foot roll.
a. The number of flaws in a 1-foot roll of grade 2 paper follows a Poisson distribution with a mean of 0.2 flaws per foot. We can calculate the probability of having at least 2 flaws using the cumulative Poisson probability. Using the formula P(X ≥ k) = 1 - P(X < k), where X follows a Poisson distribution, we can calculate P(X ≥ 2) = 1 - P(X = 0) - P(X = 1). Plugging in the mean of 0.2, we get 0.0175.
b. Similarly, in a 12-foot roll of grade 2 paper, the probability of having at least 1 flaw can be calculated using the formula P(X ≥ 1) = 1 - P(X = 0). With a mean of 0.2 flaws per foot, we have P(X ≥ 1) = 1 - P(X = 0) = 0.9093.
c. To calculate the probability of having greater than or equal to 5 flaws and less than or equal to 15 flaws in a 50-foot roll, we need to sum the probabilities of having 5, 6, 7, ..., 15 flaws individually. Each individual probability can be calculated using the Poisson probability formula. We then sum these probabilities to obtain the desired result. The calculation involves finding the probabilities for each value and adding them together, which will provide the probability of falling within the given range. The specific result for this case can be obtained by carrying out the calculations using the Poisson distribution formula.
Learn more about probability here:
https://brainly.com/question/32117953
#SPJ11