Answers
Related Questions
Simplify the expression below:
3(6y - 2x)
Answers
Answer:
18y-6x
Step-by-step explanation:
Multiply what is inside the brackets by what is outside, in this case 3.
Write the exact value of the side length of a square whose are in square units are given. For example, use sqrt(9) to represent the square root of 9. Areas: 36 37 100/9 2/5 0.0001 0.11
Answers
Answer:
a.) Side length of the square is 6 units.
b.) Side length of the square is sqrt(37) units.
c.) Side length of the square is 10/3 units.
d.) Side length of the square is sqrt(2/5) units.
e.) Side length of the square is 0.01 units.
f.) Side length of the square is sqrt(11)/10 units.
Step-by-step explanation:
To find - Write the exact value of the side length of a square whose are in square units are given
a) 36
b) 37
c) 100/9
d) 2/5
e) 0.0001
f) 0.11
Proof -
We know that ,
If a is the side length of the square then,
Area of square = a²
Now,
a) 36
⇒a² = 36
⇒a² = (6)²
⇒a = 6
∴ we get
Side length of the square is 6 units.
b.) 37
⇒a² = 37
⇒a² = [sqrt(37)]²
⇒a = sqrt(37)
∴ we get
Side length of the square is sqrt(37) units.
c.)100/9
⇒a² = 100/9
⇒a² = [10/3]²
⇒a = 10/3
∴ we get
Side length of the square is 10/3 units.
d.)2/5
⇒a² = 2/5
⇒a² = [sqrt(2/5)]²
⇒a = sqrt(2/5)
∴ we get
Side length of the square is sqrt(2/5) units.
e.)0.0001
⇒a² = 0.0001
⇒a² = [0.01]²
⇒a = 0.01
∴ we get
Side length of the square is 0.01 units.
f.)0.11
⇒a² = 0.11
⇒a² = [sqrt(11)/10]²
⇒a = sqrt(11)/10
∴ we get
Side length of the square is sqrt(11)/10 units.
We get the value of the,
a.) Side length of the square is 6 units.
b.) Side length of the square is sqrt(37) units.
c.) Side length of the square is 10/3 units.
d.) Side length of the square is sqrt(2/5) units.
e.) Side length of the square is 0.01 units.
f.) Side length of the square is sqrt(11)/10 units.
We have to find the exact value of the side length of a square whose are in square units are given
a) 36
b) 37
c) 100/9
d) 2/5
e) 0.0001
f) 0.11
We have given that,sqrt(9) to represent the square root of 9.
We know that ,
If a is the side length of the square then,
what area of the square?Area of square = a²
For option a) 36
⇒a² = 36
⇒a² = (6)²
⇒a = 6
∴ we get side length of the square is 6 units.
For the option b is 37.
⇒a² = 37
⇒\(a^2 = \sqrt{37} ^2\)
⇒\(a = sqrt(37)\)
∴ we get side length of the square is \(\sqrt(37)\) units.
c.)100/9
⇒\(a^2 = 100/9\)
⇒\(a^2= [10/3]^2\)
⇒\(a = 10/3\)
∴ we get side length of the square is 10/3 units.
for the option d.)2/5
\(a^2= 2/5\)
\(a^2 = [\sqrt(2/5)]^2\)
⇒\(a = \sqrt(2/5)\)
∴ we get side length of the square is \(\sqrt(2/5)\)units.
for the option e.)0.0001
\(a^2 = 0.0001\)
\(a^2 = [0.01]^2\)
\(a = 0.01\)
∴ we get side length of the square is 0.01 units.
For option f.)0.11
⇒\(a^2 = 0.11\)
⇒\(a^2= [\sqrt(11)/10]^2\)
\(a = \sqrt(11)/10\)
∴ we get side length of the square is\(\sqrt(11)/10\) units.
Therefore we get,we get the value of the,
a.) Side length of the square is 6 units.
b.) Side length of the square is sqrt(37) units.
c.) Side length of the square is 10/3 units.
d.) Side length of the square is sqrt(2/5) units.
e.) Side length of the square is 0.01 units.
f.) Side length of the square is sqrt(11)/10 units.
To learn more about the area visit:
https://brainly.com/question/25292087
whats the factor monimial of 14n⁴ please help
Answers
Answer:
Its gonna equal itself which is 14n^4
Step-by-step explanation:
14n4
=14*n4
=14*(n*n*n*n)
=14n4
The minimum monthly payment for Brian's credit card is 3.5% of his balance or $25, whichever is higher. If Brian's balance at the end of his last billing cycle was $722, what is his minimum monthly payment?
Answers
The minimum monthly payment for Brian is $25.
How to find the minimum payment?The minimum payment is the smallest amount of money you must pay each month to maintain the status of your account. The statement balance is your total account balance for that billing cycle. The current balance is the sum of your most recent bill plus any charges.
From the information, the minimum monthly payment for Brian's credit card is 3.5% of his balance or $25
Since the balance was $722, the amount will be:
= 3.5% × $722
= 0.035 × $722
= $25.27
Since $25.27 is more than $25, the minimum monthly payment is $25.
Learn more about percentages on:
brainly.com/question/24877689
#SPJ1
Please show me the answers step by step. I need to show my work
The volume in cubic feet of a workshop's storage chest can be expressed as the product of its three dimensions: V(x) = x^3 - 3x^2 - x +3. The depth is x + 1.
A. Find linear expressions with integer coefficients for the other dimensions.
B. If the depth of the chest is 6 feet what are the other dimensions?
Answers
The answer is in the picture I included.

A random sample of size n=200 is to be taken from a uniform population with α=24 and β=48. Based on the central limit theorem, what is the probability that the mean of the sample will be less than 35?
Answers
The probability that the mean of the sample will be less than 35 is approximately 0.0205, or 2.05%.
To solve this problem, we'll use the central limit theorem, which states that for a large enough sample size, the distribution of sample means approximates a normal distribution, regardless of the shape of the population distribution.
Given that the population follows a uniform distribution with α = 24 and β = 48, we know that the mean (μ) of the population is given by the formula:
μ = (α + β) / 2
Substituting the values, we have:
μ = (24 + 48) / 2 = 72 / 2 = 36
The standard deviation (σ) of the population is given by the formula:
σ = (β - α) / √12
Substituting the values, we have:
σ = (48 - 24) / √12 = 24 / √12 = 24 / 3.464 = 6.928
According to the central limit theorem, the distribution of sample means follows a normal distribution with a mean equal to the population mean (μ) and a standard deviation equal to the population standard deviation (σ) divided by the square root of the sample size (n). Therefore:
μ_s = μ = 36
σ_s = σ / √n = 6.928 / √200 ≈ 0.490
To find the probability that the mean of the sample will be less than 35, we need to find the area under the normal distribution curve to the left of 35. We'll use a standard normal distribution with a mean of 0 and a standard deviation of 1, and then transform it using the mean and standard deviation of the sample distribution.
Let's calculate the z-score for 35:
z = (x - μ_s) / σ_s = (35 - 36) / 0.490 ≈ -2.041
Using a standard normal distribution table or a calculator, we can find the probability corresponding to a z-score of -2.041. The probability that the mean of the sample will be less than 35 is approximately 0.0205, or 2.05%.
To know more about central limit theorem refer to
https://brainly.com/question/18403552
#SPJ11
{122,132,155,198,213,225,261,280} what is the mean absolute deviation for the data set? enter your answer as a number rounded to the nearest tenth, like this: 42.5
Answers
The mean absolute deviation (MAD) for the given data set is approximately 46.5.
How is the mean absolute deviation (MAD) calculated?The mean absolute deviation (MAD) for a data set is calculated by finding the mean (average) of the data set, then calculating the absolute deviation for each data point by minus the mean from each data point and taking the absolute value. Finally, the mean of the absolute deviations is computed to determine the MAD.
To calculate the mean absolute deviation (MAD) for the given data set, we follow these steps:
1.Find the mean value of the data set.
2.Calculate the absolute deviation for each data point by taking difference the mean from each data point and taking the absolute value.
3.Find the mean of the absolute deviations.
Now calculate the mean absolute deviation for the data set:
Step 1: Find the mean:
\(Mean =\frac{122 + 132 + 155 + 198 + 213 + 225 + 261 + 280}{8}\\ = \frac{1586}{ 8}\\ = 198.25\)
Step 2: Calculate the absolute deviation for each data point and find the sum:
|122 - 198.25| = 76.25
|132 - 198.25| = 66.25
|155 - 198.25| = 43.25
|198 - 198.25| = 0.25
|213 - 198.25| = 14.75
|225 - 198.25| = 26.75
|261 - 198.25| = 62.75
|280 - 198.25| = 81.75
Sum of absolute deviations = 76.25 + 66.25 + 43.25 + 0.25 + 14.75 + 26.75 + 62.75 + 81.75
= 372
Step 3: Find the mean absolute deviations:
\($\rm MAD =\frac{\text{Sum of absolute deviations}} { \text{Number of data points}}\\ &\\$\text{Number of data points }=$\frac{372}{8}\\ $= 46.5$\)
Rounded to the nearest tenth, the mean absolute deviation (MAD) for the given data set is approximately 46.5.
Therefore,the mean absolute deviation (MAD) for the given data set is approximately 46.5.
To learn more about mean absolute deviation from the given link
brainly.com/question/447169
#SPJ4
yesterday, 28 students took a test. the arithmetic mean of those 28 scores was 72 points. two students who were absent yesterday took the test this morning, and the arithmetic mean of all 30 test scores is 73 points. if the difference of the two scores from this morning is 22 points, what is the lower score from this morning?
Answers
Let's assume the sum of the scores of the 28 students who took the test yesterday is 28 * 72 = 2016.
We know that the sum of the scores of all 30 students is (28 * 72) + x + y, where x and y are the scores of the two students who took the test this morning.
We also know that the mean of all 30 scores is 73. So we can write an equation:
[(28 * 72) + x + y]/30 = 73
Multiplying both sides by 30, we get:
2016 + x + y = 2190
So, x + y = 174.
We are given that the difference of the two scores is 22, so we can write another equation:
y - x = 22
Solving these two equations simultaneously, we get y = 98 and x = 76.
Therefore, the lower score from this morning is 76.
To learn more refer below:
https://brainly.com/question/18495602
#SPJ11
Find the particular antiderivative of the following derivative that satisfies the given condition. C ′
(x)=2x 2
−4x;C(0)=1,000 C(x)=
Answers
The particular antiderivative of the derivative C'(x) = 2x^2 - 4x which satisfies the given condition C(0) = 1000 is C(x) = [(2 * (x³/3)) - (4 * (x²/2))] + 1000
Particular antiderivative of the derivative C'(x) = 2x^2 - 4x which satisfies the given condition C(0) = 1000 is
C(x) = ∫C'(x) dx
C(x) = ∫(2x² - 4x) dx
C(x) = (2∫x² dx) - (4∫x dx)
C(x) = [(2 * (x³/3)) - (4 * (x²/2))] + C
Differentiating both sides w.r.t. x will give us
C'(x)C'(x) = d/dx(C(x))
C'(x) = (d/dx) [(2x³/3) - (2x²)] + 0
C'(x) = [2(x^2) - 4(x)]
Since the particular antiderivative satisfies the given condition
C(0) = 1000, putting x = 0 in the antiderivative C(x),
we get:
C(0) = [(2 * 0³/3) - (4 * 0²/2)] + C
= 0 - 0 + C
= C = 1000
Therefore, the particular antiderivative of the derivative
C'(x) = 2x² - 4x which satisfies the given condition C(0) = 1000 is:
C(x) = [(2 * (x³/3)) - (4 * (x²/2))] + 1000
To know more about antiderivative visit:
https://brainly.com/question/30764807
#SPJ11
Using the model, approximate the number of ice cream cones sold when it is 85°F. Round your answer to the nearest whole number.

Answers
Answer:
76.1
Step-by-step explanation:
y = ice cream
z = Tempreature
y = 1.8(85) - 76.9
1.8(85) = 153
153 - 76.9 = 76.1
Answer:
76
Step-by-step explanation:
y = 1.8(85) - 76.9
y = 153 - 76.9
y = 76.1
and when you round to the nearest whole number,
y = 76
(q24) Find the volume of the solid obtained by rotating the region bounded by y = x and y = x^2 about the line x = - 3.

Answers
The volume of the solid is (11π/3) cubic units.
We have,
To find the volume of the solid obtained by rotating the region bounded by y = x and y = x^2 about the line x = -3, we can use the method of cylindrical shells.
The formula for the volume using cylindrical shells is given by:
V = 2π ∫ [a, b] x h(x) dx,
where [a, b] is the interval of integration, x represents the variable of integration, and h(x) represents the height of the shell at each value of x.
In this case, we want to rotate the region bounded by y = x and y = x² about the line x = -3.
Since we are rotating about a vertical line, the height of the shell at each value of x will be given by the difference between the x-coordinate of the curve and the line of rotation:
h(x) = (x - (-3)) = x + 3.
To find the interval of integration, we need to determine the x-values where the two curves intersect.
Setting x = x², we have:
x = x²,
x² - x = 0,
x (x - 1) = 0.
This gives us two intersection points: x = 0 and x = 1.
Therefore, the interval of integration is [0, 1].
Now we can set up the integral to find the volume:
V = 2π ∫ [0, 1] x (x + 3) dx.
Evaluating this integral, we have:
V = 2π ∫ [0, 1] (x² + 3x) dx
= 2π [x³/3 + (3/2)x²] evaluated from 0 to 1
= 2π [(1/3 + 3/2) - (0/3 + 0/2)]
= 2π [(2/6 + 9/6) - 0]
= 2π (11/6)
= (22π/6)
= (11π/3).
Therefore,
The volume of the solid is (11π/3) cubic units.
Learn more about the volume of solids here:
https://brainly.com/question/31259146
#SPJ1
Liz spent her summer break working at Wild Lakes waterpark. At the end of the summer, she put $570 of her earnings into a savings account that earns 1% interest each year.
Answers
The total simple interest earned by Liz at the end of 5 years is found as: $28.5
Explain about the simple interest?A straightforward but effective idea. Very simply: interest is the benefit for saving - and the penalty of borrowing.
When you deposit funds into a savings account, you are paid interest, or additional money on top. The reason for this is that the bank gives you interest for letting them use your money.When someone owes money, they are assessed this fee. It indicates the time worth of money and is charged in relation to the principal sum. It is the prize for holding out and refusing to accept an early reward.Principal P = $570
Interest r = 1%
Time t = 5 years.
SI = P*R*t / 100
SI = 570*1*5 / 100
SI = 28.5
Thus, the total simple interest earned by Liz at the end of 5 years is found as: $28.5.
Know more about the simple interest
https://brainly.com/question/25793394
#SPJ9
The complete question is-
Liz spent her summer break working at Wild Lakes waterpark. At the end of the summer, she put $570 of her earnings into a savings account that earns 1% interest each year. Find her simple interest after 5 years.
Someone plss help me on this one too!
Thanks :)

Answers
Answer: 30%
Step-by-step explanation:
Chris bought 12.4 gallons of gasoline. There are approximately 3.8 liters in 1 gallon. Which measurement is closetsto the number of liters of gasoline Chris bought? A 11.44 L B 2.74 L C 47.12 L D 14.20 L2
Answers
Answer:
C 47.12 L
Step-by-step explanation:
1 gallon = 3.8 liters
12.4 gallons = x
Cross Multiply
= 12.4 × 3.8
47.12 liters
Option C is correct
PLEASE HELP
When rolling a fair, eight-sided number cube, determine P(number greater than 2).
0.25
0.50
0.66
0.75
Answers
The value of P(number greater than 2) when rolling a fair, eight-sided number cube is 0.75
Determining P(number greater than 2) when rolling a fair, eight-sided number cubeFrom the question, we have the following parameters that can be used in our computation:
Rolling a fair, eight-sided number cube
The sample space of a eight-sided number cube is
S = {1, 2, 3, 4, 5, 6, 7, 8}
Where, the outcomes greater than 2 are
Outcomes = {3, 4, 5, 6, 7, 8}
Using the above as a guide, we have the following:
P(number greater than 2) = n(Outcomes)/n(S)
So, we have
P(number greater than 2) = 6/8
Evaluate
P(number greater than 2) = 0.75
Hence, the value of P(number greater than 2) is 0.75
Read more about probability at
https://brainly.com/question/24756209
#SPJ1
PLS
Natasha has a photo of a lasagna dish she made, which she wants to post to various
websites. The original image has a width of 300 pixels and a height of 450 pixels.
Consider each set of new dimensions or scale percents that show adjustments to this
original image. Describe how the image changed and whether the new image is
similar to the original. Show your work and explain your reasoning.
New image: 35% width, 35% height
Answers
As both the width and height of the new image are 35% of the width and height of the original image, the aspect ratio is maintained and the new image is similar to the original image.
What is a Scale Factor?A scale factor is defined as the ratio of an object's original scale to that of a new one, considering the different depictions of that object (bigger or smaller)
Given:
The dimensions of the original image are a width of 300 pixels and a height of 450 pixels.
The dimensions of the New image have 35% width and 35% height.
Width = 0.35 × 300 = 105
Height = 0.35 × 450 = 157.5
Scale factor = New Dimension/Original Dimension
Scale factor of Height = 157.5/450 = 0.35
Scale factor of width = 105/300 = 0.35
Therefore, the new image is neither scaled down nor scaled up and is the same as the original image.
To learn more about scale factor visit:
https://brainly.com/question/22312172
#SPJ13
when will a normal probability plot appear linear and what does that indicate?
Answers
A normal probability plot (also known as a normal plot or a probability plot) is a graphical tool that can be used to assess whether a set of data follows a normal distribution.
When the data being plotted follows a normal distribution, the points on the plot will appear roughly linear, with deviations from linearity being relatively minor. In other words, the plot will form a straight line.
If the plot deviates significantly from a straight line, it suggests that the data may not be normally distributed. The shape of the plot can indicate whether the data is skewed or has heavy tails compared to a normal distribution.
Therefore, a normal probability plot appearing linear indicates that the data follows a normal distribution. It is important to note that this is only one way to check for normality, and it is always recommended to use multiple methods to verify the normality assumption before applying statistical tests that assume normality.
for such more question on normal distribution
https://brainly.com/question/25224028
#SPJ11
What is the standard form of a trinomial?
Answers
Answer:
ax2 + bx + c
Step-by-step explanation:
the 2 is the square
Helpppppppp againnnnnn
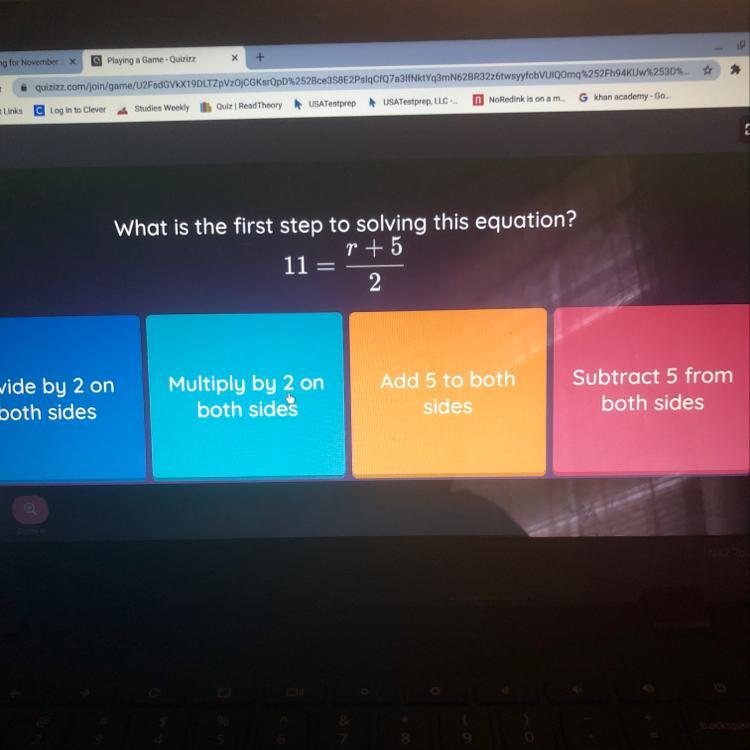
Answers
Answer: Multiply by 2 on both sides
Answer:
Multiply by 2 on both sides
Step-by-step explanation:
Hope I helped
Which graph represents
(x-2)²_(v + 1)² <
16
-<1?
ਪੁ
7.
6+
5+
44
3+
2-
+|+||||||
-
-9-8-7-6-5-4-3-2-1+12345678910111213
-2-
-3-
-4-
5+
-6-
-7-
-8-

Answers
The graph of equation of Hyperbola (x - 2)²/16 - (y + 1)²/9 ≤ 1 is shown in image.
Since, We know that;
Hyperbola is defined as, two-branched open curve, a conic section, produced by the intersection of a circular cone and a plane that cuts both nappes of the cone.
Here, We have to given that;
The equation of Hyperbola is,
⇒ (x - 2)²/16 - (y + 1)²/9 ≤ 1
Since, WE know that;
Standard form of equation of Hyperbola is,
⇒ (x - h)²/a² - (y - k)²/b² = 1
Hence, Above equation shows the Hyperbola.
So, By using tool we can draw the graph equation of Hyperbola
(x - 2)²/16 - (y + 1)²/9 ≤ 1 as shown in figure.
Therefore, The graph of equation of Hyperbola (x - 2)²/16 - (y + 1)²/9 ≤ 1 is shown in image.
Learn more about hyperbola at:
https://brainly.com/question/26250569
#SPJ1

Given: L-Lcos 0=v²/2 Solve for 0 O 0 =cos ¹[1+v²/(2L)] Oe=cos ¹[1-v²(2L)] O 0 =cos ¹¹[1-v²/(2L)] Oe=cos[1-v²/(2L)]
Answers
cos-¹[1 + v²/2L], cos-¹[1 - v²/2L], cos[1 + v²/2L], cos[1 - v²/2L]
Given: L-Lcos0=v²/2
Let's solve for 0.From L - Lcos 0 = v²/2cos 0 = 1 - v²/2LThus, cos 0 = 1 - v²/2L.We need to find the value of 0. So, we will use the inverse cosine function.The inverse cosine of (1 - v²/2L) is equal to the angle whose cosine is (1 - v²/2L).
Therefore, 0 = cos-¹[1 - v²/2L]
Thus, cos-¹[1 + v²/2L], cos-¹[1 - v²/2L], cos[1 + v²/2L], cos[1 - v²/2L]
To know more about inverse cosine function. visit:
brainly.com/question/14345853
#SPJ11
Riddle time!
A child was given a bicycle and a soccer ball for Christmas by Santa,
but the child was not happy. Why?
Answers
Answer: Because it's winter therefore he can't enjoy the gift. or he can't walk
Step-by-step explanation:
√14y²-20y+48+√14y²-20y-15=9
√14y²-20y+48-√14y²-20y-15=....?
Answers
Answer:
=−40y+33
Step-by-step explanation:
√14y2−20y+48−√14y2−20y−15
Simplifies to:
−40y+33
=−40y+33
which answer is it????

Answers
Answer:
A
Step-by-step explanation:
1 oboe in every 7 clarinets so there would be 2 in 14 and 3 in 21
ck Assignment - Unit 2A: ( Number of bagels is (Type an expression using b as the variable.) Question 6, 1.1.32 > HW Score: 21.43%, 3 of 14 points O Points: 0 of 1 Yolanda McClanahan € A bagel shop sells bagels by the score. The table at the right shows the number of bagels a shop gives per "score" ordered. Write an algebraic expression that gives the rule for finding the number of bagels in any number b of scores. Save Bagels Scores Number of Bagels 7 140 8 160 9 180 b
Answers
The proportional relationship that gives the rule for finding the number n of bagels in any number b of scores is:
n = 0.05b.
What is a proportional relationship?A proportional relationship is a function in which the output variable is given by the input variable multiplied by a constant of proportionality, that is:
y = kx
In which k is the constant of proportionality.
For this problem, we want to number n of bagels in b scores, hence the format of the relation is:
n = kb.
The number is 1/20 of the scores, hence the constant is k = 1/20 = 0.05 and the equation is:
n = 0.05b.
More can be learned about proportional relationships at https://brainly.com/question/10424180
#SPJ1
At Al's farm 15 oranges cost, $2.25, How much does 1 cost?
Answers
Answer:
15 cents
Step-by-step explanation:
2.25 /15 = .15
help meeeeeeeeeeeeeeeeeee

Answers
This is the answer
Find the values of x and y.
5.
80
X
y

Answers
Answer:
x=80 y=80
Step-by-step explanation:
x must be 80 because with an interception like this the angles that are opposite of each other will be the same degrees.
y must be 80 because it has a line parallel to the line that 80 is on
One letter is chosen at random from the word THANKS. A letter is then chosen at random from the word STARK
1. Write out ALL of the outcomes in the sample space of this chance experiment.
. 2. How many outcomes are in the sample space?
3. What is the probability that the letters chosen are AA?
Answers
1. The outcomes in the sample space of choosing a letter from the word STARK are: S, T, A, R, K.
2. Number of outcomes in the sample space = 6 (from THANKS) × 5 (from STARK) = 30.
3. The Probability of choosing the letters AA is 0, as there are no occurrences of the letter A in both words together.
1. The outcomes in the sample space of choosing a letter from the word THANKS are: T, H, A, N, K, S.
The outcomes in the sample space of choosing a letter from the word STARK are: S, T, A, R, K.
2. To find the number of outcomes in the sample space, we multiply the number of outcomes for each word.
Number of outcomes in the sample space = Number of outcomes for the first word × Number of outcomes for the second word
Number of outcomes in the sample space = 6 (from THANKS) × 5 (from STARK) = 30.
3. The probability of choosing the letters AA would be the number of favorable outcomes (which is 0 in this case) divided by the total number of outcomes in the sample space.
Probability of choosing AA = Number of favorable outcomes / Total number of outcomes
Probability of choosing AA = 0 / 30 = 0.
Therefore, the probability of choosing the letters AA is 0, as there are no occurrences of the letter A in both words together.
To know more about Probability .
https://brainly.com/question/13604758
#SPJ11
Describe how the graph of ()=(.)− compares to the graph of ()=(.)f of open x close equals 4 . open 0.5 close to the x.
Enter your answer.
Answers
Every point on the graph of g(x) is 1 unit higher than the corresponding point on the graph of f(x).
Describing how the graph comparesGiven that
f(x) = 4(0.5)^x and g(x) = 4(0.5)^x + 1
The graphs of the functions f(x) = 4(0.5)^x and g(x) = 4(0.5)^x + 1 are both exponential functions with a base of 0.5.
The only difference between these two functions is the addition of a constant term of 1 to the second function.
This constant term shifts the graph of g(x) vertically upward by 1 unit compared to the graph of f(x).
Read more about transformation at
https://brainly.com/question/1548871
#SPJ1
Complete question
Describe how the graph of f(x) = 4(0.5)^x compares to the graph of g(x) = 4(0.5)^x +1
