Tina is cutting construction paper into rectangles for a project. She needs to cut one rectangle that is 15 inches × 12 1/3 inches. She needs to cut another rectangle that is 10 1/4 inches by 10 1/3 inches. How many total square inches of construction paper does Tina need for her project?
Answers
Answer:
Step-by-step explanation:
Remark
This is an "in theory" type of question. She's going to actually need a little more.
Area 1
L = 15
W = 12 1/3
Area = L*W
Area = 15 * 12 1/3 I wonder if you know what the distributive property is. I'll try it. If you don't understand, leave a remark
Area = 15 * (12 + 1/3) Multiply both parts by 15
Area = 15*12 + 1/3 * 15
Area = 180 + 5
Area = 185 square inches.
Area 2.
This is a little harder because their are
2 fractions.
The easy way to do this is change to decimals
L = 10 1/3 = 10.33333333
W = 10 1/4 = 10.25
Area = 10.3333333 * 10.25
Area = 105.92 Add
Total Area
The total area = 290.92
Related Questions
at a party there are only single women and married men with their wives. the probability that a randomly selected woman is single is $\frac{2}{5}$. what fraction of the people in the room are married men?
Answers
60% of the people in the room are married men.
We can use the information that the probability of a randomly selected woman being single is 2/5 to find the fraction of people in the room who are married men.
Let's call the fraction of people in the room who are married men x. The fraction of people in the room who are single women is (2/5) and the fraction of people in the room who are married men and their wives is (1-x)
Since there are only single women and married men with their wives at the party, we know that the sum of the fractions of single women and married men and their wives must be 1.
(2/5) + (1-x) = 1
We can solve this equation for x:
x = 1 - (2/5) = 3/5
So the fraction of people in the room who are married men is 3/5 or 0.6
So, 60% of the people in the room are married men.
Learn more about probability:
https://brainly.com/question/30034780
#SPJ4
Find the probability of getting any triple-digit number where all the digits are the same in a lottery game that consists of selecting a three-digit number.
Answers
Thus, the probability is 1/100 or 0.01, which means there is a 1% chance of getting a triple-digit number with all the same digits in this lottery game.
To calculate the probability of getting a triple-digit number where all digits are the same in a lottery game that consists of selecting a three-digit number, you should first determine the number of favorable outcomes and the total possible outcomes.
There are 9 favorable outcomes, as the triple-digit numbers with the same digits are: 111, 222, 333, 444, 555, 666, 777, 888, and 999. Note that we don't include 000 since it's not a triple-digit number.
Now, let's find the total possible outcomes. In a three-digit number, there are 10 possible digits (0-9) for each of the three positions. However, the first position cannot be 0, as that would not be a triple-digit number.
So, there are 9 possible digits for the first position and 10 for the other two. The total possible outcomes are 9 x 10 x 10, which equals 900.
Finally, to find the probability of getting a triple-digit number with all the same digits, divide the number of favorable outcomes by the total possible outcomes:
Probability = Favorable Outcomes / Total Possible Outcomes
Probability = 9 / 900
The probability is 1/100 or 0.01, which means there is a 1% chance of getting a triple-digit number with all the same digits in this lottery game.
Know more about the probability
https://brainly.com/question/23417919
#SPJ11
PLEASE HELP ASAP
graph
graph
graph
graph
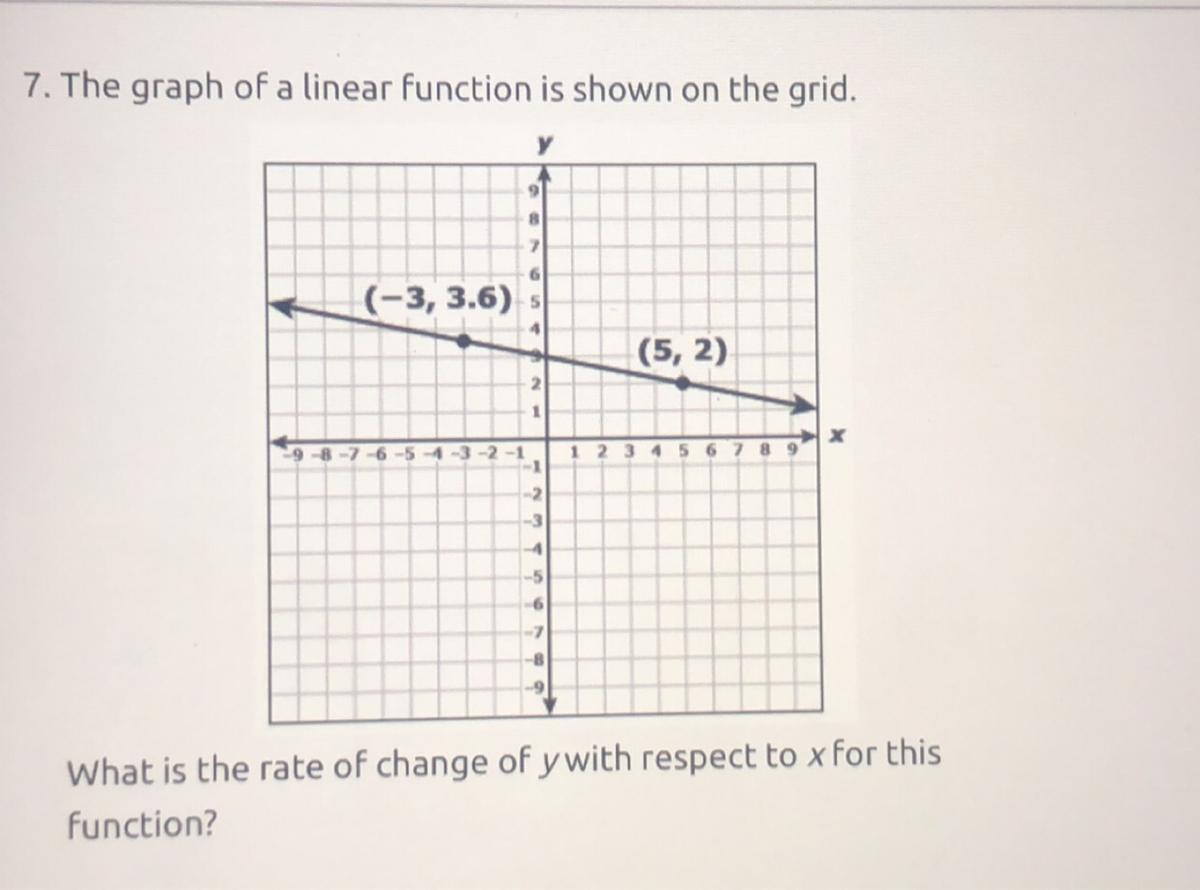
Answers
Answer: it’s 1/5
Step-by-step explanation:
I dont get the question

Answers
Las 2:3 partes de Los empleados de una empresa automotriz trabajan en ensambladura de autopartes; 1/8 parte , en el área de lavado; 1/6 parte, en el área de atención a clientes, y el resto son ejecutivos. Si en dicha empresa y 25 ejecutivos cuántos empleados hay en total
Answers
There are a total of 600 Employees in the automotive company.
The total number of employees in the automotive company, we need to determine the proportion of employees working in each area.
Let's denote the total number of employees as "x". According to the given information:
- The proportion of employees working in auto parts assembly is 2/3 of the total. So, the number of employees in auto parts assembly is (2/3)x.
- The proportion of employees working in the washing area is 1/8 of the total. So, the number of employees in the washing area is (1/8)x.
- The proportion of employees working in the customer service area is 1/6 of the total. So, the number of employees in the customer service area is (1/6)x.
- The rest of the employees are executives, and the number of executives is given as 25.
We can set up an equation to represent the total number of employees:
(2/3)x + (1/8)x + (1/6)x + 25 = x
To solve this equation, we can simplify and solve for x:
[(16/24)x + (3/24)x + (4/24)x] + 25 = x
(23/24)x + 25 = x
25 = x - (23/24)x
25 = (1/24)x
x = 24 * 25
x = 600
Therefore, there are a total of 600 employees in the automotive company.
For more questions on Employees .
https://brainly.com/question/31553508
#SPJ8
choose the table thst represents g(x) =-2•f(x) when f(x) = x +4

Answers
Answer:
c
Step-by-step explanation:
x=1 g(x)=-10
x=2 g(x)=-12
x=3 g(x)=-14
f(x)=x+4
=> g(x)=-2·(x+4)
Explain how to compare decimals use the numbers 6. 75 and 6. 732 to help you explain this process
Answers
6.75 is greater than 6.732
What are decimal numbers?
The accepted method for representing both integer and non-integer numbers is the decimal numeral system. It is the expansion of the Hindu-Arabic numeral system to non-integer values. Decimal notation is the term used to describe the method of representing numbers in the decimal system.
Here, we have
Given
6.75 and 6.732
We have to compare two given decimal numbers.
First, we convert to a decimal number
In 6.75 there are two digits after decimal point.
In 6.732, there are three digits after the decimal point
Therefore, we put zero in 6.75 to convert like a decimal number
Now, we get
6.750
The unit place of both decimal numbers are the same and the tenth place of both decimal number are same.
Now, the hundredth place of 6.750 is 5, and the hundredth place of 6.732 is 3
5 >3
Hence, 6.750 is greater than 6.732
To learn more about decimal numbers from the given link
https://brainly.com/question/28393353
#SPJ4
To clean a tank 3/4 litre of disinfectant is needed for every 6 litres of water. How many litres of disinfectant are needed for 42 litres of water?
Answers
There is need of 5.25 litres of disinfectant for 42 litres of water.
How to find disinfectant for 42 litres of water?To clean a tank with 42 litres of water, we need to determine how much disinfectant is required, given that 3/4 litre of disinfectant is needed for every 6 litres of water. Using the given ratio, we can set up a proportion to solve for the unknown quantity of disinfectant required. Cross-multiplying and simplifying, we find that 5.25 litres of disinfectant are needed to clean 42 litres of water. It is important to accurately measure and mix the disinfectant to ensure effective cleaning and sanitation. Following proper cleaning protocols and using appropriate amounts of disinfectant can help prevent the spread of harmful pathogens and maintain a safe and healthy environment.
Learn more about disinfectant
brainly.com/question/14210716
#SPJ11
Anya has $25,000 which she recently received from a trust fund, which she intends to invest in an account earning 12% annually. a) How many years would it take Anya to accumulate $40,000. b) If Anya's goal is to save $40,000 in just 3 years, what rate of return must she earn annually on her account. Show all workings and formulae
Answers
a) It would take Anya approximately 4 years to accumulate $40,000 with an annual interest rate of 12%. b) Anya must earn an annual rate of return of approximately 12.6% to save $40,000 in 3 years.
a) To calculate the number of years it would take Anya to accumulate $40,000, we can use the future value formula for compound interest:
Future Value = Present Value * (1 + interest rate)ⁿ
Where:
Future Value = $40,000
Present Value = $25,000
Interest rate = 12% = 0.12
n = number of years
Substituting the given values into the formula, we have:
$40,000 = $25,000 * (1 + 0.12)ⁿ
Dividing both sides of the equation by $25,000, we get:
(1 + 0.12)ⁿ = 40,000 / 25,000
(1.12)ⁿ = 1.6
To solve for n, we can take the logarithm of both sides of the equation:
n * log(1.12) = log(1.6)
Using a calculator, we find that log(1.12) ≈ 0.0492 and log(1.6) ≈ 0.2041. Therefore:
n * 0.0492 = 0.2041
n = 0.2041 / 0.0492 ≈ 4.15
b) To calculate the required rate of return for Anya to save $40,000 in just 3 years, we can rearrange the future value formula:
Future Value = Present Value * (1 + interest rate)ⁿ
$40,000 = $25,000 * (1 + interest rate)³
Dividing both sides of the equation by $25,000, we have:
(1 + interest rate)³ = 40,000 / 25,000
(1 + interest rate)³ = 1.6
Taking the cube root of both sides of the equation:
1 + interest rate = ∛1.6
Subtracting 1 from both sides, we get:
interest rate = ∛1.6 - 1
Using a calculator, we find that ∛1.6 ≈ 1.126. Therefore:
interest rate = 1.126 - 1 ≈ 0.126
To express the interest rate as a percentage, we multiply by 100:
interest rate = 0.126 * 100 = 12.6%
To know more about annual interest rate,
https://brainly.com/question/33696451
#SPJ11
which equation can be used to find t the total price of the feed?
Answers
Answer:
t=p(unit pf weight)
Step-by-step explanation:
t=plbs
total price is the price time the lbs of feed
(unit of weight may change) depending on question
Consider the following. x = e^t, y = e^(−3t)
(a) Eliminate the parameter to find a Cartesian equation of the curve.
(b) Sketch the curve and indicate with an arrow the direction in which the curve is traced as the parameter increases.
Answers
To indicate the direction in which the curve is traced as the parameter increases, we can draw an arrow that points from lower left to upper right, since the curve moves from the point (1, 1) at t = 0 to the right and upward as t increases.
To eliminate the parameter, we can use the fact that x = e^t and y = e^(-3t) to solve for t in terms of x and y. Taking the natural logarithm of both sides of x = e^t, we get ln(x) = t. Similarly, taking the natural logarithm of both sides of y = e^(-3t), we get ln(y) = -3t, or t = (-1/3)ln(y). Substituting this expression for t into the equation we found for ln(x), we get ln(x) = (-1/3)ln(y), which simplifies to ln(x^3) = ln(y^(-1)), or x^3 = 1/y. This is the Cartesian equation of the curve.
To sketch the curve, we can start by noting that both x and y are positive for all values of t, since e^t and e^(-3t) are always positive. As t increases, x and y both increase, but y increases much more slowly than x since e^(-3t) decreases rapidly as t increases. This means that the curve starts out very steep (since the slope of the tangent line at t = 0 is dx/dt = 1 and dy/dt = -3), but becomes flatter and flatter as t increases. The curve approaches the x-axis as t approaches infinity, but never touches it.
Know more about logarithm here:
https://brainly.com/question/30085872
#SPJ11
convert American $553 in Nepal rupees
Answers
Answer:
65,994.52 Nepalese Rupee
Step-by-step explanation:
using conversion rate 1 usd = 119.74 nepalese rupee
553*119.74
can i get answers pllzzzz

Answers
Answer:
C jsdjdjrishbxcbdjwiausus sorry I need to put the 20 words or.morrr
it's C
two differences between hybris fruits and local fruits.
Answers
Answer:
Native vegetable varieties are being organically cultivated by local farmers for hundreds of years. It is always recommended to regularly consume native vegetables where you belong to. Hybrids on the other hand are superior varieties of same vegetable species artificially crossed & developed by humans.
Step-by-step explanation:
divide 6x3 ÷3x how to do please tell me
Answers
Answer:
hey your answer would be 3x • (2x2 - 1)
please tell me if im wrong
Step-by-step explanation:
If Judy uses 400 chocolate chips to make x cookies, write an expression that represents the number of chocolate chips in each cookie.
Answers
Answer:
y=\(\frac{400}{x}\)
Step-by-step explanation:
Answer: 400/x (over x} = Y {amount on each cookie}
Step-by-step explanation:
a cone with volume 2880 m³ is dilated by a scale factor of 14. what is the volume of the resulting cone? enter your answer in the box.
Answers
For a cone with a volume 2880 m³, which is dilated by a scale factor of 14, the volume of the resulting cone is=2,744,832 m³.
The original volume of the cone is 2880 m³. It is given that the cone is dilated by a scale factor of 14.
The formula to find the volume of a cone is
V = (1/3)πr²h.
We know that the volume of a cone depends on the radius and height. When a cone is dilated by a scale factor, both the radius and height are multiplied by that scale factor.
So, the new volume can be calculated using the following formula:
New Volume = (scale factor)³ x (original volume)
Substituting the given values, we get:
New Volume = 14³ × 2880
New Volume = 2,744,832 m³
Therefore, the volume of the resulting cone is 2,744,832 m³.
To know more about volume, visit:
https://brainly.com/question/29767724
#SPJ11
A study found that 12% of dog owners brush their dog’s teeth. Of 493 dog owners, about how many would be expected to brush their dog’s teeth?
Answers
Answer:
about 59 dogs
Step-by-step explanation:
helloo!
total: 493
percentage: 12%
to find this, you could divide by 100 to find one percent of it and multiply by 12
so, 493/100=4.93
this is 1% of dogs.
so, to find 12% we would do 4.93*12 which is 59.16
hope this helped you :)
What is the difference between a repeating decimal and a terminating decimal
Answers
Answer:
Terminating decimals don't have a continuation of the same digits after the decimal point. Recurring decimals have one or more repeating numbers or sequences of numbers after the decimal point, which continue's forever.
Step-by-step explanation:
6(3)/(4)n-1(5)/(12)n help pls
Answers
Answer: To simplify the expression, you can first simplify the terms inside the parentheses.
6(3) = 18
(4)n-1(5)/(12)n = (4n-5)/(12n)
Then you can combine the like terms:
18/(4n-5)/(12n) = (18 * 12n)/(4n-5)
This is the simplified form of the original expression.
Step-by-step explanation:
Solve the equation cos x - xe =0 in the interval [0,1]. Use the results of the bisection method in the 10th iteration. O 0.617 O 0.517 O 0.527 O none of the choices
Answers
After the 10th iteration, the estimated value of the root obtained by the bisection method is approximately 0.517. Option B
To solve the equation cos(x) - x * e = 0 in the interval [0, 1] using the bisection method, we start by checking the function values at the endpoints of the interval.
For x = 0:
cos(0) - 0 * e = 1 - 0 = 1
For x = 1:
cos(1) - 1 * e ≈ 0.54 - 2.72 ≈ -2.18
Since the function values at the endpoints have opposite signs, we can apply the bisection method to find the root within the interval.
The bisection method involves repeatedly dividing the interval in half and checking the function value at the midpoint until a sufficiently accurate approximation is obtained. In this case, we will perform 10 iterations.
After the 10th iteration, the estimated value of the root obtained by the bisection method is approximately 0.517.
Therefore, the correct answer is OB) 0.517.
For more such questions on estimated value visit:
https://brainly.com/question/24239771
#SPJ8
Write the equation for a parabola with a focus at (−4,8)(-4,8)(−4,8)left parenthesis, minus, 4, comma, 8, right parenthesis and a directrix at x=−6x=-6x=−6x, equals, minus, 6.
Answers
Given:
The focus of the parabola is at (-4,8).
The directrix is at x=-6.
To find:
The equation of the parabola.
Solution:
The directrix is at x=-6, which is a vertical line. So, the parabola is horizontal.
The equation of a horizontal parabola is
\((y-k)^2=4p(x-h)\)
Where, (h,k) is vertex, (h+p,k) is focus and x=h-p.
The focus of the parabola is at (-4,8).
\((h+p,k)=(-4,8)\)
\(h+p=-4\) ...(i)
\(k=8\)
The directrix is at x=-6.
\(h-p=-6\) ...(ii)
Adding (i) and (ii), we get
\(2h=-10\)
\(h=-5\)
Putting h=-5 in (i), we get
\(-5+p=-4\)
\(p=-4+5\)
\(p=1\)
Putting h=-5, k=8 and p=1 in the standard form of the parabola.
\((y-8)^2=4(1)(x-(-5))\)
\((y-8)^2=4(x+5)\)
Therefore, the required equation of the parabola is \((y-8)^2=4(x+5)\).
Answer:
x=\(\frac{(y-8)^{2}-20 }4}\)
Step-by-step explanation:
Khan Academy:

NEED HELP!!! 30 POINTS
FIND THE RATIONAL EXPONENT FORM!!

Answers
Answer:
Step-by-step explanation:
The root which is 2 is the fractional portion of your exponent
\(\sqrt{x^{7} y^{12} }\)
=\((x^{7} y^{12} )^{\frac{1}{2} }\) >You can distribute 1/2 to each term by multiplying
\(=x^{\frac{7}{2} } y^{6\)
Anita está asiendo pan de calabaza y tiene medio galón de masa ella planea verter la masa en un molde de vidrio con longitud de 9 pulgadas un ancho de 4 y una profundidad de cuatro determine si toda la masa cabra en el sartén
Answers
All the batter would fit into the pan as it has more volume than half a gallon.
What is a cuboid?A cuboid is a three-dimensional closed figure which has volume along with surface area.
The volume of a cuboid is the product of its length, width, and height.
The total surface area of a cuboid is 2(lw + wh + wl) and the lateral surface area is 2(l + w)×h.
We know, One gallon is equal to 231 cubic inches.
Therefore, Half a gallon is equal to 231/2 cubic inches.
The volume of the pan is (9×4×4) cubic inches.
= 144 cubic inches.
Now, 144 > 231/2, so it would fit.
Q. Anita is baking pumpkin bread and has a half gallon of batter. She plans to pour the batter into a glass pan with a length of 9 inches, a width of 4 inches, and a depth of four. Determine if all the batter will fit in the pan.
learn more about cuboids here :
https://brainly.com/question/29424737
#SPJ9
(1 point) Determine whether each first-order differential equation is separable, linear, both, or neither.
1. dy/dx + exy = x2y2
2. y+ ex *sinx = x3 y'
3. ln x - x2y = xy'
4. dy/dx + cos y = tan x
Expert
Answers
Out of the given differential equations, only equation 3 is separable, and equation 4 is linear. The rest are nonlinear and neither separable nor linear.
The first-order differential equation dy/dx + exy = x2y2 is neither separable nor linear. It is a nonlinear ordinary differential equation. The presence of the term x2y2 in the equation makes it nonlinear, and the term exy makes it non-separable.
The differential equation y + ex * sin(x) = x3y' is neither separable nor linear. It is a nonlinear ordinary differential equation. The presence of the term ex * sin(x) and the term y' (derivative of y) make it nonlinear, and the term y makes it non-separable.
The differential equation ln(x) - x2y = xy' is separable but not linear. The terms ln(x) and x2y make it nonlinear, but it can be separated into two parts, one containing x and y and the other containing x and y'. Therefore, it is separable.
The differential equation dy/dx + cos(y) = tan(x) is linear but not separable. The terms cos(y) and tan(x) make it nonlinear, but it can be written in the form dy/dx + P(x)y = Q(x), where P(x) = cos(y) and Q(x) = tan(x). Therefore, it is a linear differential equation.
To learn more about differential equations click here : brainly.com/question/31492438
#SPJ11
What is the y-intercept of the graph of y = 2.5'?
a. 2.5
b. 1
a
C.
0
d. -1
PESERVED
Answers
Answer:
a. 2.5..... I believe but you should get other answers
help me plz
you will be a life saver

Answers
Answer:
2/7 , 7/13 ,5/9,10/11
Step-by-step explanation:
5/9 , 7/13 , 2/7, and 10/11
5/9 = 0.555
7/13≅ 0.54
2/7 ≅0.29
10/11=0.90
ascending order means from least to greatest:
2/7 , 7/13 ,5/9,10/11
simplify:29√3(3 – 1)
Answers
29/3(2)
29/(6)
29/6
Help ASAP !!!!! Which number line represents the solutions to \x + 4) = 2?
+
+
-7 -6 -5 -4 -3 -2 -1
0
1
2
3
+
+
0
1
2
3
-7 -6 -5 -4 -3 -2 -1
1
2
3
-7
-6 -5 -4 -3 -2 -1
+
1
2 3
О
-7 -6 -5 -4 -3 -2 -1 0

Answers
Answer:
first option
Step-by-step explanation:
Given
| x + 4 | = 2
The absolute value function always produces a positive value but the expression inside can be positive or negative, thus
x + 4 = 2 ( subtract 4 from both sides )
x = - 2
OR
- (x + 4) = 2, distribute left side
- x - 4 = 2 ( add 4 to both sides )
- x = 6 ( multiply both sides by - 1 )
x = - 6
Thus solutions are x = - 2, x = - 6
These are represented on the graph by solid blue circles at x = - 2, x = - 6
The first option represents the solution
HELPP GEOMETRY HOMEWORK

Answers
All of the angles in an acute triangle are less than 90 degrees. Triangles are referred to as right triangles when one of their angles is 90 degrees.
What is called triangle?The three vertices of a triangle make it a three-sided polygon. The angles of the triangle are formed by the three sides' end-to-end connections at a point. 180 degrees is the sum of the triangle's three angles. With three sides and three vertices, triangles are a particular kind of polygon in geometry.
It has three straight sides and is a two-dimensional figure. As a 3-sided polygon, a triangle is included. Three triangle angles added together equal 180 degrees. In one plane, the triangle is contained. When three straight lines cross, a triangle is the result. There are always three sides and three corners to a triangle (angles). A triangle's vertex is the location at which two of its sides meet.
To learn more about triangle refer to :
https://brainly.com/question/1058720
#SPJ1