What do we mean by integral?
Answers
In mathematics, an integral is either a number representing the region under a function's graph for a certain interval or a new function, the derivative of which is the original function (indefinite integral).
What is integral in math?The value obtained after integrating or adding the terms of a function that is divided into an infinite number of terms is generally referred to as an integral value.
Integral is a term derived form the word integration. Integration in calculus is the opposite of differentiation
Integration is used to get the whole by combining combine slices or smaller portions.
Finding areas, volumes, central points, and many other important things may be done with integration. However, it is simplest to begin by calculating the distance between a function and the x-axis.
Learn more about integral at:
https://brainly.com/question/27419605
#SPJ1
Related Questions
Which of the following correlation coefficients represents the strongest relationship between two variables? -.75 +.60 .00 +.30
Answers
The correlation coefficient that represents the strongest relationship between two variables is -0.75.
In correlation coefficients, the absolute value indicates the strength of the relationship between variables. The strength of the association increases with the absolute value's proximity to 1.
The maximum absolute value in this instance is -0.75, which denotes a significant negative correlation. The relevance of the reverse correlation value of -0.75 is demonstrated by the noteworthy unfavorable correlation between the two variables.
To know more about correlation coefficients, visit,
https://brainly.com/question/4219149
#SPJ4
Let P2 span{x², , 1} be a subspace of C|0,2), continuous function over (0,21 a Show that the evaluation map Ev: L + R}, L(P()) (PCO) p(1) p(2) is invertible. b Show that there are numbers 20, 21, 22 such that the quadrature formula 5. () + (2) p(c)dt = fop(0) + ap(1) + a2p(2) holds for any polynomial p in P2. Find the explicit values of ao, 21, 22. c. Does the quadrature formula holds for every function C|0,2]? Justify your answer.
Answers
The problem involves a subspace P2 of continuous functions over the interval (0, 2). It requires showing that the evaluation map Ev is invertible, determining coefficients for a quadrature formula, and discussing whether the formula holds for every function in C|0,2).
a) To show that the evaluation map Ev is invertible, we need to demonstrate that it is both injective (one-to-one) and surjective (onto). Injectivity means that different functions in P2 map to different points in R², and surjectivity means that every point in R² has a pre-image in P2. By examining the properties of P2 and the evaluation map, we can prove its invertibility.
b) The problem asks to find specific coefficients a₀, a₁, and a₂ that satisfy the given quadrature formula. We need to solve the equation ∫(0 to 2) [a₀ + a₁p(1) + a₂p(2)]p(c) dt = f₀p(0) + f₁p(1) + f₂p(2) for all polynomials p in P2. By substituting specific values for c and solving the resulting equations, we can determine the explicit values of a₀, a₁, and a₂.
c) The question inquires whether the quadrature formula holds for every function in C|0,2]. To justify our answer, we need to analyze the properties of the quadrature formula and determine if it provides accurate approximations for any arbitrary function in C|0,2]. This analysis involves considering the convergence properties of the formula and whether it can accurately capture the behavior of functions beyond polynomials.
The explanation provides an overview of the problem's components, including showing invertibility, finding coefficients for the quadrature formula, and discussing its applicability to functions in C|0,2]. It emphasizes the need for proofs, equations, and analysis to support the conclusions. The word count exceeds the minimum requirement of 100 words to provide a comprehensive explanation of the problem.
Learn more about arbitrary functions here:- brainly.com/question/31772977
#SPJ11
help me solve this pls because i need to solev this fast help me

Answers
The value of K that will make the expressions equivalent is 10.
How to evaluate the equivalent expression for the value of KConsidering the two expressions:
7 + (1/2)x - 3 + (3/4)x and 1 whole number (1/4)x + k - 6
we shall write the expression 7 + (1/2)x - 3 + (3/4)x in the form of the expression having k and then compare to know the value of k as follows;
(1/2)x + (3/4)x = (2x + 3x)/4
(1/2)x + (3/4)x = 5x/4
7 - 3 = 10 - 3 - 3
7 - 3 = 10 - 6
so;
7 + (1/2)x - 3 + (3/4)x = 5x/4 + 10 - 6
by comparison;
5x/4 is equal to the mixed fraction 1 whole number (1/4)x
k = 10 as obviously -6 is equal to -6.
Therefore, 10 is the value of k that will make expressions equivalent.
Know more about equivalent expression here: https://brainly.com/question/24734894
#SPJ1
A system of linear equations is shown in the graph. How many solutions does the system have? a coordinate plane with one line that passes through the points 0 comma 3 and 3 comma 4 and another line that passes through the points 0 comma negative 2 and 3 comma negative 1 No solution One solution at (0, 3) One solution at (−2, 0) Infinitely many solutions
Answers
The two lines are parallel, then the system of equations has no solutions.
How to solve the system of equations?A linear equation can be written as:
y = a*x +b
Where a is the slope.
If the line passes through two points (x₁, y₁) and (x₂, y₂) then the slope is:
a = (y₂ - y₁)/(x₂ - x₁)
Here the first line passes through (0, 3) and (3, 4), so the slope is:
a = (4 - 3)/(3 - 0) = 1/3
And the y-intercept is 3:
y = (1/3)*x + 3
The other line passes through (0, -2)and (3, -1)
a = (-1 + 2)/(3 - 0) = 1/3
And the y-intercept is -2, so this line is:
y = (1/3)*x - 2
Then the system of equations is:
y = (1/3)*x + 3
y = (1/3)*x - 2
So both equations have the same slope and different y-intercept, thus, the lines are parallel, then the system of equations has no solutions.
Learn more about systems of equations.
https://brainly.com/question/13729904
#SPJ1
Find at least three different sequences beginning with the terms 3, 5, 7 whose terms are generated by a simple formula or rule.
Answers
Answer:
3 5,7,9,11,13,16,17 19,21,23,25,27,29
Express y = x^2+17 / (x+1)(6+x^2 + 5x) as a sum of partial fractions
Answers
The given rational function y = (x² + 17) / [(x + 1)(6 + x² + 5x)] can be expressed as a sum of partial fractions as y = (-31/2)/(x + 1) + (-31/2)x - 51/(x² + 5x + 6).
What is the fraction?
A fraction is a mathematical representation of a part of a whole, where the whole is divided into equal parts. A fraction consists of two numbers, one written above the other and separated by a horizontal line, which is called the fraction bar or the vinculum.
To express the rational function y = (x² + 17) / [(x + 1)(6 + x² + 5x)] as a sum of partial fractions, we need to decompose it into simpler fractions with denominators of lower degree. The partial fraction decomposition of y will have the following form:
y = A/(x + 1) + (Bx + C)/(x² + 5x + 6)
To find the values of A, B, and C, we can use the method of equating coefficients. We'll multiply both sides of the equation by the common denominator to eliminate the denominators:
(x + 1)(x² + 5x + 6)y = A(x² + 5x + 6) + (Bx + C)(x + 1)
Expanding the equation and collecting like terms:
(x³ + 6x² + 11x + 6 + 5x² + 30x + 36)y = Ax² + 5Ax + 6A + Bx² + Bx + Cx + C
Combining like terms:
(x³ + 11x² + 41x + 42)y = (A + B)x² + (5A + B + C)x + (6A + C)
Now, we equate the coefficients of like powers of x on both sides of the equation:
For x³: 0 = A + B
For x²: 1 = A + B
For x¹: 11 = 5A + B + C
For x⁰: 42 = 6A + C
From the equation A + B = 0, we find that A = -B.
From the equation 1 = A + B, substituting A = -B, we have 1 = -B + B, which is always true.
From the equation 42 = 6A + C, we can substitute A = -B to get 42 = -6B + C.
From the equation 11 = 5A + B + C, we substitute A = -B and simplify to get 11 = -5B + B + C, which simplifies to 11 = -4B + C.
Now we can solve the system of equations:
-6B + C = 42
-4B + C = 11
Subtracting the second equation from the first, we have:
-2B = 31
B = -31/2
Substituting B = -31/2 into -4B + C = 11, we can solve for C:
-4(-31/2) + C = 11
62 + C = 11
C = 11 - 62
C = -51
Now that we have the values of A = -B, B, and C, we can write the partial fraction decomposition:
y = (-31/2)/(x + 1) + (Bx + C)/(x² + 5x + 6)
= (-31/2)/(x + 1) + (-31/2)x - 51/(x² + 5x + 6)
Therefore, the given rational function y = (x² + 17) / [(x + 1)(6 + x² + 5x)] can be expressed as a sum of partial fractions as y = (-31/2)/(x + 1) + (-31/2)x - 51/(x² + 5x + 6).
To learn more about the fraction visit:
brainly.com/question/30154928
#SPJ4
5√27-17√3 in radical form
Answers
Answer:
\(-2\sqrt{3}\)
Step-by-step explanation:
\(5\sqrt{27} -17\sqrt{3} =5*3\sqrt{3} -17\sqrt{3}\)
\(=15\sqrt{3} -17\sqrt{3}\)
=\(-2\sqrt{3}\)
need help with this problem

Answers
The solution to the equation is x = 0.
Option D is the correct answer.
We have,
The equation is:
(1 - 3x)^{1/3} - 1 = x
Let's start by isolating the radical term by adding 1 to both sides:
(1 - 3x)^(1/3) = x + 1
Next, we'll cube both sides to eliminate the radical:
[(1 - 3x)^(1/3)]^3 = (x + 1)^3
1 - 3x = (x + 1)^3
1 - 3x = x^3 + 3x^2 + 3x + 1
0 = x^3 + 3x^2 + 6x
Now we have a cubic equation, which we can solve by factoring out an x:
x(x^2 + 3x + 6) = 0
The quadratic factor doesn't have any real roots (since its discriminant is negative),
So the only solution is x = 0.
i.e
(1 - 3x)^(1/3) - 1 = 1^(1/3) - 1 = 0.
Thus,
The solution to the equation is x = 0.
Learn more about solutions to equations here:
https://brainly.com/question/545403
#SPJ1
9.) x2 - 6x + 25 = 0
Using the Quadratic Formula
Answers
Answer:
x = 25/4
Step-by-step explanation:
snuzjsbzhsisnzbxhsjnsbx
Compare the linear functions expressed below by data in a table and by an equation.
A 2-column table with 4 rows. Column 1 is labeled x with entries negative 6, negative four-thirds, 1, 4. Column 2 is labeled y with entries negative StartFraction 9 Over 2 EndFraction, negative 1, three-fourths, 3. y = three-fourths x.
Which of the following statements are true? Select all that apply.
If the equation were graphed, it would be a horizontal line.
Both functions have the same slope.
The origin is the y-intercept for the function expressed in the table.
The linear equation does not have a y-intercept.
The table and the graph express an equivalent function.

Answers
Answer:
b,c,e
Step-by-step explanation:
help meeeeeeeeeeeeeee pleaseeeeeee

Answers
Answer: 19.6 feet
Step-by-step explanation:
Using the Pythagorean theorem,
\(x^2 +(x+6)^2 =48^2\\\\x^2 +x^2 +12x+36=2304\\\\2x^2 +12x-2268=0\\\\x^2 +6x-1134=0\\\\x=\frac{-6 \pm \sqrt{6^2 -4(1)(-1134)}}{2(1)}\\\\x \approx 30.8 \text{ } (x > 0)\\\\\implies x+(x+6) \approx 67.6\\\\\therefore (x+(x+6))-48 \approx 19.6\)
if f(x)= -x^2 +10x + 3 and g(x) = -4x - 3x + 7 then g (x) - f (x) =
Answers
The value of operated function is -3x²-13x+4.
Given are two functions f(x) = -x²+10x+3 and g(x) = -4x²-3x+7, we need find g(x) - f(x),
So,
g(x) - f(x) = -4x²-3x+7 - (-x²+10x+3)
= -4x²-3x+7 + x²-10x-3
Combining the like terms,
= -4x²+x²-3x-10x+7-3
= -3x²-13x+4
Hence the value of operated function is -3x²-13x+4.
Learn more about function click;
https://brainly.com/question/27915566
#SPJ1
there is a 5 person round table. 5 different people sit down at the table. what are the odds that sue will sit next to bob?
Answers
The probability that Sue will sit next to Bob is 96/24 = 4. So, the odds are 4:1.
We need to find out the odds that Sue will sit next to Bob. There is a round table with five different people. Therefore, the total number of ways that five different people can be seated at a round table is (5 - 1)! = 4!.
Thus, there are 24 ways that these five different people can be seated around the table. Now, let's say Sue and Bob are sitting next to each other. Thus, there are 4! = 24 ways in which they can be seated around the table. Now, Sue and Bob can be treated as a single unit since they are sitting together. So, there are a total of 48 + 48 = 96 possible ways that Sue will sit next to Bob in a 5 person round table. There are 24 possible ways that 5 people can be seated around the table.
To learn more about probability, visit:
https://brainly.com/question/32117953
#SPJ11
first change the given pattern into a vector of zeros and ones by turning each shaded square into a 1 and each unshaded square into a 0 and then lining up each column below the column before it. then find a matrix m so that m0 but m0 for all other nonzero vectors of zeros and ones.
Answers
The pattern will be attached in the figure attached, in the matrix form.
What is the matrix?
The arrangements of numbers, variables, symbols, or phrases in a rectangular table with varying numbers of rows and columns are known as matrices, which is the plural version of the word matrix. They are rectangular arrays with defined operations for addition, multiplication, and transposition. The constituents of the matrix are its numbers or entries. Vertical entries in matrices are referred to as columns, whereas horizontal entries are known as rows. A matrix is a rectangular array of numbers, variables, symbols, or expressions that are defined for operations like subtraction, addition, and multiplication. The number of rows and columns in a matrix determines its size, sometimes referred to as its order.
We can arrange it as A = (0 0 0 0 1 1 0 0 1)
We know A*TA = 0
If A has an entry 9 then, the sum of all the diagonal elements.
So the T will be the form of the matrix attached below.
Hence the Pattern will be attached in the figure attached.
Learn more about matrix, by the following link
brainly.com/question/4030813
#SPJ4
I NEED HELP PLS I WILL GIVE CROWN: Mr. Crow, the head groundskeeper at High Tech Middle School, mows the lawn along the side of the gym. The lawn is rectangular, and the length is 5 feet more than twice the width. The perimeter of the lawn is 250 feet.
Answers
Answer:
Mr. Crow dimension of the lawn = 85 × 40
Area of the lawn = 3250 square feet
Step-by-step explanation:
Let x represents the width of the rectangle,
Then According to the question,
The length of the rectangle = 2 x + 5
Also it is given that the perimeter of the rectangle = 250 feet.
But we know that the perimeter of the rectangle = 2 × ( length + width)
Thus, the perimeter of the rectangle = 2( 2x+5+x) = 2(3x+5) = 6x+10
If x = 30, the perimeter of the rectangle = 6 × 30 + 10 = 190 < 250
Thus, x ≠ 10.
If x = 50, the perimeter of the rectangle = 6 × 50 + 10 = 310 > 250
Thus, x ≠ 50
If x = 40, the the perimeter of the rectangle = 6 × 40 + 10 = 250 = 250
Thus, x = 40
Therefore, the width of the rectangle, x = 40 feet.
And, the length of the rectangle, 2 x + 5 = 2 × 40 + 5 = 85 feet.
1) The dimension of the rectangle = 85 × 40
2)The area of the rectangle = length × width = 85 × 40 = 3250 square feet.Let x represents the width of the rectangle,
Then According to the question,
The length of the rectangle = 2 x + 5
Also it is given that the perimeter of the rectangle = 250 feet.
But we know that the perimeter of the rectangle = 2 × ( length + width)
Thus, the perimeter of the rectangle = 2( 2x+5+x) = 2(3x+5) = 6x+10
If x = 30, the perimeter of the rectangle = 6 × 30 + 10 = 190 < 250
Thus, x ≠ 10.
If x = 50, the perimeter of the rectangle = 6 × 50 + 10 = 310 > 250
Thus, x ≠ 50
If x = 40, the the perimeter of the rectangle = 6 × 40 + 10 = 250 = 250
Thus, x = 40
Therefore, the width of the rectangle, x = 40 feet.
And, the length of the rectangle, 2 x + 5 = 2 × 40 + 5 = 85 feet.
1) The dimension of the rectangle = 85 × 40
2)The area of the rectangle = length × width = 85 × 40 = 3250 square feet.
A jar contains 19 coins consisting of quarters and dimes. The total value of the coins is $3.70. Determine the number of quarters and the number of dimes in the jar.
# of quarters-______________ # of dimes-______________
A large box of apricots cost $7 and a small box of apricots cost $4. Chad purchases a total of 26 large and small boxes. The total cost of the boxes is $146. How many large and small boxes did Chad purchase?
# of large boxes-_______________ # of small boxes-_______________
Answers
Answer:
# of quarters: 12 # of dimes: 7
# of large boxes: 14 # of small boxes: 12
Step-by-step explanation:
Problem 1: Dimes and Quarters
Let q represent the number of quarters
Let d represent the number of dimes
We have as a first equation
q + d = 19 (1)
Each quarter is worth $0.25 so q quarters worth = 0.25q
Each dime is worth $0.10 so d dimes worth = 0.10d = 0.1d
Total value of q quarters and d dimes
0.25q + 0.1d = 3.70 (2)
We have two equations in 2 variables which can be solved as follows
Multiply equation (2) by 4 so that the coefficients of q in both equations are the same, namely 14(0.25q + 0.1d) = 4 x 3.70
q + 0.4d = 14.8 (3)Subtract equation 3 from equation 1 to eliminate q term
q + d - (q + 0.4d) = 19 - 14.8
q + d - q - 0.4d = 4.2
0.6d = 4.2
d = 4.2/0.6 = 7Substitute this value of d in equation 1
q + 7 = 19
q = 19 - 7 = 12
Therefore there are 12 quarters and 7 dimes
Verify:
12 x 0.25 + 7 x 0.10 = 3.70
Problem 2: Apricots
Solution strategy is the same as Problem 1 so I am skipping lengthy explanations
Let L be the number of large boxes, S be the number of small boxes
We have
L + S = 26 (1)
Total cost of boxes
7L + 4S = 146 (2)
Multiply equation (1) by 4 :
4L + 4S = 4 x 26 = 104 (3)
Subtract (2) from (3):
7L + 4S - (4L + 4S) = 146 - 104
3L = 42
L = 42/3 = 14
Substitute in (1)
14 + S = 26
S = 26-14
S = 12
So there are 14 large and 12 small boxes
Check:
14 x 7 + 12 x 4 = 146
What are the steps to solve for x?
x+ax=b
Answers
Answer:
\(\displaystyle x = \frac{b}{1 + a}\)
General Formulas and Concepts:
Algebra I
Equality Properties
Multiplication Property of EqualityDivision Property of EqualityAddition Property of EqualitySubtraction Property of EqualityTerms/Coefficients
FactoringStep-by-step explanation:
Step 1: Define
Identify.
\(\displaystyle x + ax = b\)
Step 2: Solve for x
Factor: \(\displaystyle x(1 + a) = b\)[Division Property of Equality] Divide 1 + a on both sides: \(\displaystyle x = \frac{b}{1 + a}\)Chad is renting a moving van for one day. It costs $20 to rent the van for the day. It also costs $0.60 for each mile the van is driven. Chad uses this model to find the total cost for driving m miles: initial cost + cost for one mile × number of miles Which expression can be used to find the total cost of renting the van?
Answers
Answer:
20 + 0.6x
Step-by-step explanation:
Answer:
B (20 + 0.60m)
Step-by-step explanation:
1 tablespoon is equivalent to _____ ml.
Answers
Answer:
14.7868 ml are equal to 1 tablespoon
Step-by-step explanation:
1 tablespoon is equivalent to __14.7868___ ml.
Hope This Helped
Answer:
14.7868ml or 15 ml
Step-by-step explanation:
it can be either one since 14.7686 is close to 15
Which of the following statements are true? select all that apply.
Answers
Answer:
what are the statements?
65/5*2 equals what ?
Answers
Answer:
26.
Step-by-step explanation:
Answer:26
Step-by-step explanation:
65/5*2
65/5
13
13*2
26
H= 51.34
Please work out the volume of this.

Answers
The volume of the prism is
70 cm³How to find the volume of the prismThe volume of the prism is solved by the formula
= area of triangle * depth
Area of the triangle
= 1/2 base * height
base = p = cos 51.34 * √41 = 4
height = q = sin 51.34 * √41 = 5
= 1/2 * 4 * 5
= 10
volume of the prism
= area of triangle * depth
= 10 * 7
= 70 cm³
Learn more about volume of prism at
https://brainly.com/question/23766958
#SPJ1
1) Fill in the blank with True or False.

Answers
Answer:w
Step-by-step explanation:
i don’t understand.
online school is hard

Answers
Answer:
4. \(6\sqrt{3}\)
6. \(\frac{2\sqrt{6} }{3}\)
8. 23.78
What are the missing parts that correctly complete the proof?
Drag the answers into the boxes to correctly complete the proof.
(Please refer to the images provided for the answer options and equation image.)

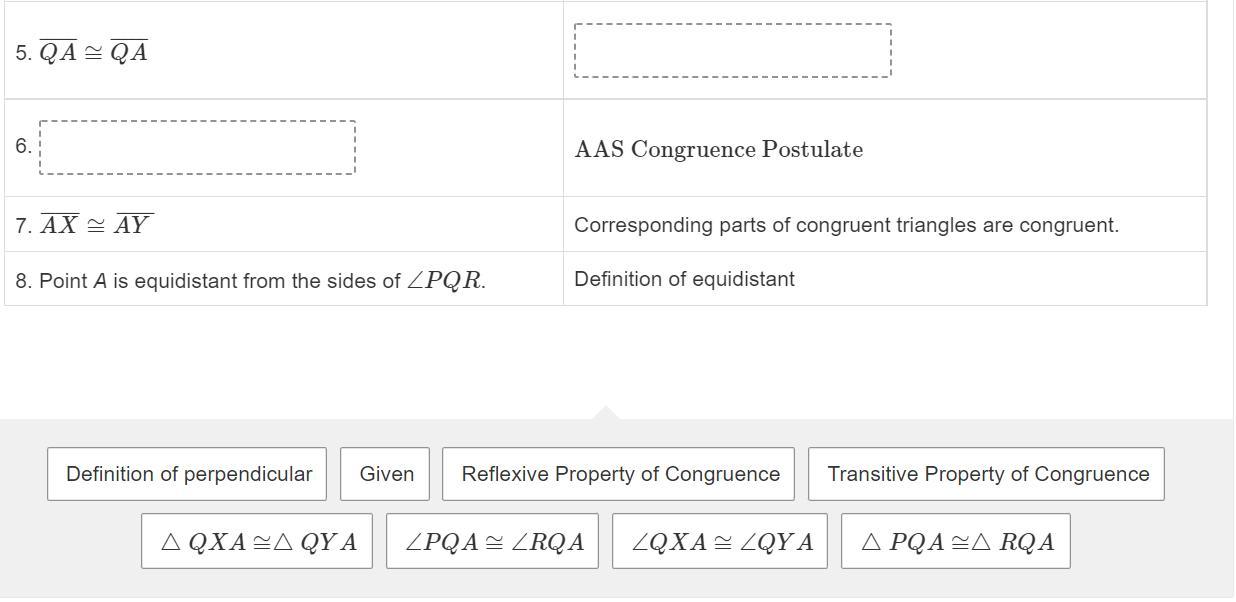
Answers
It has been proved that point A is equidistant from the sides of angle PQR.
What is a Bisector ?Any line or point that divides an angle or a side in equal parts is called a Bisector.
It is given that
Point A is the bisector of Angle PQR
Referring to the image
1. QA is the bisector of angle PQR : Given
2.Angle PQA = Angle RQA : Definition of Bisector
3.Angle QXA = Angle QYA : Definitions of Perpendicular
4. Angle QXA = Angle QYA : All right angles are congruent.
5. QA ≅QA : Reflexive Property of Congruence
6. ΔPQA ≅ΔRQA : AAS Congruence Postulate
7. AX ≅AY Corresponding parts of Congruent Triangles
8. Point A is equidistant from the sides of Angle PQR : Definition of equidistant.
To know more about Bisector
https://brainly.com/question/12896755
#SPJ1
please help its due today


Answers
The measure of the angles are: K, L, C, N, O G = -3/4, 65, 8 40.4, 89 and 44 respectively
How to find the measure of the angles?To determine K
9x + 2 = 23 + 5x -1
9x -5x = -1 +2
4x = -3Making x the subject we have
x= -3/4
To find the value of L
Let angle L be x
x + 65 = 130
x=130-65
That is x = 65
To find the m<C
4x + 7 = 3x + 15
Collecting like terms
4x - 3x = 15-7
Making x the subject of the relation we have
x=8
To find the m<N
7x + 6x - 1 = 90
13x = 89
x=89/13
x= 6.9
Substitute x = 6.9 in 6x -1
6(6.9) -1
m<L = 40.4
To find the m<O
5x - 11 = 4x +9
5x-4x = 9 +11
x=20
By substitution in 4x +9 we have
4(20) +9
80+9
m<S = 89
To find the value of the angle G
6x - 4 = 5x +4
6x - 5x = 4+4
x = 8
Therefore the m<G is
5x +4
5(8) +4
40+4 = 44 digress
Learn more about measure of angles in https://brainly.com/question/28451077
#SPJ1
You are playing a card game where the total points you earn depends on theasuits of the cards you draw. The total number of points is given by the expression3a + 3b-2c-2d, where a represents the number of hearts, b is diamonds, c isclubs, and d is spades. What is the total number of points you earn if you draw 3hearts, 2 diamonds and 1 spade? *
Answers
3a + 3b-2c-2d
a= number of hearts
b= number of diamons
c= number of clubs
d= number of spades
if you draw 3hearts, 2 diamonds, and 1 spade, just replace a=3, b=2, c=0, d=1
3(3) + 3(2)-2(0)-2(1)
Multiply each term and add the results:
9+6-0-2 = 13
13 points
In a particular chi-square goodness-of-fit test, there are six categories and 325 observations. Use the 0.10 significance level.
How many degrees of freedom are there?
What is the critical value of chi-square? (Round your answer to 3 decimal places.)
Answers
In this particular chi-square goodness-of-fit test with six categories, 325 observations, and a 0.10 significance level, there are 5 degrees of freedom and the critical value of chi-square is 9.236.
To find chi-square goodness-of-fit test question.
To determine the degrees of freedom in this particular chi-square goodness-of-fit test with six categories and 325 observations, you need to subtract 1 from the number of categories. So, the degrees of freedom are:
Degrees of Freedom (df) = Number of Categories - 1
Degrees of Freedom (df) = 6 - 1
Degrees of Freedom (df) = 5
Next, to find the critical value of chi-square at a 0.10 significance level and 5 degrees of freedom, you can use a chi-square distribution table or an online calculator. After consulting the table or calculator, you will find that the critical value of chi-square is:
Critical Value of Chi-Square (rounded to 3 decimal places) = 9.236
So, in this particular chi-square goodness-of-fit test with six categories, 325 observations, and a 0.10 significance level, there are 5 degrees of freedom and the critical value of chi-square is 9.236.
To learn more about chi-square
https://brainly.com/question/4543358
#SPJ11
Use the following system of equations to select the appropriate response.
Step 1 of the substitution Method to solve system of equations. use the second equation and solve for the x Variable.
A. x = y - 3
B. x = -y + 3
C. x = -y - 3
D. x = y + 3

Answers
Answer:
Step-by-step explanation:
Given system of equations is,
8x + 10y = 120 -----(1)
x - y = -3 ------(2)
From the second equation,
x = y - 3 [Option A]
Now we substitute the value of x in equation (1) and solve for y,
8(y - 3) + 10y = 120
8y - 24 + 10y = 120
18y = 120 - 24
y = \(\frac{96}{18}\) = 8
From equation (2)
x - 8 = -3
x = 8 - 3
x = 5
I will give brainliest

Answers
Answer:
Garage A- 3x12+10=46
Garage B- 4x12+3=51
Garage A is $5 cheaper than Garage B when parking for 12 hours
Step-by-step explanation:
You plug in the variables given to each garage into y=mx+b. Both Garage A and B have a constant (A-$10, B-$3). X in this equation would be the number of hours and m is the amount charged per hour.