What is the correct justification for the first step in solving this equation? 3(x - 4) + 1 = 12x
Answers
Answer:
x = -11/9
Step-by-step explanation:
3(x-4) + 1 = 12x
Step 1: Use distributive property
3*x - 3*4 + 1 = 12x
3x - 12 + 1 = 12x
Step 2: Combine like terms
3x - 11 = 12x
Step 3: Subtract '3x' from both sides
-11 = 12x - 3x
-11 = 9x
Step 4: Divide both sides by 9
-11/9 = x
Answer:
Solve the first part in Parenthese
Step-by-step explanation:
3(X-4)
3x-12+1=12x
-1 -1
3x-11-12=12x
-12 -12
3x-11=0x
-11 -11
3x= -11x
/3 /3
x= -11/9
Related Questions
Which of the following is parallel to the line 2x+4y=16
Answers
Answer:
Option C: y=-1/2x + 8
Step-by-step explanation:
So we have the options:
A) y=2x+5
B) y=-1/2x+4
C)y=-1/2x+8
D)y=-2x+5
But first let's define what parallel even is. When two lines are parallel it means that there slope is the same value and the same sign, while there y-intercepts are different, because if they were the same, then they wouldn't be parallel, they would just be the same exact line.
So we're given the equation in standard form. To find the slope we can change it so it's in the form of y=mx+b. This can be done by simply isolating y. The reason we want it in the slope-intercept form is because m represents the slope and b represents the y-intercept. m is the slope because as x increases by 1 the y-value will increase by m. So the "rise" will be m and the "run" will be 1, thus the slope will be m/1 or in other words m because the slope is defined as rise/run. So let's start the steps to isolating y
Original equation
2x+4y=16
Subtract 2x from both sides
4y=-2x+16
Divide both sides by 4
y = -1/2x + 4
Here we have it in slope-intercept form. In this case the slope, or m, is -1/2 and the y-intercept or b is 4. So now let's look at the other equations.
Option A: This equation has a slope of 2, which is not the same as -1/2 so it is not parallel
Option B: This equation has a slope of -1/2 which is the same as -1/2 so it might be parallel. But look at the y-intercept it's 4, that's the same y-intercept as the original equation. This means the two equations are equal and not parallel
Option C: This equation has a slope of -1/2 which is the same as -1/2 so it might be parallel. It has a y-intercept of 8 which is not the same as 4, so the two lines are parallel and not equal! This is the answer
Austin will run at least 33 miles this week. So far, he has run 20 miles. What are the possible numbers of additional miles he will run? Use t for the number of additional miles he will run.
Answers
Answer:
t ≥ 13
Step-by-step explanation:
t + 20 ≥ 33
subtract 20 from each side
he will run at least another 13 miles
Which of the following is equivalent to 5x+7y=-14?
y=5/7x-2
y=7/5x-2.8
y=-5/7x+2
y=-5/7x-2
Answers
The given equation is equivalent to y = -5x/7 - 2
Given that an equation, 5x+7y = -14, we need to find an equivalent equation for thus,
So,
5x+7y = -14
Minus 5x from both the sides
7y = -14 - 5x
Divide the equation by 7,
y = -5x/7 - 2
Hence the given equation is equivalent to y = -5x/7 - 2
Learn more about equation click;
https://brainly.com/question/29657983
#SPJ1
HELP PLEASE. Two points are 17 units apart on a number line. The coordinate of one point is -20.
What are the possible coordinates of the other?
Answers
Answer: -37 & -3
Step-by-step explanation:
-20-17= -37
-20+17= -3
The graph represents the number of hours that Walter sleeps in terms of the number of days

Answers
Note that the function that represents Walters' situation is y= 8x (Option D)
How is this so?The function y = 8x represents a linear relationship between the number of days (x) and the number of hours Walter sleeps (y).
It suggests that Walter's sleep duration increases by 8 hours for each additional day.
The graph of this function would be a straight line with a slope of 8, indicating a consistent increase in sleep hours as the number of days increases. The function assumes a positive correlation between the two variables.
Learn more about function at:
https://brainly.com/question/11624077
#SPJ1
Full Question:
The graph represents the number of hours that Walter sleeps in terms of the number of days.
Which function represents Walter’s situation?
A.) y= 6x
B.) y= 6
C.) y= 8
D.) y= 8x

Find M<1, M<2, M<3, M<4, M<5

Answers
Answer:
Step-by-step explanation:
m∠2 = 52° [Alternate interior angles]
m∠1 + m∠2 = 180° [Linear pair of angles are supplementary]
m∠1 = 180° - 52°
m∠1 = 128°
m∠3 = 47° [Alternate interior angles]
m∠5 + 52° + 47° = 180° [Sum of linear angles is 180°]
m∠5 = 180° - 99°
= 81°
m∠4 + 47° = 180° [Sum of consecutive interior angles is 180°]
m∠4 = 180° - 47°
m∠4 = 137°
You and your friends order food from a menu where each item costs the same amount. Write an expression in the simplest form that represents the total amount of money the order will costs.
4 sodas
1 milkshake
5 cheeseburgers
2 orders of chicken fingers
4 orders of french fries
Answers
Answer:
16x=y
Step-by-step explanation:
Since all of them are of equal cost, they can all be represented with the value of x. So basically, multiply x with the number of products (which is 16), then just make it equal to something to make it an expression (the total cost), which will be y, since it is unknown
to calculate the center line of a control chart you compute the ________ of the mean for every period.
Answers
The centre line of a control chart is calculated by computing the average (mean) of the data for every period.
In control chart analysis, the centre line represents the central tendency or average value of the process being monitored. It is typically obtained by calculating the mean of the data points collected over a specific period. The purpose of the centre line is to provide a reference point against which the process performance can be compared. Any data points falling within acceptable limits around the centre line indicate that the process is stable and under control.
The calculation of the centre line involves summing up the values of the data points and dividing it by the number of data points. This average is then plotted on the control chart as the centre line. By monitoring subsequent data points and their distance from the centre line, deviations and trends in the process can be identified. Deviations beyond the control limits may indicate special causes of variation that require investigation and corrective action. Therefore, the centre line is a critical element in control chart analysis for understanding the baseline performance of a process and detecting any shifts or changes over time.
To learn more about mean refer:
https://brainly.com/question/20118982
#SPJ11
I need help on how to do this ASAP please

Answers
Step-by-step explanation:
Solve for x
(3x + 94) + (x + 30) + (2x - 4) = 180
(3 + 1 + 2)x = 180 - (94 + 30 - 4)
6x = 60
x = 60/6
x = 10°
Solution → x = 10°
__________
\( \: \)
3x + 94 + x + 30 + 2x - 4 = 180
(3x + 1x + 2x) + (94 + 30 - 4) = 180
6x + 120 = 180
6x = 180 - 120
6x = 60
x = 10
Is (–39, 42) a solution to the equation y = x + 81?
Answers
Answer:
Yes
Step-by-step explanation:
Yes because if you insert -39 for x and 42 for y then your equation is:
42 = -39 + 81
If you were to solve this equation it would be true.
Help me
Line ℓ is perpendicular to line m. Find the value of x and w.

Answers
w° = 180° - 138°
w = 42
19° + x° + w° = 90°
sub. in w=42,
19° + x° + 42° = 90°
x° = 90° - 19° - 42°
x° = 29°
x = 29
At what rate has she been making deposits to her savings?

Answers
Answer:
20 dollars per month
Step-by-step explanation:
85 = 25 + x3
60 = x3
20 = x
In problems 3–18, use the elimination method to find a general solution for the given linear system, where differentiation is with respect to t.
dx/dt + y = t^2
-x + dy/dt=1
Answers
To use the elimination method, we want to eliminate one of the variables (either x or y) by adding or subtracting the two equations. Let's eliminate x by multiplying the first equation by -1 and adding the two equations:
-x dt/dt - y = -t^2
-x + dy/dt = 1
--------------------
dy/dt - y = -t^2 + 1
This is a first-order linear differential equation, which we can solve using an integrating factor. The integrating factor is e^(-t), so we multiply both sides by e^(-t):
e^(-t) dy/dt - e^(-t) y = -t^2 e^(-t) + e^(-t)
The left side can be written as the derivative of (e^(-t) y), so we integrate both sides:
e^(-t) y = ∫(-t^2 e^(-t) + e^(-t)) dt + C
e^(-t) y = (t^2 - 1) e^(-t) + C
y = t^2 - 1 + Ce^t
Now we can substitute this expression for y into either of the original equations to find x. Let's use the first equation:
dx/dt + y = t^2
dx/dt + t^2 - 1 + Ce^t = t^2
dx/dt = 1 - Ce^t
x = t - Ce^t + D
So the general solution to the linear system is:
x = t - Ce^t + D
y = t^2 - 1 + Ce^t
where C and D are constants determined by initial conditions.
Find more about elimination method
brainly.com/question/31266268
#SPJ11
Graph y = -1/3x + 5
khan academy
Answers
Graph the line using the slope and y-intercept, or two points.
Slope:
−1/3
y-intercept:
(0, 5)
x y
0 5
15 0
HELP NOW FOR MEGA POINTS
Which statement below about the graph of f(x)=-log(x+4)+2 is true?
1) f(x) has a y-intercept at (0,2)
2) −f(x) has a y-intercept at (0,2)
3) As x → ∞, f(x) → ∞.2)
4) x → −4, f(x) → ∞
SHOW WORK
Answers
Answer:
4 IS THE ANSWER MATE
Step-by-step explanation:
Absolutely, I can do that!
Let's take a look at each statement:
1) f(x) has a y-intercept at (0,2)
To find the y-intercept, we need to set x to 0 and solve for y. Plugging in x = 0 into the equation for f(x), we get:
f(0) = -log(0+4) + 2
f(0) = -log(4) + 2
f(0) = -0.602 + 2
f(0) = 1.398
Since the y-coordinate of the y-intercept is 1.398, not 2, this statement is false.
2) The function -f(x) has a y-intercept at (0,2)
Since the negative sign in front of f(x) reflects the graph of f(x) across the x-axis, we can determine the y-intercept of -f(x) by taking the opposite of the y-intercept of f(x). Since the y-intercept of f(x) is not 2, this statement is also false.
3) As x approaches positive infinity, the function f(x) approaches negative infinity.
The function f(x) is a logarithmic function with a negative coefficient, which means it approaches negative infinity as x approaches positive infinity. Therefore, this statement is true.
4) As x approaches -4 from the right, the function f(x) approaches negative infinity.
As x approaches -4 from the right, the value of f(x) becomes more and more negative without bound, which means that f(x) approaches negative infinity as x approaches -4 from the right. Therefore, this statement is also true.
In summary, statements (1) and (2) are false, while statements (3) and (4) are true.
The parallel sides of a trapezium are 20 cm and 10cm and its non parallel sides is 2 m if one of the parallel side is double he oher find the measures of the parallel sides
Answers
Answer:
The parallel sides of a trapezium are 20 cm and 10cm and its non parallel sides is 2 m if one of the parallel side is double he oher find the measures of the parallel sides
Step-by-step explanation:
Base of triangle :
(20 - 10) / 2 = 10/2 = 5m
The height, h :
You have a money jar containing nickles and quarters worth $1.55. The money jar contains 11 coins. How many of each coin do you have? HELP
Answers
Answer:
6 quarters and 1 nickel
Step-by-step explanation:
6 quarters each worth 25 cents adding up to 1.50$ and then one nickel adding on .05 $ to get you too 1.55$
Answer:
6 nickels ($0.30)
5 quarters ($1.25)
Step-by-step explanation:
the biblical account of joshua's long day is a miracle of: timing intervention of natural laws visible appearance all of these
Answers
Answer:
According to search result [1], the Biblical account of Joshua's long day is a miracle of "all of these": timing, intervention of natural laws, and visible appearance.
Step-by-step explanation:
I will give you 5 stars and will also give you brainlist. Including giving you 5 star on other questions you answered from others if you help me with this science question.

Answers
Answer:
50% red nose 50% black nose
Step-by-step explanation:
help if you know it pls don't use this as a opportunity to get points cause I really need the right answers for this test or I will fail the quarter.

Answers
Answer:
1. False
2. True
3. Sorry luv I don't know this one
4. True
5. False
6. False
Hope you pass and don't fail the quarter :)
A 6-sided number cube is rolled. Which model shows a subset of outcomes that are
odd AND multiples of 3?
Answers
Model C shows a subset of outcomes that are odd AND multiples of 3.
Which model among the options represents a subset of outcomes?Model C represents a subset of outcomes that are both odd numbers and multiples of 3 among the given options.
In Model C, the outcomes depicted satisfy the condition of being odd (numbers that are not divisible by 2) and multiples of 3. This means that the numbers shown in Model C are both odd and can be divided evenly by 3.
By examining the outcomes in Model C, one can observe that they meet the criteria of being odd and divisible by 3, forming the desired subset.
Learn more about subset
brainly.com/question/24138395
#SPJ11
Please solve for the variable and then find missing angle or side lengths

Answers
Answer:
ggg
Step-by-step explanation:
a bundle of stacked and tied into blocks that are 1,2 metres high.how many bundles are used to make one block of card?
Answers
The number of bundles to be used to make one block of cardboard is 8 bundles.
How to calculate the number of bundles used to make one block of cardboard?We shall convert the measurements to a consistent unit in order to estimate the number of bundles used to make one block of cardboard.
Now, we convert the height of the bundles and the block into the same unit like centimeters.
Given:
Height of each bundle = 150 mm = 15 cm
Height of one block = 1.2 meters = 120 cm
Next, we divide the height of the block by the height of each bundle to find the number of bundles:
Number of bundles = Height of block / Height of each bundle
Number of bundles = 120 cm / 15 cm = 8 bundles
Therefore, it takes 8 bundles to make one block of cardboard.
Learn more about measurements at brainly.com/question/26160352
#SPJ1
Question completion:
Your question is incomplete, but most probably your full question was:
The 150mm bundles are stacked and tied into blocks that are 1.2 meters high. how many bundles are used to make one block of cardboard
Using a graph below , state the variables and whether each is categorical or quantitative.

Answers
Solution
Quantitative variables are any variables where the data represent amounts (e.g. height, weight, or age).
Categorical variables are any variables where the data represent groups.
Thus,
Variable 1 : Number of cases (Quantitative)
Variable 2: Date of symptom Onset (Categorical)
What is the solution to 4x+6
Answers
Answer:
Step-by-step explanation:
10x
Answer:
10x thats the answer very easy
(8.65x103)(9.3x10-23)
Answers
To multiply the given expressions (8.65 x 10^3) and (9.3 x 10^-23), we can apply the laws of exponents. In multiplication, we multiply the coefficients and add the exponents.
First, we multiply the coefficients:
8.65 * 9.3 = 80.295
Next, we add the exponents:
10^3 * 10^-23 = 10^(3 + (-23)) = 10^-20
Combining the coefficient and the exponent, we get:
80.295 x 10^-20
Thus, the final result of the multiplication is 80.295 x 10^-20.
In scientific notation, this can be written as 8.0295 x 10^-19, where the coefficient is 8.0295 and the exponent is -19.
This means that the product of (8.65 x 10^3) and (9.3 x 10^-23) is approximately 8.0295 x 10^-19. This result represents a very small value due to the negative exponent, indicating that the product is much smaller than 1.
To learn more about coefficients : brainly.com/question/1594145
#SPJ11
Please answer this question, i request
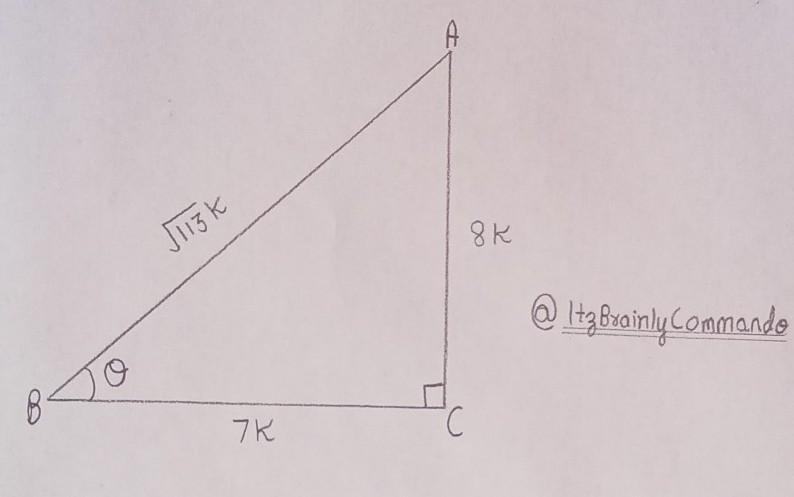
If cot θ = 7/8 , evaluate :-
(1 + sin θ)(1 – sin θ)/(1 + cos θ)(1 - cos θ)
Answers
\({\large{\textsf{\textbf{\underline{\underline{Given :}}}}}}\)
\( \star \: \tt \cot \theta = \dfrac{7}{8} \)
\( {\large{\textsf{\textbf{\underline{\underline{To \: Evaluate :}}}}}}\)
\( \star \: \tt \dfrac{(1 + \sin \theta)(1 - \sin \theta) }{(1 + \cos \theta) (1 - \cos \theta) }\)
\({\large{\textsf{\textbf{\underline{\underline{Solution :}}}}}}\)
Consider a \(\triangle\) ABC right angled at C and \(\sf \angle \: B = \theta \)
Then,
‣ Base [B] = BC
‣ Perpendicular [P] = AC
‣ Hypotenuse [H] = AB
\( \therefore \tt \cot \theta = \dfrac{Base}{ Perpendicular} = \dfrac{BC}{AC} = \dfrac{7}{8}\)
Let,
Base = 7k and Perpendicular = 8k, where k is any positive integer
In \(\triangle\) ABC, H² = B² + P² by Pythagoras theorem
\( \longrightarrow \tt {AB}^{2} = {BC}^{2} + {AC}^{2} \)
\( \longrightarrow \tt {AB}^{2} = {(7k)}^{2} + {(8k)}^{2} \)
\(\longrightarrow \tt {AB}^{2} = 49{k}^{2} + 64{k}^{2} \)
\(\longrightarrow \tt {AB}^{2} = 113{k}^{2} \)
\(\longrightarrow \tt AB = \sqrt{113 {k}^{2} } \)
\(\longrightarrow \tt AB = \red{ \sqrt{113} \: k}\)
Calculating Sin \(\sf \theta \)
\( \longrightarrow \tt \sin \theta = \dfrac{Perpendicular}{Hypotenuse}\)
\( \longrightarrow \tt \sin \theta = \dfrac{AC}{AB}\)
\(\longrightarrow \tt \sin \theta = \dfrac{8 \cancel{k}}{ \sqrt{113} \: \cancel{ k } }\)
\(\longrightarrow \tt \sin \theta = \purple{ \dfrac{8}{ \sqrt{113} } }\)
Calculating Cos \(\sf \theta \)
\( \longrightarrow \tt \cos \theta = \dfrac{Base}{Hypotenuse}\)
\( \longrightarrow \tt \cos \theta = \dfrac{BC}{ AB} \)
\( \longrightarrow \tt \cos \theta = \dfrac{7 \cancel{k}}{ \sqrt{113} \: \cancel{k } }\)
\(\longrightarrow \tt \cos \theta = \purple{ \dfrac{7}{ \sqrt{113} } }\)
Solving the given expression :-
\( \longrightarrow \: \tt \dfrac{(1 + \sin \theta)(1 - \sin \theta) }{(1 + \cos \theta) (1 - \cos \theta) } \)
Putting,
• Sin \(\sf \theta \) = \(\dfrac{8}{ \sqrt{113} }\)
• Cos \(\sf \theta \) = \(\dfrac{7}{ \sqrt{113} }\)
\( \longrightarrow \: \tt \dfrac{ \bigg(1 + \dfrac{8}{ \sqrt{133}} \bigg) \bigg(1 - \dfrac{8}{ \sqrt{133}} \bigg) }{\bigg(1 + \dfrac{7}{ \sqrt{133}} \bigg) \bigg(1 - \dfrac{7}{ \sqrt{133}} \bigg)} \)
Using (a + b ) (a - b ) = a² - b²
\(\longrightarrow \: \tt \dfrac{ { \bigg(1 \bigg)}^{2} - { \bigg( \dfrac{8}{ \sqrt{133} } \bigg)}^{2} }{ { \bigg(1 \bigg)}^{2} - { \bigg( \dfrac{7}{ \sqrt{133} } \bigg)}^{2} } \)
\(\longrightarrow \: \tt \dfrac{1 - \dfrac{64}{113} }{ 1 - \dfrac{49}{113} } \)
\(\longrightarrow \: \tt \dfrac{ \dfrac{113 - 64}{113} }{ \dfrac{113 - 49}{113} } \)
\(\longrightarrow \: \tt { \dfrac { \dfrac{49}{113} }{ \dfrac{64}{113} } }\)
\(\longrightarrow \: \tt { \dfrac{49}{113} }÷{ \dfrac{64}{113} }\)
\(\longrightarrow \: \tt \dfrac{49}{ \cancel{113}} \times \dfrac{ \cancel{113}}{64} \)
\(\longrightarrow \: \tt \dfrac{49}{64} \)
\(\qquad \: \therefore \: \tt \dfrac{(1 + \sin \theta)(1 - \sin \theta) }{(1 + \cos \theta) (1 - \cos \theta) } = \pink{\dfrac{49}{64} }\)
\(\begin{gathered} {\underline{\rule{300pt}{4pt}}} \end{gathered} \)
\( {\large{\textsf{\textbf{\underline{\underline{We \: know :}}}}}}\)
✧ Basic Formulas of Trigonometry is given by :-
\(\begin{gathered}\begin{gathered}\boxed { \begin{array}{c c} \\ \bigstar \: \sf{ In \:a \:Right \:Angled \: Triangle :} \\ \\ \sf {\star Sin \theta = \dfrac{Perpendicular}{Hypotenuse}} \\\\ \sf{ \star \cos \theta = \dfrac{ Base }{Hypotenuse}}\\\\ \sf{\star \tan \theta = \dfrac{Perpendicular}{Base}}\\\\ \sf{\star \cosec \theta = \dfrac{Hypotenuse}{Perpendicular}} \\\\ \sf{\star \sec \theta = \dfrac{Hypotenuse}{Base}}\\\\ \sf{\star \cot \theta = \dfrac{Base}{Perpendicular}} \end{array}}\\\end{gathered} \end{gathered}\)
\({\large{\textsf{\textbf{\underline{\underline{Note :}}}}}}\)
✧ Figure in attachment
\(\begin{gathered} {\underline{\rule{200pt}{1pt}}} \end{gathered} \)
Learning Task 1. Complete the following table. Write your answer in your
ne bank
the bank
is called
nofcbook
Selling Price
Rate of Discount
Discount
Sale Price
money
po 50. 00
1)
P550. 00
20%
35%
wed.
amount
3) P1,550. 00
P697. 50
ited or
Mark-up Price
Selling Price
ufter a
4)
Original Price
p950. 00
P550. 00
P1,550. 00
Mark-up Rate
20%
35%
5)
6)
P697. 50
honey
how
Total Sales
Commission
Rate of Commission
5%
7)
P 15,000. 00
8)
14%
P20,458. 00
Simple Interest
Rate
Time
9)
Principal Amount
P25,000. 00
p4,200. 00
5. 5 %
3 1/2 years
10)
P252. 00
4 years
E
werin
Answers
Answer: 057
Step-by-step explanation:
A bank loan processing system has three components with individual reliabilities as shown: R 1 = 0.82 R 2 = 0.991 R 3 = 0.98 What would be the reliability of the bank system above if each of the three components had a backup with a reliability of 0.80? How would the total reliability be different?
Answers
To calculate the reliability of the bank loan processing system with backup components, we can use the concept of series-parallel system reliability.
In the original system, the three components are connected in series. To calculate the overall reliability of the system, we multiply the reliabilities of the individual components:
R_system = R_1 * R_2 * R_3 = 0.82 * 0.991 * 0.98 ≈ 0.801
So, the reliability of the bank loan processing system without backup components is approximately 0.801.
Now, if each of the three components has a backup with a reliability of 0.80, we have a parallel configuration between the original components and their backups. In a parallel system, the overall reliability is calculated as 1 minus the product of the complement of individual reliabilities.
Let's calculate the reliability of each component with the backup:
R_1_with_backup = 1 - (1 - R_1) * (1 - 0.80) = 1 - (1 - 0.82) * (1 - 0.80) ≈ 0.984
R_2_with_backup = 1 - (1 - R_2) * (1 - 0.80) = 1 - (1 - 0.991) * (1 - 0.80) ≈ 0.9988
R_3_with_backup = 1 - (1 - R_3) * (1 - 0.80) = 1 - (1 - 0.98) * (1 - 0.80) ≈ 0.9992
Now, we calculate the overall reliability of the system with the backups:
R_system_with_backup = R_1_with_backup * R_2_with_backup * R_3_with_backup ≈ 0.984 * 0.9988 * 0.9992 ≈ 0.981
Therefore, the reliability of the bank loan processing system with backup components is approximately 0.981.
Comparing the two scenarios, we can see that introducing backup components with a reliability of 0.80 has improved the overall reliability of the system. The total reliability increased from 0.801 (without backups) to 0.981 (with backups). Having backup components in a parallel configuration provides redundancy and increases the system's ability to withstand failures, resulting in higher reliability.
Learn more about approximately here:
https://brainly.com/question/31695967
#SPJ11
what information can you gather from the standard deviation, particularly as it pertains to the percentage of normal distribution?
Answers
The standard deviation of a data set provides information about the spread or dispersion of the data around the mean.
It helps to determine the percentage of normal distribution in the data. If the standard deviation is small, it indicates that the data is tightly clustered around the mean and a high percentage of the data falls within a few standard deviations of the mean. On the other hand, if the standard deviation is large, it suggests that the data is more spread out and a smaller percentage of the data falls within a few standard deviations of the mean.
In a normal distribution, approximately 68% of the data falls within one standard deviation of the mean, 95% within two standard deviations, and 99.7% within three standard deviations. So, the standard deviation provides insight into the proportion of data points that are close to the mean, and therefore, the shape of the distribution.
Learn more about Standard Deviation here:
https://brainly.com/question/475676
#SPJ4