What is the probability of guessing all of the correct answers?
P(correct) = _______
Answers
Since each response is distinct, the odds of correctly predicting each response is 1/3× 1/3× 1/3, or 1/27.
Probability is the ability to happen. The occurrence of random events is the subject of this branch of mathematics. The value is expressed as a number between 0 and 1.
For instance, when a coin is tossed into the air, a Head or Tail are two possible results.
Hence, the term "probability" is most commonly used to refer to the likelihood that a specific event (or group of events) will occur, either as a linear scale from 0 (impossibility) to 1 (certainty) or as a percentage between 0 and 100%. Statistics is the study of events subject to probability.
To learn more about probability, visit:
brainly.com/question/11234923
#SPJ1
Related Questions
A thief entered an orange garden and stole some oranges. The guard caught him. To get rid of him, the thief gave him half of the stolen oranges and two more oranges. The thief was left with only 9 oranges. How many oranges did he stole
Answers
The thief stole 22 oranges using algebraic equations.
To solve this problem, we need to use algebra. Let x be the number of oranges the thief stole.
According to the problem, the thief gave the guard half of the stolen oranges and two more. This means that he gave away (1/2)x + 2 oranges.
We also know that the thief was left with only 9 oranges. So we can set up the equation:
x - [(1/2)x + 2] = 9
Simplifying this equation:
(1/2)x - 2 = 9
(1/2)x = 11
x = 22
Therefore, the thief stole 22 oranges.
The problem presents us with a scenario where a thief entered an orange garden and stole some oranges. However, he was caught by the guard. In order to get rid of the guard, the thief decided to give him half of the stolen oranges and two more. As a result, the thief was left with only 9 oranges.
To solve this problem, we used algebraic equations. We let x be the number of oranges the thief stole. We also knew that the thief gave away (1/2)x + 2 oranges to the guard. Using this information, we were able to set up an equation where x - [(1/2)x + 2] = 9. Simplifying the equation, we were left with (1/2)x - 2 = 9. Solving for x, we found that the thief had stolen 22 oranges.
In conclusion, algebraic equations are a useful tool in solving mathematical problems. By setting up an equation and simplifying it, we were able to determine the number of oranges that the thief had stolen.
To learn more about algebraic equations, refer here:
https://brainly.com/question/953809#
#SPJ11
Consider f(x)=x(x^3+1)+6x^2-8xDescribe the end behavior include how you got your awnser
Answers
To find the end behavior of the function:
\(f(x)=x(x^3+1)+6x^2-8x\)We look at the graph of it:
And look at what happens when the x is to small and to big.
• When x goes to infinity the function goes to infinity.
,• When x goes to minus infinity the function goes to infinity.
The other way to find this is taking the limits to infinity in each end:
\(\lim _{x\to\infty}f(x)=\infty\)\(\lim _{x\to-\infty}f(x)=-\infty\)
ILL GIVE BRAINLIST!! PLEASE HELP!!

Answers
Answer:
125
Step-by-step explanation:
125x5=625
125x8=1000
\(\frac{1}{2} x-7=\frac{1}{3} (x-12)\)
Answers
1/2x = 1/3x + 3
1/2x - 1/3x = 3
1/6x = 3
X = 18
A vegetable distributor knows that during the month of august, the weights of its tomatoes are normally distributed with a mean Of 0.62 lb and a standard deviation of 0.15.
A ) What percent of the tomatoes weight less than 0.77 lb?
B ) In a shipment of 8000 tomatoes can be expected to weight more then 0.32 lb?
C ) in a shipment of 3500 tomatoes how many tomatoes can be expected to weight from 0.32 lb to 0.92 lb
Answers
In a shipment of 3500 tomatoes can be expected to weight from 0.32 lb to 0.92 lb is 3340. When A vegetable distributor knows that during the month of august, the weights of its tomatoes are normally distributed with a mean Of 0.62 lb and a standard deviation of 0.15.
Define mean and standard deviation.A low standard deviation implies that the data are grouped around the mean, whereas a large standard deviation shows that the data are more dispersed. In contrast, a high or low standard deviation indicates that the data points are, respectively, above or below the mean. A standard deviation that is close to zero implies that the data points are close to the mean. The standard deviation measures the degree of dispersion of a set of data. Each data point is compared to the average of all the data points, and standard deviation gives a determined value that indicates whether the data points are clustered together or dispersed. The standard deviation of a normal distribution indicates how far values deviate from the mean.
Given,
A vegetable distributor knows that during the month of august, the weights of its tomatoes are normally distributed with a mean Of 0.62 lb and a standard deviation of 0.15.
Normal variable of x = 0.32
Z = X - mean/ standard deviation
Z = 0.32 - 0.62/0.15
Z = -2
Now, normal variable of x = 0.92
Z1 = X - mean/ Standard deviation
Z1 = 0.92 - 0.62/ 0.15
Z1 = 2
Required area,
P(-2 ≤ z ≤ 2)
2P(0 ≤ z ≤2 )
2 × 0.4772
0.9542
Weight of 3500 tomatoes
3500 × 0.9542
3340
To learn more about standard deviation and mean, visit:
https://brainly.com/question/23907081
#SPJ1
What's the sum of 488 in scientific notations?
Answers
The sum of 488 in scientific notations is 4.88 × 10^1.
We are given that;
The number = 488
Now,
488 in scientific notation
Step 1: Move the decimal point so that there is only one non-zero digit to the left of it.
488.0
The decimal point is moved one place to the left.
4.88
Step 2: Write the number as a product of the decimal and a power of 10.
The decimal point was moved one place to the left, so the exponent is 1.
4.88 × 10^1
Therefore, by the algebra the answer will be 4.88 × 10^1.
More about the Algebra link is given below.
brainly.com/question/953809
#SPJ1
The height of an object at any time can be found with the polynomial - 16t ^ 2 + 550 where t is the time in seconds. How high is the object after 3.5 seconds?
Answers
Answer:
354 m
Step-by-step explanation:
to find the height substitute t = 3.5 into the height polynomial
height = - 16(3.5)² + 550 = - 16(12.25) + 550 = - 196 + 550 = 354
What is the mode of the following numbers?
10, 6, 4, 4, 6, 4, 1
Answers
Answer:
4
Step-by-step explanation:
10 = one time
6 = two times
4 = three times
1 = one time
mode means most, which number is there the most of
p x 3,700=4,070
There was a % percent increase in the number of visitors.
Answers
There was 110% percent increase in the number of visitors.
Percent:
Basically, percentage refers the number or ratio that can be expressed as a fraction of 100.
Given,
p x 3,700=4,070
There was a % percent increase in the number of visitors.
Here we need to find the increase in the percentage.
While we looking into the given question we have identified that,
The Old population = 3700
And the new population = 4070
So, the value of P is calculated as,
=> p = 4070/3700
=> p = 1.1
When we convert this into percent then we get 110% as increase.
To know more about Percentage here.
https://brainly.com/question/13729841
#SPJ1
Which statement about the ordered pairs (-9, 3) and (2, -4) is true for the equation 6x=14? 2
Both ordered pairs are solutions.
(-9, 3) is a solution to the equation.
Neither ordered pair is a solution.
(2, 4) is a solution to the equation.
Answers
Answer:
neither ordered pair is a solution
Step-by-step explanation:
to determine if the pairs are a solution, substitute the x- coordinate into the left side of the equation and if equal to the right side then they are a solution.
(- 9, 3 ) with x = - 9
6(- 9) = - 54 ≠ 14 ← not a solution
(2, - 4 ) with x = 2
6(2) = 12 ≠ 14 ← not a solution
Need help to solve Solving system of equation by Substitution method ( standard form)
y= -7x-23
3x-4y= 30
This is what I have so far, but I'm stuck at the last part where I have to divide -25x with 122 which gave me a decimal number.

Answers
3x-4y= 30
3x-4(-7x-23)=30
3x+28x+92=30 -4 multiplied by -7x and -23 is a positive outcome not negative
31x+92=30
31x=30-92
31x=-62
x= -62/31
x= 2
I think this is the answer, I am a little rusty in the substitution method
Consider the function represented by 9x+3y= 12 with x as the independent variable. How can this function be written
using function notation?
flv -v+
f(x) = - 3x + 4
f(x) = - *x+
f(V) = - 3y + 4
Answers
Answer:
b) y = f(x) = -3x+4
Step-by-step explanation:
Explanation
Given that the equation 9x+3y =12
Let 'x' be an independent variable and 'y' be the dependent variable then the function is of the form
y = f(x)
3 y = 12 - 9x
\(y = \frac{-9x+12}{3}\)
y = -3x+4
The function represented by y = -3x+4
Which situation can be represented by -35 + 35? Choose all that apply.
Last night Jenny read 35 fewer pages than the night before. She reads 35 pages tonight.
A diver is 35 feet below the surface of the water and then comes up to the surface.
Eric adds 35 milliliters of water to a test tube containing 35 milliliters.
Manuel owes the electric company $35 and he pays them $35.
Answers
Answer: B & D
Step-by-step explanation:
In this scenario we want to come out with a situation where both sides are in an even playing field, as the equation solves to zero.
If a diver is 35 feet below water (-35) and he comes up to breathe, he goes up 35ft (35) meaning he is at the surface (0).
If Manuel is in debt for 35$ (-35) and then he pays off the 35$ (+35) then he is even (at 0).
Answer:
A diver is 35 feet below the surface of the water and then comes up to the surface.
what is y = |x + 4| − 8 reflected over the y axis then translated 2 units down
Answers
Answer: y = |x - 4| − 10
Step-by-step explanation: Reflection over the y-axis switched the first sign and -2 was added to -8 due to the translation 2 units down.
Hope this helps!
I Need the answer and the steps

Answers
Answer:
perimeter=16root(5) mm
area=80 mm^2
Step-by-step explanation:
perimeter of a square is =4a=4*4root(5) mm
=16root(5) mm
area of a square is=a^2=(4root(5))^2=80 mm^2
solving polynomial(-2y-6)(-3y-8)
Answers
Answer:
(-2y-6)(-3y-8)
= 6y²+16y+18y+48
= 6y²+ 34 y +48
Hope this helps
if u have question let me know in comments ^_^
Answer:
Here is your answer!!!
Step-by-step explanation:
-2y(-3y-8)-6(-3y-8)
6y^2+16y+18y+48
6y^2+34y+48
Please Help Me Guys :)

Answers
Answer:
5. x=80°, y= 80°
Step-by-step explanation:
5.x=80 (Vertically opposite angle V.O.A)
y=80 (alternate angle)
Apple Picking
Name
josiph
Linda and Robin went apple picking. After picking all day, Linda had 15 more
apples than Robin. Together, they gathered 105 apples. Figure out how many
apples Linda got and how many Robin got. Linda got 1/3 of all her apples from
the first tree and % of all her apples from the second tree. She got the rest of her
apples from the third tree. Robin got 1/3 of all her apples from the first tree and
5/9 of all her apples from the second tree. She got the rest of her apples from
the third tree. Who got more apples from the third tree? How many more?
Answers
We can write the system of equations:
x = y + 15
x + y = 105
Solving the system, we will see that Robin has 45 apples and Linda 60 apples.
How many apples do Robin and Linda got?Here we can only solve the first part, as the second is incomplete.
Let's define the variables:
x = apples that Linda has.
y = apples that Robin has.
We know that Linda has 15 more apples than Robin, and that between the two there are 105 apples, so we can write the system of equations:
x = y + 15
x + y = 105
To solve this, we can replace the first equation into the second one, so we get:
(y + 15) + y = 105
2y + 15 = 105
2y = 105 - 15 = 90
y = 90/2 = 45
So Robin has 45 apples, and Linda has 15 more, so she has:
x = 45 + 15 = 60
Learn more about systems of equations:
https://brainly.com/question/13729904
#SPJ1
what is .6239 as fraction?
Answers
Answer:
.6239 as a fraction is 6239/10000.
All you have to do is put the digits after the decimal over a number that is one place value larger
Can someone help?
Identify the coefficients and degree of each item
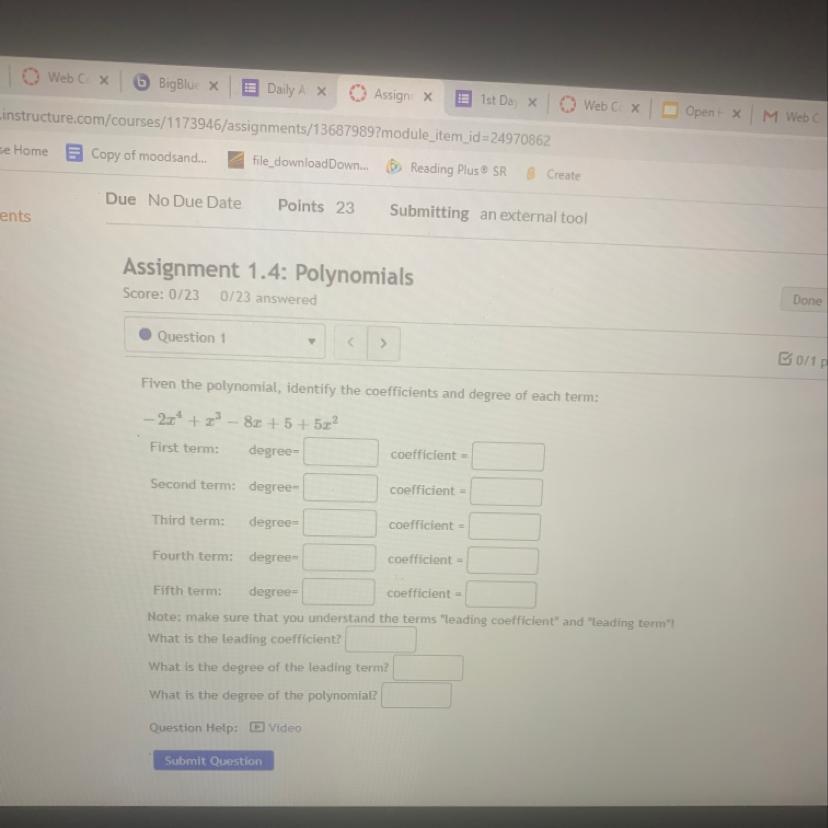
Answers
Answer:
first term: degree=4, coefficient= -2
second term: degree=3, coefficient=1
third term: degree=1, coefficient= -8
fourth term: degree= 1, no coefficient
fifth term: degree=2, coefficient=5
Step-by-step explanation:
Answer:
Hey there!
First term, degree 4, coefficient -2.
Second term, degree 3, coefficient 1
Third term, degree 1, coefficient -8
Fourth term, degree 1, coefficient none
Fifth term, degree 2, coefficient 5
Hope this helps :)
Ben's earnings for work he did from Monday through Saturday were: $78, $94, $115, $108, $67, $78. What was his average daily pay for the days he worked?
Answers
Answer:
His average daily pay was $90.
Step-by-step explanation:
78+94+115+108+67+78=540
540/6= 90.
El triángulo ABC es equilátero y L, M y N son los puntos medios de BC, AB y CA respectivamente. Si MN = 3, ¿cuál es el valor de ML?

Answers
The value of ML = 3, using the mid-point theorem of triangles.
According to the midpoint theorem, "the line segment of a triangle crossing the midpoints of two sides of the triangle is said to be parallel to its third side and also half the length of the third side."
In the question, we are given that triangle ABC is an equilateral triangle, and L, M, and N are the midpoints of BC, AB, and CA respectively.
Thus, by the midpoint theorem, we can say that:
MN || BC, and MN = (1/2)BC,ML || AC, and ML = (1/2)AC, andNL || AB, and NL = (1/2)AB.Assuming AB = BC = AC = x units, we get:
MN = (1/2)BC = x/2,ML = (1/2)AC = x/2, andNL = (1/2)AB = x/2.
Thus, the triangle LMN is an equilateral triangle.
Thus, MN = ML = NL.
Given MN = 3, we can write the value of ML = 3.
Thus, the value of ML = 3, using the mid-point theorem of triangles.
Learn more about the mid-point theorem of triangle at
https://brainly.com/question/9635025
#SPJ1
The given question is in Spanish. The question in English is:
"Triangle ABC is equilateral and L, M, and N are the midpoints of BC, AB, and CA respectively. If MN = 3, what is the value of ML?"
Consider the following functions. (*) cos(24), 1₂(X) - 1₁ g(x) + CO²(x) Solve for C₁, C₂, and cy so that ola)-0 on the interval (, ). If a nontrivial solution exsts, state it. If only the tri
Answers
The determinant of the matrix is (g(b) - g(a))^2 - 4δ(g(b) - g(a))(δ(b - a)).If (g(b) - g(a))^2 - 4δ(g(b) - g(a))(δ(b - a)) = 0, then the non-trivial solution exists. Otherwise, only the trivial solution exists.
The given functions are cos(24), 1₂(x) - 1₁g(x) + CO²(x). We are to solve for C₁, C₂, and C₃ so that b) 0 on the interval (a, b). If a nontrivial solution exists, state it. If only the trivial solution exists, state that as well.Step-by-step solution:
Given functions: cos(24), 1₂(x) - 1₁g(x) + CO²(x)Let A(x) = cos(24)B(x) = 1₂(x) - 1₁g(x) + CO²(x)Consider the following conditions:
At x = a, A(a) = cos(24)At x = b, A(b) = cos(24)B(a) = 1₂(a) - 1₁g(a) + CO²(a)B(b) = 1₂(b) - 1₁g(b) + CO²(b)Condition: b) 0 on the interval (a, b)A(a) = cos(24)A(b) = cos(24)B(a) = 1₂(a) - 1₁g(a) + CO²(a)B(b) = 1₂(b) - 1₁g(b) + CO²(b)On applying integration on the given functions, we get:∫A(x)dx = cos(24)(x - x) = 0 At x = a, ∫B(x)dx = C₁(x - a) + C₂(x - a)g(a) + C₃(x - a)Owing to the conditions, we have∫B(x)dx = 0 On substituting the values of a and b, we have C₁(b - a) + C₂(g(b) - g(a)) + C₃(δ(b - a) = 0 If the determinant of the matrix is zero, then there exist non-trivial solutions for C₁, C₂, and C₃.δ is the Kronecker delta function given as:δ(t) = 1 if t = 0 and δ(t) = 0 if t ≠ 0
To know more about non-trivial solution,visit:
https://brainly.com/question/30930809
#SPJ11
Write each vector as a linear combination of the vectors in S. (If not possible, enter IMPOSSIBLE.) S = {(2,-1, 3), (5,0,4)) (a) z = (11,-8, 20) z= S2 v=(23,--, 75 4' 4 (b) v= S1 + S2 (c) w= (1,-8,12) w= S1 S2 u=
Answers
Vector z can be expressed as a linear combination of vectors in S, while vectors v and w cannot be expressed in terms of S.
(a) To express vector z = (11, -8, 20) as a linear combination of the vectors in S = {(2, -1, 3), (5, 0, 4)}, we need to find scalars x and y such that z = x(2, -1, 3) + y(5, 0, 4). By solving the system of equations, we find that x = 3 and y = 1. Therefore, z = 3(2, -1, 3) + (5, 0, 4) = (11, -8, 20), so it is possible.
(b) To express vector v = (23, ?, 75, 4) as a linear combination of the vectors in S = {(2, -1, 3), (5, 0, 4)}, we add the two vectors in S together: (2, -1, 3) + (5, 0, 4) = (7, -1, 7). However, since the given vector v has a missing component denoted by "?", we cannot determine the linear combination of v using the vectors in S. Therefore, it is not possible to express v as a linear combination of the vectors in S.
(c) To express vector w = (1, -8, 12) as a linear combination of the vectors in S = {(2, -1, 3), (5, 0, 4)}, we need to find scalars x and y such that w = x(2, -1, 3) + y(5, 0, 4). However, upon solving the system of equations, we find that there are no values of x and y that satisfy the equation. Therefore, it is impossible to express w as a linear combination of the vectors in S.
Learn more about Vector click here :brainly.com/question/13322477
#SPJ11
2/3(9x-6)=1/4(12-4x)
Answers
Answer:
x = 1
Step-by-step explanation:
\(\frac{2}{3}\) (9x - 6) = \(\frac{1}{4}\) (12 - 4x) ← distribute parenthesis on both sides
6x - 4 = 3 - x ( add x to both sides )
7x - 4 = 3 ( add 4 to both sides )
7x = 7 ( divide both sides by 7 )
x = 1
write a quadratic function in the given form whose graph satisfies the given condition.
passes through (-4, 0), (2, 0), & (6, -20) in intercept form.
pls dont just steal points away...i rly need help with this...can you provide work to if not thats ok
Answers
Answer:
\(\displaystyle y=-\frac{1}{2}(x+4)(x-2)\)
Step-by-step explanation:
We want to find a quadratic that passes through the points:
\((-4, 0), \, (2, 0), \text{ and } (6, -20)\)
In intercept form.
First, note that the first two given points are the x-intercepts of our quadratic. Intercept or factored form is given by:
\(y=a(x-p)(x-q)\)
Where p and q are the x-intercepts, and a is the leading coefficient.
So, we will substitute -4 and 2 for p and q:
\(y=a(x-(-4))(x-(2))\)
Simplify:
\(y=a(x+4)(x-2)\)
Next, the third point (6, -20) tells us that y = -20 when x = 6. So:
\((-20)=a(6+4)(6-2)\)
Solve for a:
\(-20=10(4)a\Rightarrow 40a=-20\)
Thus:
\(\displaystyle a=\frac{-20}{40}=-\frac{1}{2}\)
Hence, our equation in intercept from is:
\(\displaystyle y=-\frac{1}{2}(x+4)(x-2)\)
3x2 +4x-5 = 5x2 + 2x +1
Answers
The values of x which are solutions to the given quadratic equation as required to be determined are; x = (1 ± i√11) / 2.
What is the solution for x in the given quadratic equation?It follows from the task content that the given quadratic equation is to be solved for variable, x.
3x² + 4x - 5 = 5x² + 2x + 1;
By collect like terms and evaluating; we have that;
2x² - 2x + 6 = 0
By solving the equation by means of the formula method; we find that;
x = (1 ± i√11) / 2
Ultimately, the values of x which holds True are; x = (1 ± i√11) / 2.
Read more on quadratic equations;
https://brainly.com/question/30487356
#SPJ1
who wants to help me??

Answers
Answer:
Function 1 is negative with a slope of -5 and Function 2 is positive with a slope of 3.
Step-by-step explanation:
Slope Formula: (y2 - y1)/(x2 - x1)
Function 1: m = -2 - (-12) / 1 - 3
m = 10 / -2
m = 5 / -1
m = -5
Function 2: m = 3/1
m = 3
Megan boarded a train at 10:23 and arrived at her destination
3 hours 5 minutes later. What time did she arrive at her.
destination
Answers
She arrived at 1:28
A pharmacist mixed 800 mg of a liquid drug (sg = 0.8) in enough liquid to prepare 2 liters of a final product. what is the ratio strength of the final product?
Answers
The ratio strength of the final product is 1:0.4.
What is ratio strength?One technique to indicate concentration is through ratio strength, which uses a ratio to characterize drug concentration. In this context, concentration is defined as the quantity of a solute that makes up one unit in the whole volume of a solution or combination. You should be an expert in ratio strength calculations as a pharmacy student. Ratio strength uses a ratio to describe the medication concentration. So, what it implies is, you have one unit of solute contained in the complete amount of preparation and so generally your ratio strength is expressed as one is to something. In the case of a weight-in-weight, volume-in-volume, or weight-in-volume situation, you would interpret a ratio of 1:2,000 differently.
Drug strength = 800mg
whole volume =2ltrs
The ratio of the solution in 1 ltr is = 400 mg
The ratio strength of the solutions is = 1:0.4
To know more about ratio strength, visit;
https://brainly.com/question/12123928
#SPJ4