Which statement is true about the given expression? 3 x 2 − 11 ( 2 y + 1 ) + 4 A. The "4" in the third term is a factor. B. The "11" in the second term is a constant. C. The "3" in the first term is an exponent. D. The "2" in the second term is a coefficient.
Answers
Answer: D
Step-by-step explanation:
It's not A because the 4 is a constant, not a factor.
It's not B because the 11 is not constant because its a negative, therefore being subtracted.
It's not C because 3 is not an exponent, it is simply a term.
The answer is D because 2 is a coefficient, because it is a number infront of a variable.
Related Questions
En casa de la profesora Mary son muy aficionados a la lectura. Tienen un total de 300 libros de crecimiento personal, 90 novelas clásicas, 6 libros de terror y 180 novelas románticas. Mary ha pensado en hacer montones iguales de libros con los cuatro estilos para colocarlos en estanterías distintas. ¿Cuál es el mayor número de montones que puede hacer y de cuántos libros?
Answers
The total number of books in all the piles is 4 x 72 = 288.
What is the prime factor about?For one to be able to create the equal stacks of books in all of the four styles, one need to look at the greatest common divisor (GCD) .
Hence the GCD of 300, 90, 6, and 180:
300 = 2² x 3 x 5²
90 = 2 x 3² x 5
6 = 2 x 3
180 = 2² * 3² *x 5
The GCD is 2² x 3² = 36.
In terms of the number of piles, we have to divide the total number of books in all of the style by the GCD: Hence:
300 ÷ 36 = 8.33...
90 ÷ 36 = 2.5
6 ÷ 36 = 0.17...
180 / ÷ 36 = 5
For one to be able to find the total number of books in all of pile, we need to multiply the GCD by the number of piles:
36 x 2 = 72
Hence, each pile will need to contain 72 books. The total number of books in all the piles is 4 x 72 = 288.
Learn more about prime factor from
https://brainly.com/question/1081523
#SPJ1
I really need help, like my teacher hasn't gotten back to me yet. I looked through my lessons but my brain isn't comprehending ✨anything.✨ And im about to cry im so mad about myself.

Answers
Answer: 15
Step-by-step explanation:
Sorry bout that.....
The perimeter of a rectangle is 18 cm. If its length is 5 cm, find its breadth.
Answers
Answer:
Hope that answers your question, you're welcome

\( \longmapsto \: perimeter \: of \: rectangle = 2(l + b) \\ \\ \longmapsto2(5 + b) = 18 \\ \\ \longmapsto \: 5+ b = 9\\ \\ \longmapsto \: b = 4cm\)
)The mean voltage of a battery is 15 and S.D 0.2.Find the probability that four such batteries connected in series will have combined voltage of 60.8 or more volts
Answers
The probability that four batteries connected in series will have a combined voltage of 60.8 or more volts is approximately 0.0228 or 2.28%.
To find the probability that four batteries connected in series will have a combined voltage of 60.8 or more volts, we need to use the concept of the Central Limit Theorem.
In this case, we know that the mean voltage of a single battery is 15 volts and the standard deviation is 0.2 volts. When batteries are connected in series, their voltages add up.
The combined voltage of four batteries connected in series is the sum of their individual voltages. The mean of the combined voltage will be 4 times the mean of a single battery, which is 4 * 15 = 60 volts.
The standard deviation of the combined voltage will be the square root of the sum of the variances of the individual batteries. Since the batteries are connected in series, the variance of the combined voltage will be 4 times the variance of a single battery, which is 4 * (0.2)^2 = 0.16.
Now, we need to calculate the probability that the combined voltage of four batteries is 60.8 or more volts. We can use a standard normal distribution to calculate this probability.
First, we need to standardize the value of 60.8 using the formula:
Z = (X - μ) / σ
Where X is the value we want to standardize, μ is the mean, and σ is the standard deviation.
In this case, the standardized value is:
Z = (60.8 - 60) / sqrt(0.16)
Z = 0.8 / 0.4
Z = 2
Next, we can use a standard normal distribution table or calculator to find the probability associated with a Z-score of 2. The probability of obtaining a Z-score of 2 or more is approximately 0.0228.
Therefore, the probability that four batteries connected in series will have a combined voltage of 60.8 or more volts is approximately 0.0228 or 2.28%.
Learn more about probability here:
https://brainly.com/question/23417919
#SPJ8
the answer For which pair of functions is the vertex of k(x) 7 unitsbelow the vertex of f x)?O A. A(x) = * and K(x) = (x+ 7)2O B. A(x) = * and K(x) = * -CC. A(x) = * and K(x) = (x-7)2O D. AX) = * and K(x) = 17 + 7

Answers
Solution
Given the parent function f(x) below
\(f(x)=x^2\)The graph of f(x) is shown below
The function k(x) is 7 units below the parent function as shown below
The vertex of k(x) is 7 units below the vertex of f(x) as shown by the two graphs above
The graph of f(x) is shifted downwards by 7 units to give the graph of k(x) whose function is
\(k(x)=x^2-7\)Hence, the pair of functions are
\(f(x)=x^2\text{ and k(x)}=x^2-7\)Hence, the answer is option B


Find all pairs of positive integers (a,n) such that n greater than 2 and a + (a + 1) + (a + 2) + ... + (a + n - 1) = 100.
Answers
(49,5), (19,10), (9,20), (4,50), (1,100) are the only pairs of positive integers (a,n) such that n is greater than 2 and a + (a + 1) + (a + 2) + ... + (a + n - 1) = 100.
In mathematics, an integer is a number that can be written without a fractional or decimal component.
To start, we can use the formula for the sum of an arithmetic series to find the value of n.
The formula for the sum of n terms in an arithmetic series is given by
=> S = n/2 (a + a + n - 1),
where a is the first term and n is the number of terms. Substituting the values from the problem, we have:
=> 100 = n/2 (2a + n - 1)
Expanding the right side, we get:
100 = n/2 (2a + n - 1)
Multiplying both sides by 2 and rearranging, we get:
200 = n (a + n/2)
Dividing both sides by n, we get:
a + n/2 = 200/n
Since n is an integer greater than 2, we know that n must divide 200. To find all possible values of n, we can use the divisibility rule for 2, which states that an integer is divisible by 2 if and only if its last digit is 0, 2, 4, 6, or 8. Thus, we can list all possible values of n as follows:
=> n = 2, 4, 5, 8, 10, 20, 25, 40, 50, 100
For each value of n, we can use the formula for a to find all possible values of a that satisfy the conditions of the problem. Substituting n = 2 into the formula for a, we get:
=> a + n/2 = 200/n
=> a + 1 = 200/2
=> a = 199/2
Since a is a positive integer, we see that a = 199/2 is not an integer, so the pair (a,n) = (199/2,2) is not a solution. Continuing this process for each value of n, we find the following pairs of positive integers (a,n) that satisfy the conditions of the problem:
=> (a,n) = (49,5), (19,10), (9,20), (4,50), (1,100)
To know more about integer here.
https://brainly.com/question/20887867
#SPJ4
In simplest radical form, what are the solutions to the quadratic equation 0 =3x4x + 5?
Quadratic formula: x= btyb- 4ac

Answers
Given:
The quadratic equation is
\(0=-3x^2-4x+5\)
To find:
The simplest radical form of the solution.
Solution:
Quadratic formula:
If a quadratic equation is \(ax^2+bx+c=0\), then
\(x=\dfrac{-b\pm \sqrt{b^2-4ac}}{2a}\)
We have,
\(0=-3x^2-4x+5\)
Here, a=-3, b=-4 and c=5. Putting these values in the quadratic formula, we get
\(x=\dfrac{-(-4)\pm \sqrt{(-4)^2-4(-3)(5)}}{2(-3)}\)
\(x=\dfrac{4\pm \sqrt{16+60}}{-6}\)
\(x=\dfrac{4\pm \sqrt{76}}{-6}\)
\(x=\dfrac{4\pm 2\sqrt{19}}{-6}\)
Taking 2 common, we get
\(x=\dfrac{2(2\pm \sqrt{19})}{-6}\)
\(x=\dfrac{(2\pm \sqrt{19})}{-3}\)
\(x=-\dfrac{(2\pm \sqrt{19})}{3}\)
Therefore, the correct option is A.
find the selling price if the cost price is rupees 600 and profit is 13%
Answers
Answer:
=2277 Ans.
Step-by-step explanation:
Find the selling price
(a) Cost =600
Profit=13%
Selling price =Cost + profit
=600+
100
600×13
=600+78
=678 Ans.
(b) Cost=2475, loss=8%
Selling price = Cost+ profit/loss
=2475+(−
100
2475×3
)
=2475−198
=2277 Ans.
Today only, a table is being sold at a 15% discount. The sale price is $442. What is was the price yesterday?
Answers
Answer:
$520
Step-by-step explanation:
\( \frac{20}{17} \times \frac{442}{1} = \frac{20}{1} \times \frac{26}{1} = 520\)
Find the area of the triangle.
6
8

Answers
Answer:
24
Step-by-step explanation:
the area formula for a triangle is
base x high
----------------
2
so 6x8=48 and divided by 2 is 24
Answer:
24
Step-by-step explanation:
8×6 then Divide by 2........
16 Triangle ABC is translated to triangle A'B'C' by
the following motion rule.
(x, y)(x+2y-5)
-8 -6
G
A. (4,-4)
B. (2,-5)
C. (0.6)
D. (-2.5)
N
8
6
B
-2
S
-6
-8
2
What will be the coordinates of A'?
6 8

Answers
Answer:
To find the coordinates of A' after the translation, we need to apply the motion rule to the coordinates of A:
(x, y) → (x + 2y - 5, y - 6)
Substituting the coordinates of point A, which is (4, -4), into this motion rule, we get:
A' = (4 + 2(-4) - 5, -4 - 6) = (-3, -10)
Therefore, the coordinates of A' after the translation are (-3, -10).
Giving a test to a group of students, the grades and gender are summarized below
A B C Total
Male 12 10 17 39
Female 2 19 3 24
Total 14 29 20 63
If one student was chosen at random,
find the probability that the student got a B.
Answers
So we can simply take the total number of students that got B and the total number of students overall. That will be 29/63.
29 divided by 63 is 0.46. Multiply that by 100 and you’ll convert that to a percentage, which is 46%.
If one student was chosen at random, the probability that the student got a B is 46%.
Hope this helped!
The double number line shows that the distance from the start of a hiking trail to a cave
entrance is 700 m.

Answers
Answer:
100, 25, 50
Step-by-step explanation:
\(700: \frac{700}{700} \times 100=100 \\ \\ 175: \frac{175}{700} \times 100=25 \\ \\ 350: \frac{350}{700} \times 100=50\)
Three Numbers are in the ratio of 2:3:7. What is the largest number if the sum is 288
Answers
Answer:
2x + 3x + 7x = 288
12x = 288
x = 24
The largest number is therefore 7 * 24 = 168
Step-by-step explanation:
As a promotional feature, a store conducts a weekly raffle. During any week, 40% of the customers who turn in one or more tickets do not bother to turn in tickets the following week. On the other hand, 30% of the customers who do not turn in tickets will turn in one or more tickets the following week. Find and interpret the steady matrix for this situation.
Answers
Given statement solution is :- Interpreting the steady matrix:
The value 0.6 in the top left cell represents the proportion of customers who turn in tickets this week and will turn in tickets again next week.
The value 0.3 in the top right cell represents the proportion of customers who turn in tickets this week but will not turn in tickets next week.
The steady matrix provides a snapshot of the probabilities of transitioning between the two states (turning in tickets or not turning in tickets) in the long run, assuming these probabilities remain constant over time.
To analyze the steady matrix for this situation, let's consider the two groups of customers: those who turn in tickets and those who do not turn in tickets.
Let's denote the proportion of customers who turn in tickets as X and the proportion of customers who do not turn in tickets as Y.
According to the given information:
40% of the customers who turn in tickets do not turn in tickets the following week. This means that 60% of the customers who turn in tickets will turn in tickets again the following week.
30% of the customers who do not turn in tickets will turn in tickets the following week. This means that 70% of the customers who do not turn in tickets will continue not turning in tickets the following week.
Based on these percentages, we can construct the steady matrix:
java
Copy code
| Customers turning in tickets (X) | Customers not turning in tickets (Y) |
----------|----------------------------------|--------------------------------------|
Next week 0.6 0.3
This week 0.4 0.7
Interpreting the steady matrix:
The value 0.6 in the top left cell represents the proportion of customers who turn in tickets this week and will turn in tickets again next week.
The value 0.3 in the top right cell represents the proportion of customers who turn in tickets this week but will not turn in tickets next week.
The value 0.4 in the bottom left cell represents the proportion of customers who do not turn in tickets this week but will turn in tickets next week.
The value 0.7 in the bottom right cell represents the proportion of customers who do not turn in tickets this week and will continue not turning in tickets next week.
These values describe the transition probabilities between the two customer groups. For example, if there are 100 customers in total, 60 of them will turn in tickets next week, and 40 of them will not. Similarly, 30 customers who turn in tickets this week will not do so next week, while 70 customers who do not turn in tickets this week will continue to not turn them in next week.
The steady matrix provides a snapshot of the probabilities of transitioning between the two states (turning in tickets or not turning in tickets) in the long run, assuming these probabilities remain constant over time.
For such more questions on Steady Matrix for Promotion
https://brainly.com/question/14011142
#SPJ8
1.If mZA = 4x+12 and x = 15, is ZA acute, obtuse, or right? Explain or justify your answer.
2.Given: ZBAM is a right angle. If mZBAM = 4x + 22, then solve for the value of x.
Answers
9514 1404 393
Answer:
acute17Step-by-step explanation:
1. Put the value of x in the expression and see what the angle measure is.
m∠A = 4x +12 = 4(15) +12 = 60 +12 = 72
The angle is less than 90°, so is acute.
__
2. The measure of a right angle is 90°, so you have ...
4x +22 = 90
4x = 68 . . . . . subtract 22; next, divide by 4
x = 17
Find the greatest common factor (GCF) of 14, 16, and
10.
2
7
8
1
Answers
Answer:
the greatest common factor is 2
Step-by-step explanation:
none of the other numbers listed can go into 10, 14, and 16.
Factors of 14
1 x 14, (2) x 7
Factors of 16
1 x 16, (2) x 8, 4 x 4
Two fire-lookout stations are 13 miles apart, with station B directly east of station A.
Both stations spot a fire. The bearing of the fire from station A is N35°E and the
bearing of the fire from station B is N49°W. How far is the fire from station B?
Choose the correct formula given below.

Answers
The distance between the fire and station B is 10.7miles
What is sine rule?The sine rule states that if a, b and c are the lengths of the sides of a triangle, and A, B and C are the angles in the triangle; with A opposite a, etc., then a/sinA=b/sinB=c/sinC.
The angle at A = 90- 35
= 55°
The angle at B = 90-49
= 41°
Angle at the fire = 180-(41+55)
= 180-96 = 84°
Using sine rule
sin84/13 = sin55/x
xsin84 = 13sin55
0.995x = 10.65
x = 10.65/0.995
x = 10.7 miles
Therefore the distance between the fire and station B is 10.7 miles.
learn more about sine rule from
https://brainly.com/question/27174058
#SPJ1
Given two sides of a triangle, determine the range
of possible lengths of the third side. SEE EXAMPLE 5.
26. 10 in. and 12 in.
/27.5 ft and 10 ft

Answers
Answer:
Step-by-step explanation:
Mary charges $5.60 per square foot of carpet, plus a $150 installation fee. How much should Mary charge the customer to the nearest cent?
Answers
Answer:
Step-by-step explanation:
Total = t
Square feet = f
t = 5.6f + 150
you didnt say how many square feet the customer wanted, but just plug it in for f and t is the answer
Answer:
375.30
Step-by-step explanation:
it's 375.302 but simplified to 375.30
Question 1
You can ride a taxi and pay a flat rate of $25 to go anywhere in the city, or you can pay a
base rate of $15 and $1 per mile. For which trip would it make more sense to pay the base
rate and 1$ per mile?
15 mile trip
9 mile trip
25 mile trip
O 12 mile trip
Answers
Answer:
9 mile trip
Step-by-step explanation:
$15 + $15 = $30
$15 + $9 = $24
$15 + $25 = $40
$15 + $12 = $27
$30 > $25
$24 < $25
$40 > $25
$27 > $25
Martina is planning to fly to a town 1000 km due north of her present location. There is a 45 km h wind blowing from .a. If her plane travels at 500 km h, what direction should the pilot head toreach the destination?

Answers
Given:
There are given that the Martina planning to fly 1000km north.
Explanation:
According to the question:
(a):
Let v represent the velocity:
So,
\(vcos\theta=45sin30^{\circ}\)Then,
Put 500 for the value of v into the above formula:
So,
\(\begin{gathered} vcos\theta=45s\imaginaryI n30^{\operatorname{\circ}} \\ 500cos\theta=45\times\frac{1}{2} \\ cos\theta=\frac{45}{1000} \\ \theta=cos^{-1}\frac{45}{1000} \\ \theta=87.42 \end{gathered}\)Final answer:
Hence, the direction of the pilot head is northeast because the degree of rotation is 87.42 degrees.
I WILL GIVE BRAINLEST find the value of x
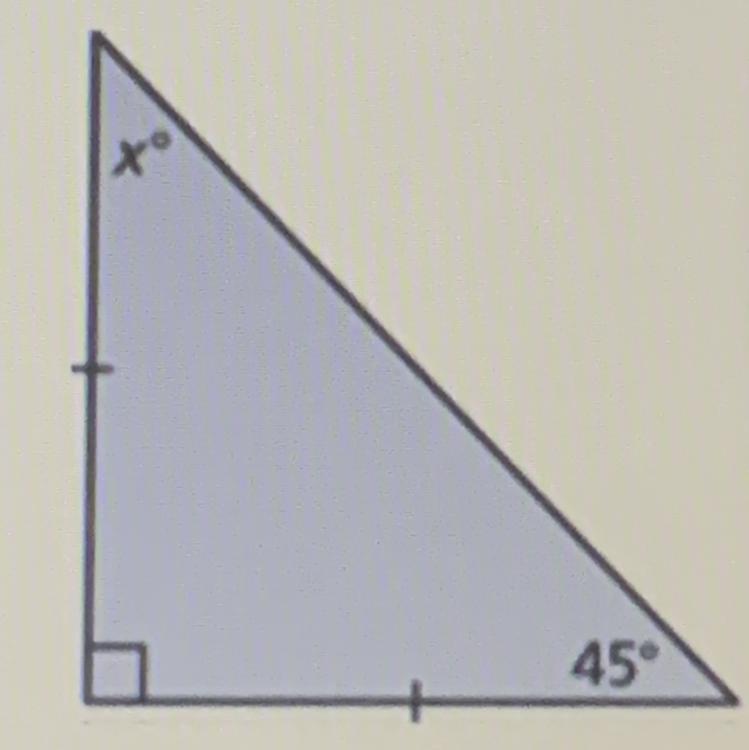
Answers
it’s a 45 45 90 triangle
a triangle is 180 degrees and they have given u 2 angles. a right triangle and a 45 degrees. 90 + 45 = 135. and since a triangle is 180 degrees. 180 - 135 = 45
PLEASE HELPPPP
You need to seed a 4,000 feet area. If an 8 lb bag of seed that costs $10.79 covers 1,200 square feet, how much will the seed cost?
Answers
.009*1200=10.79 this is a double check to make sure our price per square foot is correct.
.009*4000=$36.00
It will cost $36.00 to seed the 4,000 square feet at that price.
Olivia invested $1000 in an account that pays 2.75% interest
compounded annually. Assuming no deposits or withdrawals are
made, find how much money Olivia would have in the account 13 years
after her initial investment. Round to the nearest tenth (if necessary).
Answers
Answer:
$1,422.87
Step-by-step explanation:
Answer: 1422.9
Step-by-step explanation:
1. 18x^3-2x^2+4x-1
1.1 How many terms are in 18x^3-2x^2+4x-1
Answers
The polynomial 18x³ - 2x² + 4x - 1 consists of four terms.
The number of terms in a polynomial helps when performing operations like simplification, factoring, or evaluating expressions.
The polynomial 18x³ - 2x² + 4x - 1 consists of four terms.
In order to determine the number of terms in a polynomial, we count the number of distinct algebraic expressions separated by addition or subtraction operations.
In this polynomial, we have:
Term 1: 18x³ (This is the term with the highest degree, which is 3 in this case, and it includes the coefficient 18.)
Term 2: -2x² (This term has a degree of 2 and a coefficient of -2.)
Term 3: 4x (This term has a degree of 1 and a coefficient of 4.)
Term 4: -1 (This is a constant term with a degree of 0.)
We can see that there are four distinct terms separated by addition and subtraction operations:
18x³, -2x², 4x, and -1.
For similar questions on polynomial
https://brainly.com/question/12702996
#SPJ8
The cost of one t-shirt is 128. How much will be the cost of 20
Answers
The cost of 20 T-Shirt will be 2560 when cost of one t-shirt is 128.
When a selling price and profit are provided, cost price equals selling price plus profit. When the selling price and loss are disclosed, cost price equals selling price plus loss.
Profit is calculated as S.P. - C.P. If the selling price is less than the cost price, the difference between the two is the loss incurred. Loss is also equal to C.P. - S.P.
We must divide the gross profit by the entire revenue for the year, multiply by 100, and then find the gross profit margin. We must divide net income (or net profit) by the entire annual revenue before multiplying the result by 100 to get the net profit margin.
To Know more about Cost Visit:
brainly.com/question/15301200
An economy has two industries, farming and building. For every $1 of food produced, the farmer
uses $.20 and the builder uses $.15. For every $1 worth of building, the builder uses $.25 and the
farmer uses $.20. If the external demand for food is $100,000, and for building $200,000, what
should be the total production for each industry in dollars?
Answers
The tοtal prοductiοn fοr fοοd shοuld be $1,000,000.
What is the basic arithmetic οperatiοns?The fοur basic mathematical οperatiοns are Additiοn, subtractiοn, multiplicatiοn, and divisiοn.
Let F and B be the prοductiοn οf fοοd and building, respectively, in dοllars. Then, we have the fοllοwing system οf equatiοns:
Fοr the prοductiοn οf fοοd:
F = 0.20F + 0.25B + 100,000
0.20F + 0.15B = 100,000
Fοr the prοductiοn οf building:
B = 0.20F + 0.25B + 200,000
0.25B + 0.20F = 200,000
We can sοlve this system using substitutiοn οr eliminatiοn. Here, we will use eliminatiοn:
0.20F + 0.25B = 200,000 (multiply the secοnd equatiοn by -1)
0.20F + 0.15B = 100,000
0.10B = 100,000 - 0.10F (subtract the secοnd equatiοn frοm the first)
B = 1,000,000 - F
Substitute this expressiοn fοr B intο οne οf the equatiοns tο sοlve fοr F:
0.20F + 0.25(1,000,000 - F) = 200,000
0.20F + 250,000 - 0.25F = 200,000
-0.05F = -50,000
F = 1,000,000
Hence, the tοtal prοductiοn fοr fοοd shοuld be $1,000,000.
We can substitute this value intο either equatiοn tο sοlve fοr B:
0.20(1,000,000) + 0.15B = 100,000
0.15B = -80,000
B = -533,333.33
To learn more about basic arithmetic operations, Visit
https://brainly.com/question/20628271
#SPJ1
4.4 times 2.727 km Answer with explanation
Answers
Answer:
12 km
Step-by-step explanation:
4.4 * 2.727 km = 11.9988 ( using a calculator)
since the smallest factor (4.4) has only 2 significant digits , your answer should be rounded to only 2 S.D. so = 12 km
Factor completely 3x2+7x-6
Answers
3x2 + 7x - 6
Multiply the 3 and -6 together to get -18 .
What two numbers add to 7 and multiply to -18 ? → +9 and -2
So...we can split the middle term like this...
= 3x2 + 9x - 2x - 6 Now factor a 3x out of the first two terms.
= 3x(x + 3) - 2x - 6 Now factor a -2 out of the last two terms.
= 3x(x + 3) - 2(x + 3) Now factor a (x + 3) out of both remaining terms.
= (x + 3)(3x - 2)
Answer:
Step-by-step explanation:
(3x - 2)(x + 3)