Write an expression below that includes: one variable, two operations and three terms?

Emma is asking an empirical question so that she she can test her hypothesis

What is a Empirical hypothesis?

Something empirical is something based on real evidence .

Evidence that is verifiable by observation as opposed to something that is true in theory or by some kind of reckoning or logic

A hypothesis is a theory that is put forward for further examination to see if it is correct. It is not a call but it is an explanation that fits the available evidence so , An empirical hypothesis is a theory that is based on real evidence and experience but that has not been proven yet

So, According to the question

Emma wants to know if long distance relationship in college have a greater likelihood of ending than a relationship that are not long distance and for that she creates a questionnaire and the crowds 100 student from our school to complete the questionnaire so she can test her hypothesis

Here, Emma is asking an empirical questions ( question about experience) and will conclude the test based on these 100 students experience and thoughts.

To know more about Hypothesis here

https://brainly.com/question/29576929

#SPJ4

Answer:

The zeros of this function are -2 and 4.

No, only one zero makes sense in terms of the problem. Because there is no negative distance from the platform.

Step-by-step explanation:

Zeros are solutions, are x-intercepts. see image. When the diver is 0 feet (horizontally) from the platform, they are 8 feet up above the surface of the water. Once they jump, they are moving in a curved path that is half of a parabola. The zeros are where the diver is 0 feet above the water. We can calculate an answer of -2 but the diver is not going backwards (yikes! the pool side deck surface, ouch!) The diver will be 4 feet out (horizontally) from the platform when they hit the water. see image.

Solving:

set the function equal to 0.

Factor.

Set each factor equal to zero.

Solve. see image.

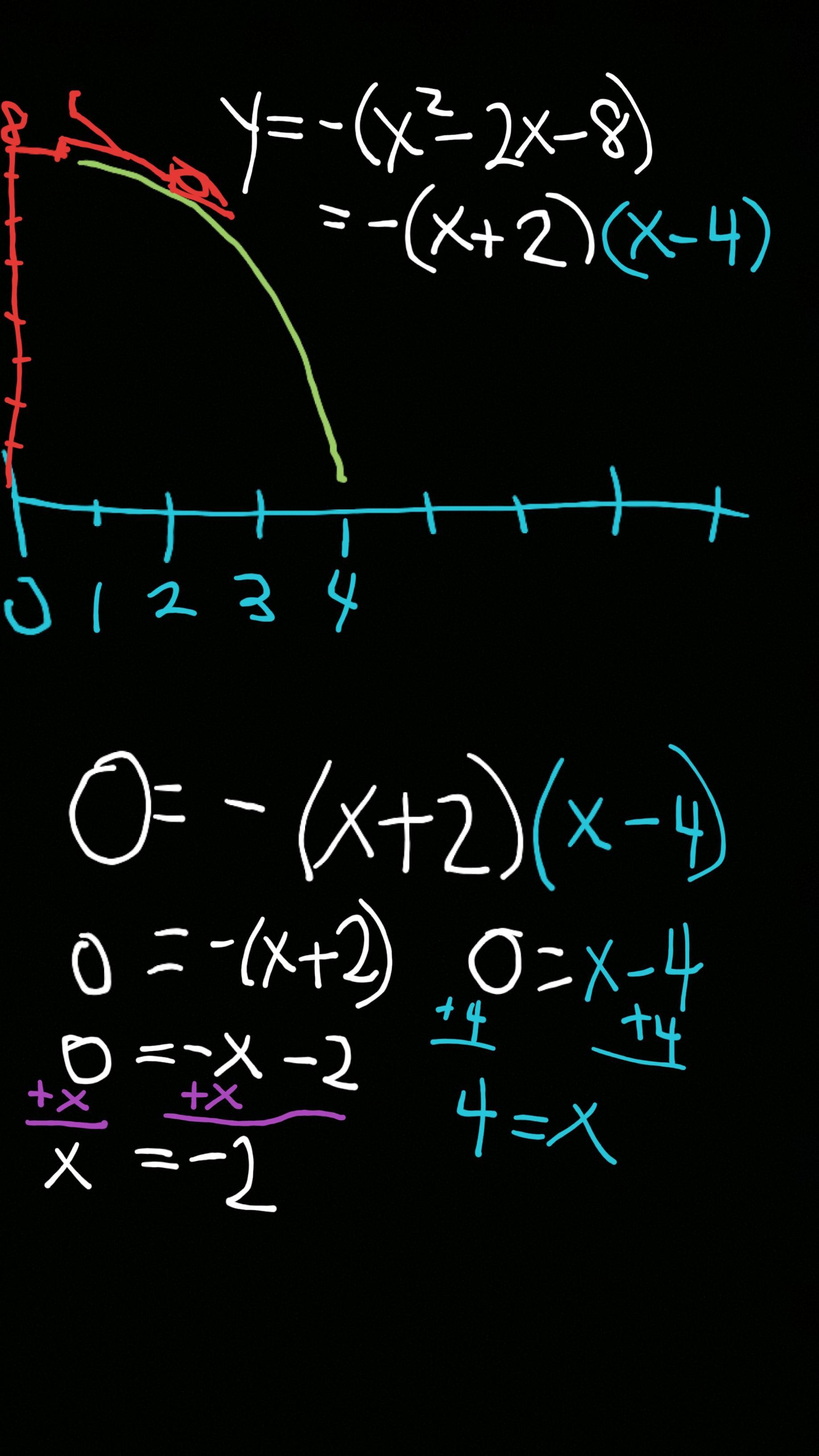
Find zeros

No

-2 doesn't make any sense as distance can't be negative

Answer:

2867 R5

Step-by-step explanation:

Answer:

287

Reminder:5

Decimal

2014÷7

=287.7142857142857

Answer:

Since each question has 3 choices and only one is correct, the probability of answering a single question correctly is 1/3.

To find the probability of answering all 10 questions correctly, we need to multiply the probabilities of each individual question together, since the events are independent.

Therefore, the probability of acing the test is:

(1/3)^10 = 0.000005904

Or approximately 0.0006%, which is a very small probability.

Step-by-step explanation:

By applying the theorem of intersecting secants, the measure of angle XYZ is equal to: A. 35°.

By critically observing the geometric shapes shown in the image attached below, we can deduce that they obey the theorem of intersecting secants.

The theorem of intersecting secants states that when two (2) lines intersect outside a circle, the measure of the angle formed by these lines is equal to one-half (½) of the difference of the two (2) arcs it intercepts.

By applying the theorem of intersecting secants, angle XYZ will be given by this formula:

<XYZ = ½ × (m<WZ - m<XZ)

Substituting the given parameters into the formula, we have;

<XYZ = ½ × (175 - 105)

<XYZ = ½ × 70

<XYZ = 35°.

By applying the theorem of intersecting secants, we can infer and logically deduce that the measure of angle XYZ is equal to 35°.

Read more on intersecting secants here: https://brainly.com/question/1626547

#SPJ1

Answer: 35

Step-by-step explanation:

The minimum distance between the sports car and the truck is 250 miles, and it occurs at exactly noon when they both started moving.

We can use the Pythagorean theorem to find the distance between them at time t:

d² = x² + y²

Where d is the distance between the sports car and the truck, x is the distance traveled by the truck in time t, and y is the distance traveled by the sports car in time t.

x = 60t (distance traveled by truck)

y = 80t (distance traveled by the sports car)

So, the distance between them is:

d² = (60t)² + (80t)²

d² = 3600t² + 6400t²

d² = 10000t²

d = 100t

Now we need to find the time t when the distance d is at its minimum. To do this, we take the derivative of d with respect to t and set it to zero:

d' = 100

Setting d' = 0, we get:

100 = 0

This is not possible, so we conclude that the minimum distance occurs at the time when the derivative of d is zero, which is:

t = 0

The minimum distance is simply the distance between their initial positions:

d = √(250² + 0²) = 250 miles

Therefore, the minimum distance between the sports car and the truck is 250 miles, and it occurs at exactly noon when they both started moving.

More about the speed link is given below.

https://brainly.com/question/7359669

#SPJ1

We will find the speeds of both persons, the correct option is:

"Ramon rides his bike 3 miles per hour slower than Hector rides his bike."

First, we know that Ramon rides 45 miles in 3 hours, then its speed is:

S = 45mi/3h = 15mi/h

And we know that Hector position is given by:

y = 18x

Where x is time in hours, so his velocity is 18mi/h.

Then we can see that:

So the correct statement is:

"Ramon rides his bike 3 miles per hour slower than Hector rides his bike."

If you want to learn more about speed, you can read:

https://brainly.com/question/4931057

Answer:

true

Step-by-step explanation:

Answer:

true

Step-by-step explanation:

To represent the situation, you would have to do 14÷3 because there are 3 cats that eat 14 pounds of food in a week and to figure out how much a cat eats in a week, you would have to divide.

Step-by-step explanation:

Here we are given that , the Value of

And we need to find the prove that ,

We know a identity as ,

On cubing both sides of the given equation,

Simplify and then substitute ,

Hence Proved !

The given function f(x) = 16^x is an exponential function.

An exponential function is a mathematical function in which an independent variable appears in the exponent. In this case, the base of the function is 16, and the variable x is the exponent.

In the given function f(x) = 16^x, the variable x represents the exponent to which 16 is raised. As x increases, the function value grows rapidly, indicating exponential growth. The base of 16 signifies that the function is being multiplied by 16 for each unit increase in the exponent.

In contrast, a linear function has a constant rate of change, and a quadratic function has a squared term. The given function does not involve a linear relationship or a squared term, which confirms that it is not a linear or quadratic function.

Therefore, based on the given form f(x) = 16^x and the exponential growth nature of the function, we can classify it as an exponential function.

Learn more about exponential function here:

brainly.com/question/29287497

#SPJ11

Answer:

ax²+bx+c=0

Being the standard formula

Step-by-step explanation:

By direct substitution

a=1

b=7

c=8

If the quadratic equation x² +7x+8=0 then the correct form after substituting a, b, and c into the Quadratic Formula is x=-7±√7²-4(1)(8)/2(1)

A quadratic equation is a second-order polynomial equation in a single variable x , ax²+bx+c=0. with a ≠ 0 .

The given quadratic equation is x² +7x+8=0.

The quadratic equation standard form is ax²+bx+c=0

When we compare both the equations

The value of a is 1, b is 7 and c is 8

x=-b±√b²-4ac/2a

x=-7±√7²-4(1)(8)/2(1)

=-7±√49-32/2

x=-7±√17/2

Hence, if the quadratic equation x² +7x+8=0 then the correct form after substituting a, b, and c into the Quadratic Formula is x=-7±√7²-4(1)(8)/2(1)

To learn more on Quadratic equation click:

https://brainly.com/question/17177510

#SPJ2

Answer:

x = 6

Step-by-step explanation:

Here it's sayin that Q is a point in between the end-points P & R. If we join P & R , it forms a line segment & Q is in the line segment PR. Hence PQ & QR are also line segments which combine to form line segment PR. So ,

PR = PQ + QR

Putting the given values of PQ ,QR & PR

=> 4x - 12 + 2x + 7 = 31

=> 6x - 5 = 31

=> 6x = 31 + 5

         = 36

=> x = 36/6 = 6

Answer:

its hard to do this without a graph to draw on for you but see explanation

Step-by-step explanation:

from each point count how many units it is from the mirror line and then count that many in the opposite direction. for example, the top left point is 3 away from the mirror line from the left so count 3 units to the right of the mirror line and that is where that point will be.

Answer:  34

================================================

Work Shown:

S = sum of the first 8 numbers

S/8 = 40 is the mean of those first 8 numbers

S = 8*40

S = 320 so the first 8 numbers add to this value

-------

R = sum of the 12 remaining values

R/12 = 30

R = 12*30

R = 360 is what the other 12 values add to

-------

R+S = 360+320 = 680  is what all twenty values add to. Divide this by 20 to get the overall average

(R+S)/20 = 680/20 = 34

Answer:

D

Step-by-step explanation:

hope it will help you that's all I can do

The quadratic equation with zeros at -6 and 2 is y² + 4y - 12 = 0. This is in standard form, which is ax² + bx + c = 0, with a = 1, b = 4, and c = -12.

To find the quadratic equation with zeros at -6 and 2, we can start by using the fact that if a quadratic equation has roots x₁ and x₂, then it can be written in the form

(y - x₁)(y - x₂) = 0

where y is the variable in the quadratic equation.

Substituting the given values of the zeros, we get

(y - (-6))(y - 2) = 0

Simplifying this expression, we get

(y + 6)(y - 2) = 0

Expanding this expression, we get

y² - 2y + 6y - 12 = 0

Simplifying this expression further, we get

y² + 4y - 12 = 0

So the quadratic equation with zeros at -6 and 2 is

y² + 4y - 12 = 0

This is the standard form of a quadratic equation, which is

ax² + bx + c = 0

where a, b, and c are constants. In this case, a = 1, b = 4, and c = -12.

To know more about quadratic equation:

https://brainly.com/question/30098550

#SPJ1

The measures of angles 1 and 2 are both 80° because they are corresponding angles.

Since line c is parallel to line d, we know that ∠1 and ∠2 are corresponding angles, meaning they have the same measure.

Therefore, we can solve for the measure of either angle and know that the other is the same.

From the figure, we can see that the angle formed by lines c and a is a straight angle, which means its measure is 180 degrees.

We also know that ∠1 and the angle adjacent to it (formed by lines a and d) form a straight angle, so the measure of this angle is also 180 degrees.

Subtracting the measure of the known angle from 180 degrees, we can solve for the measure of ∠1:

180 degrees - 100 degrees = 80 degrees.

Therefore, the measures of ∠1 and ∠2 are both 80 degrees.

To learn more about corresponding angles please click on below link        

https://brainly.com/question/1597341

#SPJ4

Answer:

36 x 2 = 72

36 divide by 2 = 18

72+18=90

90 is the answer

Step-by-step explanation:

The property of real number addition which justifies that the two expressions are equivalent is the associative property of addition.

An expression can be defined as a mathematical equation which is used to show the relationship existing between two or more variables and numerical quantities.

Next, we would evaluate both expressions as follows:

When x = 10, we have:

(x + 7) + 3 = (10 + 7) + 3

(10 + 7) + 3 = 17 + 3 = 20.

When x = -4, we have:

(x + 7) + 3 = (-4 + 7) + 3

(-4 + 7) + 3 = 3 + 3 = 6.

When x = 10, we have:

x + 10 = 10 + 10 = 20

When x = -4, we have:

x + 10 = -4 + 10 = 6

The associative property of addition states that when three (3) numbers are added, the end result (output) would always be the same regardless of the way the numbers are arranged (grouped).

In conclusion, the associative property of addition justifies that the two expressions are equivalent.

Read more on associative property of addition here: https://brainly.com/question/7102355

#SPJ1

The values of Z such that e² = 2 + i√3 are Z = ln(2 + i√3) + 2πik, where k is an integer.

To find the values of Z, we can start by expressing 2 + i√3 in polar form. Let's denote it as re^(iθ), where r is the modulus and θ is the argument.

Given: 2 + i√3

To find r, we can use the modulus formula:

r = sqrt(a^2 + b^2)

= sqrt(2^2 + (√3)^2)

= sqrt(4 + 3)

= sqrt(7)

To find θ, we can use the argument formula:

θ = arctan(b/a)

= arctan(√3/2)

= π/3

So, we can express 2 + i√3 as sqrt(7)e^(iπ/3).

Now, we can find the values of Z by taking the natural logarithm (ln) of sqrt(7)e^(iπ/3) and adding 2πik, where k is an integer. This is due to the periodicity of the logarithmic function.

ln(sqrt(7)e^(iπ/3)) = ln(sqrt(7)) + i(π/3) + 2πik

Therefore, the values of Z are:

Z = ln(2 + i√3) + 2πik, where k is an integer.

The values of Z such that e² = 2 + i√3 are Z = ln(2 + i√3) + 2πik, where k is an integer.

To know more about polar form visit

https://brainly.com/question/30824428

#SPJ11

Answer:

4 cm by 24 cm: Yes

6 cm by 22 cm: No

8 cm by 12 cm: Yes

24 cm by 8 cm: No

32 by 6 cm: No

Step-by-step explanation:

Step 1: Find the area of the triangle.

Step 2: Go through each dimensions of the rectangles.

For a sample of rating of an automobile manufacturer's van regarding rating of 28.3 miles/gallon (mpg), the Z-test statistic value is equals to the -1.631.

Z-test is a parametric test. When the sample size is sufficiently large, it is mostly used. This test statistic is computed a standard normal distribution. It is then compared with the critical value (obtained from z-table). If the test statistic value is greater than it, there is evidence to reject the null hypothesis. We have an automobile manufacturer with its van rating. A sample of 200 vans is randomly considered. Sample size, n = 200

Mean of rating = 28

Standard deviations = 2.6

Lavel of significance= 0.01

To test the firm'claim we have to consider hypothesis testing here. Since it is claimed by an automobile manufacturer that its car has a 28.3 miles/gallon (MPG) rating, therefore, the null hypothesis and alternative can be stated as:

\(H_0 :μ = 28.3\)

\(H_a :μ≠28.3\)

Now, to determine the value of the test statistic, consider the Z-test : \( z = \dfrac{{M - \mu }}{{\dfrac{\sigma }{{\sqrt n }}}}\)

where, M -> mean

n --> sample size

Substitute all known values in above formula, \(= \dfrac{{28 - 28.3}}{{\dfrac{{2.6 }}{{\sqrt {200} }}}}\\\)

\(= \dfrac{{-0.3}}{{\dfrac{{0.26 }}{{\sqrt {2} }}}}\\\)

=> z = - 1.631

Hence, required test statistic value is -1.631.

For more information about Z-test, visit :

https://brainly.com/question/30754810

#SPJ4

Answer:

Explanation:

From the graph, the points are best modeled using an exponential function.

Among the given options, Options 3 and 4 are exponential functions.

The third and fourth functions are tested below:

Option 3

Option 4

From the above, we can see that the function that best fits the data shown in the scatterplot is:

Answer:

3(x+4)=5x-9

or,3x+12=5x-9

or,12+9=5x-3x

or,21=2x

orx=21/2

x=10.5

The value of x from the given rectangle is 10.5 units.

The opposite sides of a rectangle are 3(x+4) and 5x-9.

A rectangle is a closed two-dimensional figure with four sides. The opposite sides of a rectangle are equal and parallel to each other and all the angles of a rectangle are equal to 90°.

Now, the opposite sides of a rectangle are congruent

So, 3(x+4) = 5x-9

⇒ 3x+12=5x-9

⇒ 5x-3x=12+9

⇒ 2x=21

⇒ x=10.5 units

Therefore, the value of x from the given rectangle is 10.5 units.

Learn more about a rectangle here:

https://brainly.com/question/10046743.

#SPJ2

1.4 feet

let me know if you get it right hope i helped!

Answer:

14

Step-by-step explanation:

a2+(48)2=

 502\,\,50^{2}502

Use the Pythagorean Theorem.

a2+2304=a^{2}+2304=a2+2304=

 2500\,\,25002500

Simplify.

−2304-2304\phantom{=}−2304=

 −2304\,\,-2304−2304

Subtract 2304 from both sides.

a2=a^{2}=a2=

 196\,\,196196

a2=\sqrt{a^{2}}=a2

​=

 ±196\,\,\pm\sqrt{196}±196

​

Square root both sides.

a=a=a=

 14\,\,1414

Simplify. Ignore negative root, as length must be positive.

A coffee's temperature changed by -54°F in =

\( = 22\frac{1}{2} \: minutes\)

The temperature change in the coffee each minute :-

\( = - 54 \div 22\frac{1}{2} \)

\( = - 54 \div \frac{(2 \times 22 + 1)}{2} \)

\( = - 54 \div \frac{45}{2} \)

\( = - 54 \times \frac{2}{45} \)

\( = \frac{ (- 54 \times 2)}{ \: \: 45} \)

\( = \frac{ - 108}{ \: \: \: 45} \)

\(\color{magenta} = - 2.4°F\)

Therefore , the temperature change in the coffee each minute was = -2.4°F .