if fm= 11, Mg= 3, and PM = 2, what is the measure of PQ?
Answers
We would apply the theorem of the angle formed by two intersecting chords. The given chords are PQ and FG. The theorem states that
angle formed by two intersecting chords = 1/2(sum of intercepted arcs
By applying this theorem, it means that
Related Questions
4. A county clerk has a given amount of money to budget for cultural events. Cultural Events
based on the scattplot, what does the point(0,18)represent?
A.the total amount of the budget givin to the country
B.
the total amount of the budget spent after 18months
C.the average amount spend out of the budget each month
D.the predicted amount of time after which the entire budget will be spent

Answers
Step-by-step explanation:
fjmfplfpefpı6j6jefkıbnkfeknkefekfekffn
5. Let f be a continuous and differentiable function for all real numbers. The only critical points for f are located
at x = -3 and x = 5. If F"(x) = 2x - 2, which of the following must be true?
I. f has a relative maximum at x = -3.
II. f has a relative maximum at x = 5.
III. f has a point of inflection at x = 1.
(A) I only
(B) II only
(C) III only
(D) I and III only
(E) II and III only
Answers
Look at the figure:
An image of a right triangle is shown with an angle labeled x.
If tan x° = a divided by 4 and cos x° = 4 divided by b what is the value of sin x°?
sin x° = 4b
sin x° = b divided by a
sin x° = 4a
sin x° = a divided by b
Answers
By using trigonometric functions, the value of \(\sin \text{x}^\circ\) is \(\frac{\text{a}}{\text{b}}\).
What are trigonometric functions?Trigonometric functions are also known as Circular Functions can be simply defined as the functions of an angle of a triangle. It means that the relationship between the angles and sides of a triangle are given by these trig functions. The basic trigonometric functions are sine, cosine, tangent, cotangent, secant and cosecant.
Given
\(\sin \text{x}^\circ= \ ?\)
\(\tan \text{x}^\circ=\dfrac{\text{a}}{4}\)
\(\cos \text{x}^\circ=\dfrac{4}{\text{b}}\)
Formula to find \(\sin \text{x}^\circ\)
\(\sin \text{x}^\circ=\dfrac{\text{Opposite}}{\text{Hypotenuse}}\)
\(\rightarrow\sin \text{x}^\circ=\bold{\dfrac{a}{b}}\)
Therefore, by using trigonometric functions, the value of \(\sin \text{x}^\circ\) is \(\frac{\text{a}}{\text{b}}\).
To know more about trigonometric functions, visit:
https://brainly.com/question/31540769

Someone answer this plzz Simplify 9^-3
Answers
Answer:
0.00137
Step-by-step explanation:
Answer:
Find the GCD (or HCF) of numerator and denominator. GCD of 9 and 3 is 3.
9 ÷ 33 ÷ 3.
Reduced fraction: 31. Therefore, 9/3 simplified to lowest terms is 3/1
Step-by-step explanation:
Find the GCD (or HCF) of numerator and denominator. GCD of 9 and 3 is 3.
9 ÷ 33 ÷ 3.
Reduced fraction: 31. Therefore, 9/3 simplified to lowest terms is 3//
Solve the equation
: above
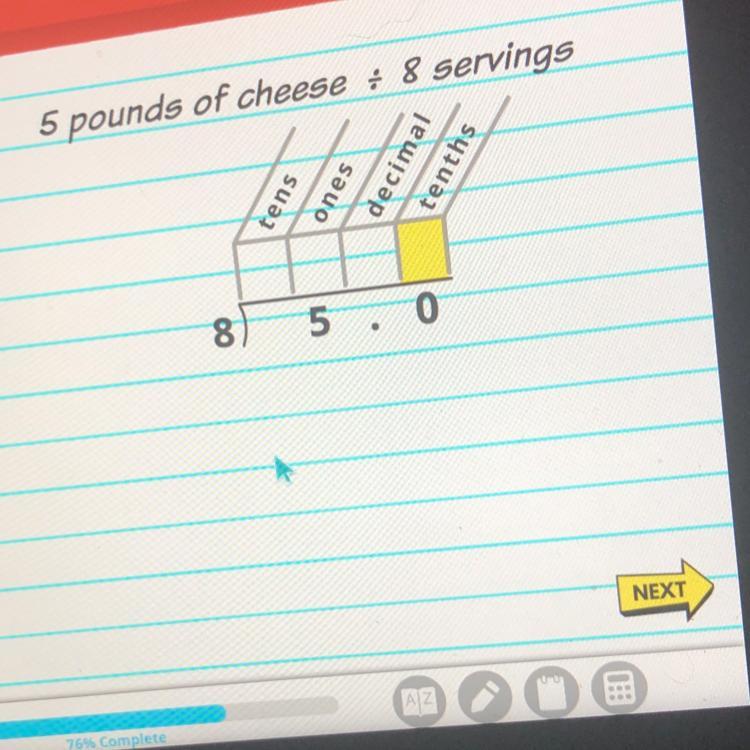
Answers
Answer:
5/8 =0.625
Step-by-step explanation:
Hope this helps
Decide which ONE of the following statements is FALSE.
A) A line contains at least two points
B) Three non-colinear points determine a plane
C) Through any two distinct points there exists exactly one line
D) Any three points lie on a distinct line
Answers
True or False.
A is true, a line has two points which are the two ends.
Answer: Choice D
Any three points lie on a distinct line
=============================================================
Explanation:
Let's go through each statement to see which are true, and which are false.
A) True. A line is uniquely defined by two points. In other words, you need 2 points to form a line.B) True. A plane is a flat surface, such as a table top or a piece of paper. The paper is not allowed to bend or curve. The three points cannot all fall on the same line, hence the "non-colinear" condition put in there. If the 3 points were colinear, then infinitely many planes would go through those points. So we make them non-colinear so a unique single plane can be defined. C) True. See choice A above.D) False. While two points form a single line, that third point may or may not be on that line. If the three points form a single line, then we consider the points colinear. If the points don't all fall on the same line, then we say they are non-colinear. For non-colinear points, a triangle forms. If choice D said "any three colinear points lie on a distinct line", then that statement would be true.Side note: The spelling of "colinear" is sometimes "collinear" with two "L"s, instead of one. I'm not 100% sure which spelling is more acceptable. I've found sources that have used both.
Real-life Problems Question 10

Answers
Answer :
a) It is given that
Everyday a machine makes 500000 staples and puts them into the boxes.
The machine need 170 staples to fill a box
One box contans = 170 staples.
Total number of staples = 500000
Number of box required to fill 500000 staples = Total number of staples/No. of staples 1 box contains.
\( \: :\implies \) 500000 /170
\( :\implies \: \) 2941 (approx)
Therefore, 2941 boxes are required to fill with 500000 staples.
b) It is given that,
Each staple is made of 0.21 g of metal .
Total weight of metal = 1 kg = 1000 g
1 staple = 0.21 g
Number of staples = weight of metal/ Weight of 1 staple.
\( \: :\implies \) 1000/0.21
\( \: :\implies \) 4761 (approximately)
Therefore, 4761 staples can be made from 1 kg of the metal.
30 divided by 1.25 with work
Answers
Answer:
24
Step-by-step explanation:
If you need an explaination you should immediatly remove yourself from the Gene pool, this is a 3rd grade question.
The result is: 30 divided by 1.25 equals 24.
To divide 30 by 1.25, follow these steps in words:
The division 30 ÷ 1.25 can be written as the fraction 30/1.25.
Remove the decimal by multiplying both the numerator and denominator by a power of 10 to convert the decimal into a whole number.
Since 1.25 has two decimal places, multiply both the numerator and denominator by 100 to remove the decimal:
30/1.25 = (30 * 100) / (1.25 * 100) = 3000 / 125
Find the greatest common divisor (GCD) of the numerator and denominator and divide both by the GCD to simplify the fraction:
GCD of 3000 and 125 is 25.
3000 ÷ 25 = 120
125 ÷ 25 = 5
So, 3000/125 simplifies to 120/5.
Divide the numerator by the denominator to get the final result.
Now, simply divide the numerator (120) by the denominator (5):
120 ÷ 5 = 24
The result is 24.
So, 30 divided by 1.25 equals 24.
To learn more on division click:
brainly.com/question/21416852
#SPJ6
Is 312mg greater than 312dg
Answers
312 mg is not greater than 312 dg
A cone has a volume of 2560 Pi cm cubed and a height of 30cm. Find the radius
Answers
•A volume of 2560 pi cm cube
•A height of 30 centimeters
The the required one
•The radius of the cone
Then find the solution
Using the formula
V=πr squared * h/3
Solving for
r=3V 3·2560
π·30≈9.02703
πh=
Which of these polynomial functions is graphed below?

Answers
Answer:
B
Step-by-step explanation:
The \(y\)-intercept is \((0,27)\), eliminating all the options except for B.
Use the Pythagorean Theorem to find the missing length and then round the
result to the nearest tenth.
a = 7, b = 7, C =
Answers
Answer:
C (Hypotenuse) = 9.9
Step-by-step explanation:
Given the two sides of a triangle, where a = 7, and b = 7:
We can use the Pythagorean Theorem where it states: c² = a² + b².
Substitute the given values into the Pythagorean Theorem to find the length of the hypotenuse.
c² = a² + b²
c² = 7² + 7²
c² = 49 + 49
c² = 98
\(\LARGE\mathsf{\sqrt{c^2}\:=\:\sqrt{98}}\)
c = 9.89 or 9.9
Therefore, the length of the hypotenuse is 9.9, making the given triangle an isoceles triangle with two equal sides.
What is the first step in evaluating the expression below 3x{9-(4+2)/2
Answers
The first step in evaluating the expression 3x{9-(4+2)/2 is to simplify the innermost parentheses. By performing operations within parentheses first, we can simplify the expression and make it easier to evaluate.
To begin, we evaluate the expression within the parentheses (4+2), which equals 6. Thus, the original expression becomes 3x{9-6/2}.
The next step is to simplify the division operation. We divide 6 by 2, resulting in 3. Now the expression becomes 3x{9-3}.
The subsequent step is to simplify the subtraction operation. We subtract 3 from 9, giving us 6. The expression now becomes 3x6.
Finally, we multiply 3 by 6, yielding a final result of 18. Therefore, the evaluation of the expression 3x{9-(4+2)/2 is equal to 18.
By simplifying the parentheses, performing the division, and evaluating the subtraction, we systematically reduce the expression to its simplest form. Following the order of operations (also known as PEMDAS or BODMAS), which prioritizes parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right), ensures an accurate evaluation of the expression.
For more questions on BODMAS
https://brainly.com/question/24383445
#SPJ8
Select the correct answer. What is the period of ? A. B. C. D.
Answers
Answer:=
2π/3
Step-by-step explanation:
plato
the fraction 1-4 is blank to the decimal of 0.25
Answers
Answer:
equal
Step-by-step explanation:
1/4 = .25
1÷4=.25
Solve for x: 3x + 2 < 14 and 2 x-5> -11.
Answers
Therefore the value of x is less than 4 when x is in the inequality 3x + 2 < 14 and greater than -3 when x is in the inequality 2 x-5> -11
What is inequality?In mathematics, "inequality" refers to a relationship between two expressions or values that is not equal to each other. Therefore, inequality emerges from a lack of balance. For instance, let's say you have 225 and want to purchase a new bicycle that costs $250.
What is expressions or values?Expressions are carried out, or evaluated, in order to yield a value. In an evaluation process, values of expressions can be employed as components of surrounding expressions.
To solve for x in the inequality 3x + 2 < 14, we can begin by subtracting 2 from both sides to get 3x < 12. Then, dividing both sides by 3 gives x < 4.
To solve for x in the inequality 2 x-5> -11, we can begin by adding 5 to both sides to get 2x> -6, then divide both sides by 2 to get x > -3
So x can be any number greater than -3 and less than 4
To more about Expressions visit:https://brainly.com/question/29284305
#SPJ1
7. You roll a standard dice twice and flip a coin. What is the probability you 5 points
roll a 5, roll a 3 and then flip heads? *
Answers
Answer:
can you just mark me brainlest
Step-by-step explanation:
Answer:
the answer would probably
Step-by-step explanation:
PAY ATTENTION IN CLASS AND YOU WOULD KNOWWW
A box contains 1111 large marbles and 1111 small marbles. Each marble is either green or white. 66 of the large marbles are green, and 44 of the small marbles are white. If a marble is randomly selected from the box, what is the probability that it is small or green? Express your answer as a fraction or a decimal number rounded to four decimal places.
Answers
Answer:
0.7727 = 77.27% probability that it is small or green
Step-by-step explanation:
A probability is the number of desired outcomes divided by the number of total outcomes.
In this question:
11 large + 11 small = 22 total marbles = 22 total outcomes
11 small marbles + 6 green large marbles = 17 desired outcomes.
What is the probability that it is small or green?
\(p = \frac{17}{22} = 0.7727\)
0.7727 = 77.27% probability that it is small or green
Two cars leave the same point at the same time travelling in opposite directions. one car travels west at 20 mph and the other travels east at 60 mph. In how many hours will they be 280 miles apart?
Answers
It will take 3.5 hours for the two cars to be 280 miles apart.
To determine the time it takes for the two cars to be 280 miles apart, we can use the concept of relative velocity.
Since the cars are traveling in opposite directions, their velocities can be added together to find their relative velocity:
Relative velocity = Velocity of car traveling east + Velocity of car traveling west
Relative velocity = 60 mph + 20 mph
Relative velocity = 80 mph
The relative velocity of the cars is 80 mph, which means that they are moving away from each other at a combined speed of 80 mph.
To find the time it takes for them to be 280 miles apart, we can use the formula:
Time = Distance / Speed
Plugging in the values, we have:
Time = 280 miles / 80 mph
Time = 3.5 hours
Therefore, it will take 3.5 hours for the two cars to be 280 miles apart.
During this time, the car traveling east would have covered a distance of 60 mph × 3.5 hours = 210 miles, while the car traveling west would have covered a distance of 20 mph × 3.5 hours = 70 miles.
The sum of these distances is indeed 280 miles, confirming the result.
For more questions on relative velocity.
https://brainly.com/question/11418015
#SPJ8
Can’t figure this out help please

Answers
Answer:
the slope which is given is to plot the points on a graph.
KI's net income for 2021 is _____.
a.
$188.2 million
b.
$118.5 million
c.
$149.3 million
d.
$162.8 million
Answers
Which number sentence is true? 4.05<0.45,4.05=0.45,0.45<0.5
Answers
Answer:
.45<.5
Step-by-step explanation:
the last one is correct
Answer:
0.45 < 0.5
Step-by-step explanation:
4.05<0.454.05 is not less than 0.45
4.05=0.454.05 is not equal to 0.45
0.45 < 0.50.45 is less than 0.5
therefore only the final statement is true.
A spherical balloon is expanding at the rate of 60 pie in^3/sec. How fast is the surface area of the balloon expanding when the radius of the balloon is 4 inches?
Answers
30 is the fast surface area of the balloon expanding when the radius of the balloon is 4 inches
Now, According to the question:
A spherical balloon is expanding at the rate of 60 pie cubic inches /sec.
The radius of the balloon is 4 inches.
Now, Solving the problem:
By using formula:
The formula for the volume of a sphere is V = 4/3 π r³,
where V = volume and r = radius.
Volume = \(\frac{4}{3}\pi r^3\)
We have that:
Differentiate the formula:
\(\frac{dV}{dt}=4\pi r^2.\frac{dr}{dt}= 60\)
=> \(\frac{dr}{dt}=\frac{60}{4\pi r^2}\)
We know that \(A=4\pi r^2,\) and so we also have
\(\frac{dA}{dt}=8\pi r.\frac{dr}{dt}\)
Plug in what we found for dr/dt and plug in r=4 to get:
\(\frac{dA}{dt}=8\pi r.\frac{60}{4\pi r^2}\)
\(\frac{dA}{dt}=\frac{120}{r}=\frac{120}{4}\)
\(\frac{dA}{dt}=30\)
Learn more about Volume of sphere at:
https://brainly.com/question/9994313
#SPJ4
tan-1 5/12 is equal to..
a. sin-1 12/13
b. cos-1 12/13
c.sec-1 12/13
d. cosec-1 12/13
Answers
Answer:
\(\textsf{b)} \quad \cos^{-1}\left(\dfrac{12}{13}\right)\)
Step-by-step explanation:
\(\textsf{Let}\;\;x=\tan^{-1}\left(\dfrac{5}{12}\right)\)
Therefore:
\(\implies \tan x=\tan\left(\tan^{-1}\left(\dfrac{5}{12}\right)\right)\)
\(\implies \tan x=\dfrac{5}{12}\)
The tan trigonometric ratio is the ratio of the side opposite the angle to the side adjacent the angle of a right triangle. Therefore, O = 5 and A = 12. Calculate the hypotenuse (H) by using Pythagoras Theorem.
\(\implies a^2+b^2=c^2\)
\(\implies \sf O^2+A^2=H^2\)
\(\implies 5^2+12^2=\sf H^2\)
\(\implies \textsf{H}=\sqrt{5^2+12^2}\)
\(\implies \textsf{H}=13\)
Therefore, the values are:
θ = xO = 5A = 12H = 13\(\boxed{\begin{minipage}{8cm}\underline{Trigonometric ratios}\\\\$\sf \sin(\theta)=\dfrac{O}{H}\quad\cos(\theta)=\dfrac{A}{H}\quad\tan(\theta)=\dfrac{O}{A}$\\\\\\$\sf\csc(\theta)=\dfrac{H}{O}\quad\sec(\theta)=\dfrac{H}{A}\quad\cot(\theta)=\dfrac{A}{O}$\\\\where:\\\phantom{ww}$\bullet$ $\theta$ is the angle.\\\phantom{ww}$\bullet$ $\sf O$ is the side opposite the angle.\\\phantom{ww}$\bullet$ $\sf A$ is the side adjacent the angle.\\\phantom{ww}$\bullet$ $\sf H$ is the hypotenuse.\\\end{minipage}}\)
Substitute the values into the sin, cos, sec and cosec ratios then take their inverses.
\(\sin x = \dfrac{5}{13}\implies \sin^{-1}(\sin x) = \sin^{-1}\left(\dfrac{5}{13}\right)\implies \boxed{x= \sin^{-1}\left(\dfrac{5}{13}\right)}\)
\(\cos x = \dfrac{12}{13}\implies \cos^{-1}(\cos x) = \cos^{-1}\left(\dfrac{12}{13}\right)\implies \boxed{x= \cos^{-1}\left(\dfrac{12}{13}\right)}\)
\(\sec x = \dfrac{13}{12}\implies \sec^{-1}(\sec x) = \sec^{-1}\left(\dfrac{13}{12}\right)\implies \boxed{x= \sec^{-1}\left(\dfrac{13}{12}\right)}\)
\(\csc x = \dfrac{13}{5}\implies \csc^{-1}(\csc x) = \csc^{-1}\left(\dfrac{13}{5}\right)\implies \boxed{x= \csc^{-1}\left(\dfrac{13}{5}\right)}\)
Therefore, from the given answer options:
\(x=\tan^{-1}\left(\dfrac{5}{12}\right)=\cos^{-1}\left(\dfrac{12}{13}\right)\)
Rearrange the fractions in ascending order:
1/3, 3/11, 6/19, 5/8
Answers
Answer: 3/11, 6/19/, 1/3, 5,8
Hope this helps
Step-by-step explanation:
At an average rate of 38 miles an hour, how far can you travel in 4 hours?
a.
156 mi
b.
195 mi
c.
190 mi
d.
152 mi
Answers
Translate 2 3 y − 9 < y + 1 into a sentence. Nine than two-thirds of number is less than the number .
Answers
The sentence translation of "2/3y - 9 < y + 1" is "Nine less than two-thirds of a number is less than the number."
To translate the inequality expression "2/3y - 9 < y + 1" into a sentence, we can break it down into smaller parts:
"2/3y" represents two-thirds of a number.
"9" represents the number nine.
"y + 1" represents the number increased by one.
Now let's construct the sentence:
"Nine less than two-thirds of a number" - This refers to the expression "2/3y - 9," indicating that we have subtracted nine from two-thirds of a number.
"is less than" - This is the comparison symbol in the inequality.
"the number" - This refers to the expression "y + 1," representing the number increased by one.
Combining these parts, we form the sentence: "Nine less than two-thirds of a number is less than the number."
Hence, the correct sentence translation of "2/3y - 9 < y + 1" is "Nine less than two-thirds of a number is less than the number."
For more such questions on sentence translation, click on:
https://brainly.com/question/28958546
#SPJ8
Evaluate the surface integral.
S(x + y + z) dS, S is the parallelogram with parametric equations
x = u + v, y = u − v, z = 1 + 2u + v, 0 ≤ u ≤ 7, 0 ≤ v ≤ 3.
Answers
Answer:
\(\frac{115\sqrt{14} }{2}\)
Step-by-step explanation:
∫∫ ( x + y + z ) ds
where :
x = u + v , y = u - v , z = 1 + 2u + v ,
0 ≤ u ≤ 5,
0 ≤ v ≤ 1
R ( u,v ) = < u+v , u-v , 1 + 2u +v > next we have to differentiate
attached below is a detailed solution

The surface integral S(x + y + z) dS, where S is the parallelogram is \(115\sqrt{14} /2\) and this can be determined by integrating the given expression.
Given :
The surface integra S(x + y + z) dS, S is the parallelogram with parametric equations x = u + v, y = u − v, z = 1 + 2u + v, 0 ≤ u ≤ 7, 0 ≤ v ≤ 3.
The following calculation can be used in order to determine the surface integral.
\(\rm R_u\times R_v = <3,1,-2>\)
\(\rm ||R_u\times R_v|| = \sqrt{14}\)
Now, evaluate the surface integral.
\(\rm I = \rm \int\int_s(x+y+z)dr\)
\(\rm I = \int^5_{u=0}\int^1_{v=0}(u+v+u-v+1+2u+v)||R_u\times R_v||dA\)
\(\rm I = \int^5_{u=0}\int^1_{v=0}(u+v+u-v+1+2u+v)\sqrt{14} \;du\;dv\)
\(\rm I = \sqrt{14} \int^5_0(v+4uv+\dfrac{v^2}{2})^1_0\;du\)
\(\rm I = \sqrt{14} (\frac{3}{2}u+4\frac{u^2}{2})^5_0\)
Simplify the above expression.
\(\rm I = \dfrac{115\sqrt{14} }{2}\)
The surface integral S(x + y + z) dS, where S is the parallelogram is \(115\sqrt{14} /2\)
For more information, refer to the link given below:
https://brainly.com/question/22008756
9x^2 - y^2 - 90x + 4y + 140= 01. does the ellipse open horizontally or vertically?2. give the coordinates of the right vertex3. give the coordinates of the left vertex4. give the coordinates of the right focus(round to the nearest hundredths)5. give the coordinates of the left focus(round to the nearest hundredths)6. give the eccentricity
Answers
the function is:
\(9x^2-y^2-90x+4y+140=0\)We can graph the function to be sure:
1) In this case the ellipse open vertically because there is a contraction in the x axis.
2) The right vertex is in the coordinate (8,2)
3) the eft vertex is in the coordinate (2,2)
4) the right focus is in the coordinate (14.5,2)
5) the left focus is in the coordinate (-4.5,2)
6) and the eccentricity is the divition between the distance of the center to the vertex by the distance of the center to the focus so:
\(e=\frac{9.5}{3}=3.16\)
Richest people. Find the net
worth of the worlds three
Richest people. Put these
monetary values in Perspective
through any techniques you wish
Answers
Answer:
Elon Musk 269.7 B
Jeff Bezos: 170.15 B
Bill Gates: 130.23 B
Step-by-step explanation:
If you had a trail of 1 billion 1 dollar bills, it would stretch out to 96,900 miles.
If you did that with 100 billion 1 dollar bills, that would be 9,690,000 miles.
That is more than 300 times the circumference of the Earth.